Wolfram Demonstrations Project

12,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

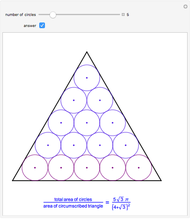
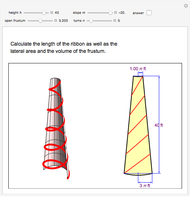
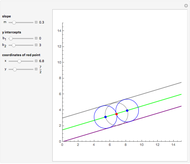
Tangent Circles to Two Parallel Lines and Passing through a Point

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

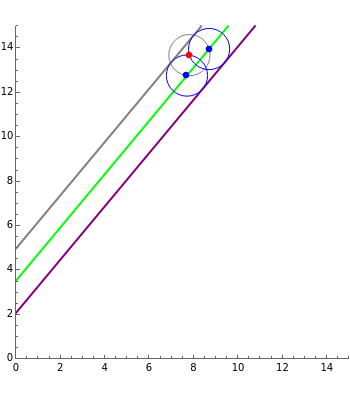
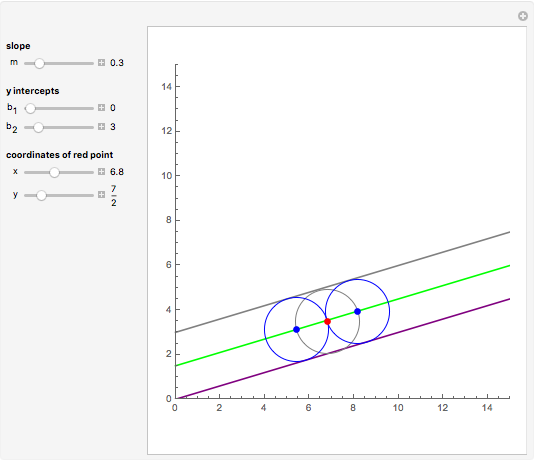
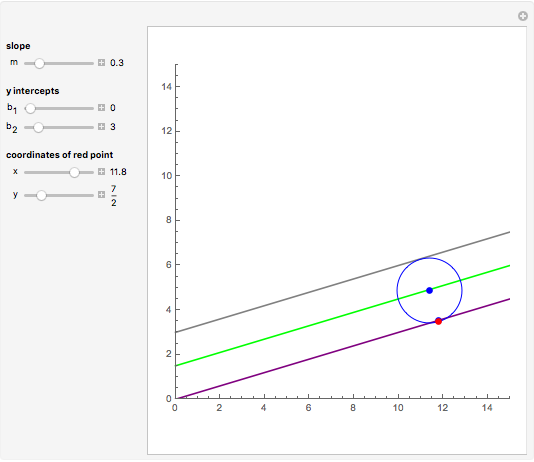
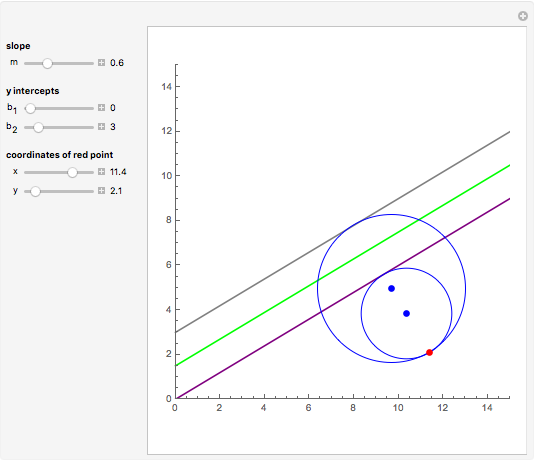
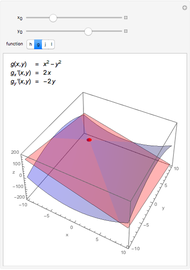
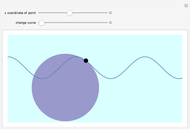
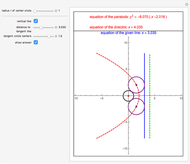
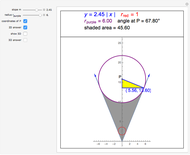
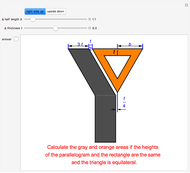
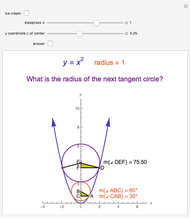
This Demonstration is based on an example from a Wolfram Education Group seminar: Given two parallel lines and a point between them, draw circles of radius centered at and through and tangent to the lines. Then the circle centered at with radius passes through and .

[more]

Here we allow the two blue circles to be constructed when the point is not between the two lines.

[less]

Contributed by: Abraham Gadalla (July 2011)
Open content licensed under CC BY-NC-SA

Snapshots

Details

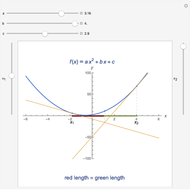
The distance from a point in the - plane to the line with equation , where is the slope of the line and is the intercept, can be proven to be .

See the Wolfram Education Group seminar, Calculus: Fundamentals.

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send