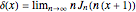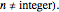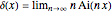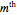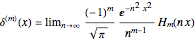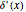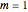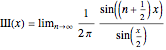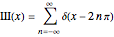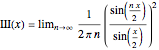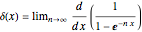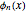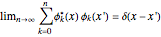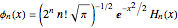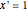The Deltafunction as the Limit of Some Special Functions
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
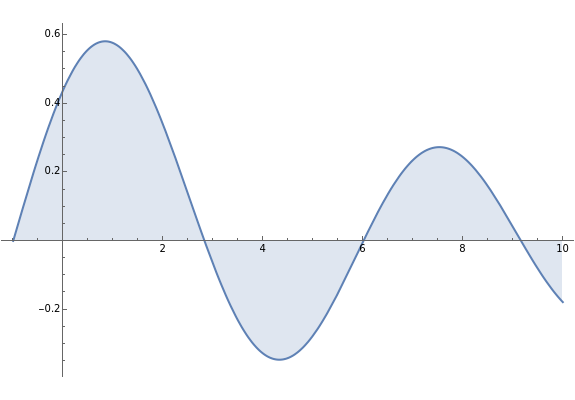
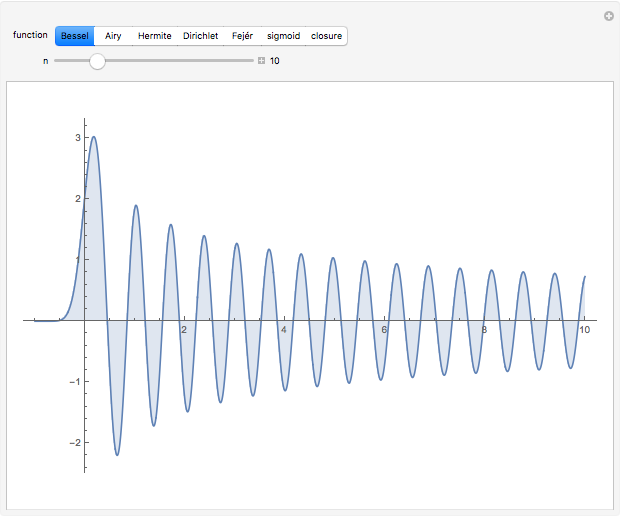
Another Demonstration gives some representations for the Dirac deltafunction as the limit of elementary functions (see the related links below).This Demonstration illustrates several additional limiting relations involving special functions and the deltafunction:
[more]
Contributed by: S. M. Blinder (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
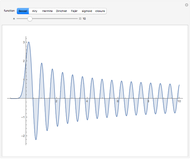
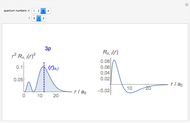
Snapshot 1: Bessel function representation with 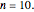 All but the leftmost peak will produce canceling contributions as
All but the leftmost peak will produce canceling contributions as 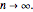
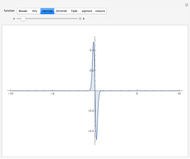
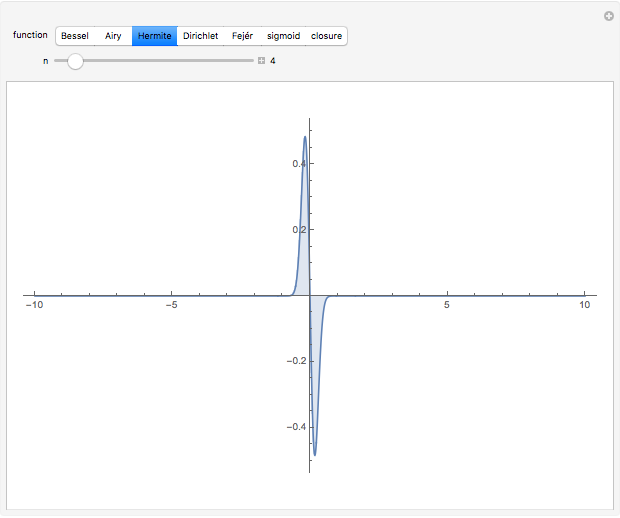
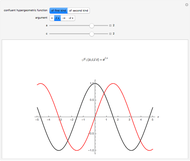
Snapshot 2: approach to 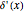 using Hermite polynomial
using Hermite polynomial 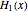
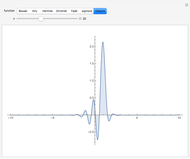
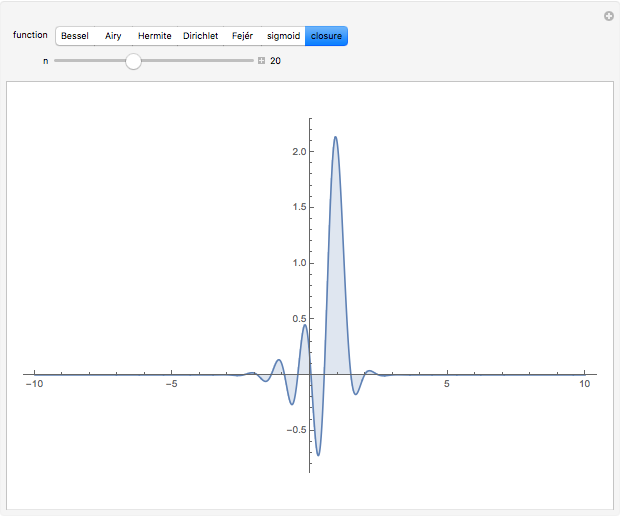
Snapshot 3: closure relation approaching 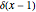
Permanent Citation