Through Any Three Points

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
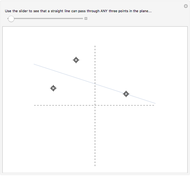
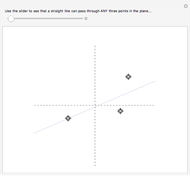
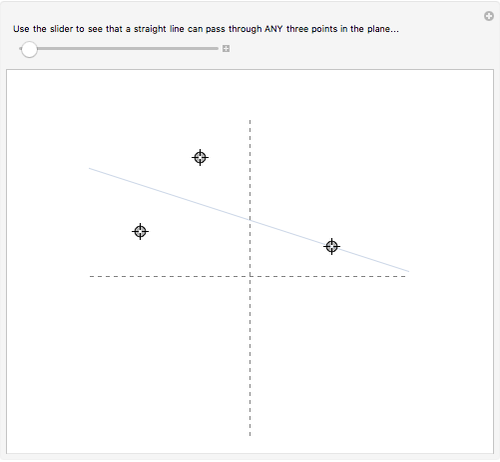
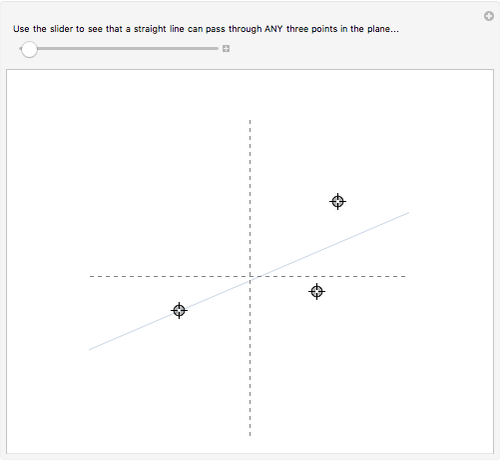
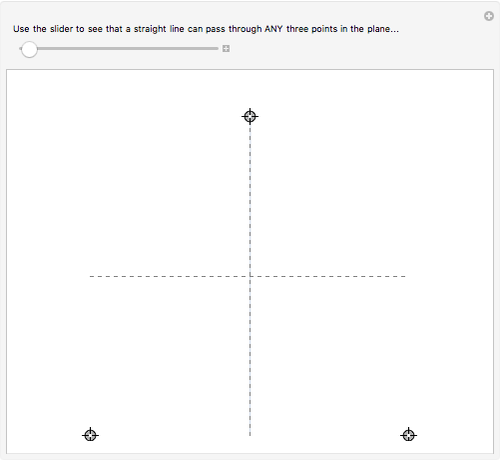
Drag the three locators anywhere in the plot area. Then move the slider button to the right, to show that a straight line can pass through ANY three points in a plane…
Contributed by: Mark D. Normand (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
The Snapshots show some possible positions for the three locators and the automatically oriented line.
An idealized mathematical line has zero thickness. As in other real-world depictions of a line, a plotted line in Mathematica must have sufficient positive thickness in order to be visible. (In mathematics the region between two parallel lines is called a strip.) It is easier to make a strip with some positive thickness pass through several points than if the corresponding line has zero thickness. This Demonstration shows that if the thickness can be increased indefinitely, then the strip can be made to pass through any three points in a plane. Of course, this is not what is normally understood by the phrase "to pass a line through any three points in a plane"!
When the three locators identify the vertices of a triangle, the line is automatically oriented so as to join the midpoint of the triangle's shortest side with the vertex opposite that side. Then, as the slider is used to increase the thickness, the three points identified by locators will eventually be covered by a strip of minimum thickness, at which point a message appears above the plot area. The message can also be made to appear without increasing the thickness by moving the locators so that two or all three lie directly on top of one another or by positioning them so that all three lie at different locations along a zero-thickness line (for example, along one of the dashed axis lines).
A strip of maximum thickness, i.e., one so thick it fills the entire plot area before the message appears, can be forced by dragging two of the locators to adjacent corners of the plot area and the third to the midpoint of the opposite side, as shown in Snapshot 3. The dashed axis lines can be helpful in the precise placement of the three locators.
The source of the quip on which this Demonstration is based is unknown to the author.
Permanent Citation