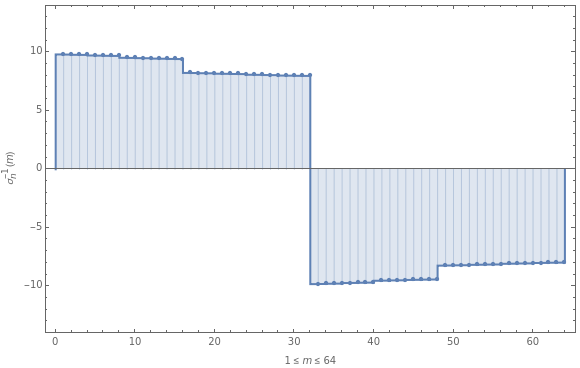
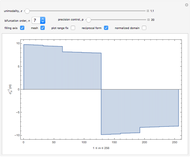
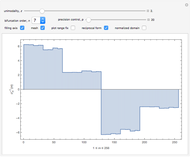
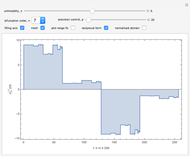
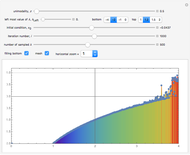
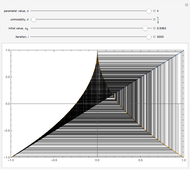
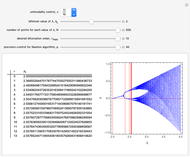
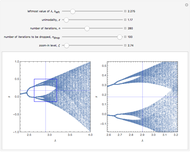
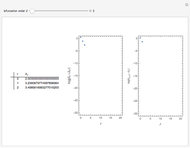
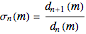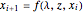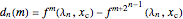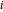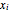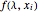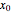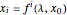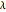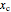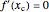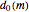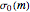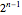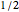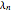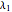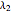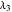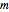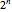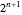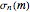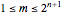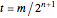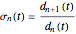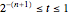Trajectory-Scaling Functions for Generalized Logistic Maps with z-Unimodality

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
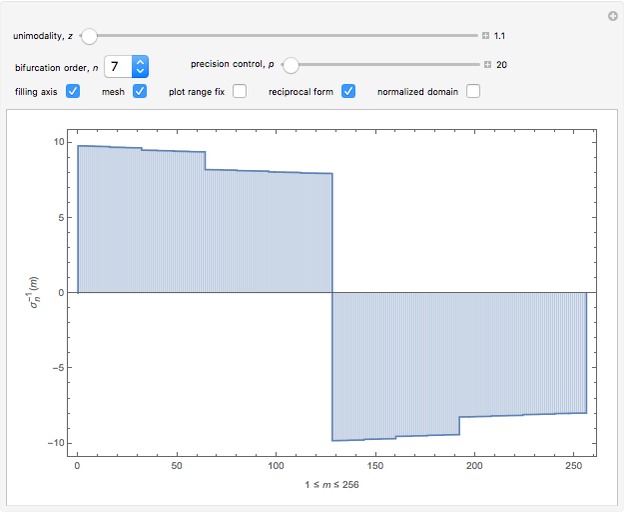
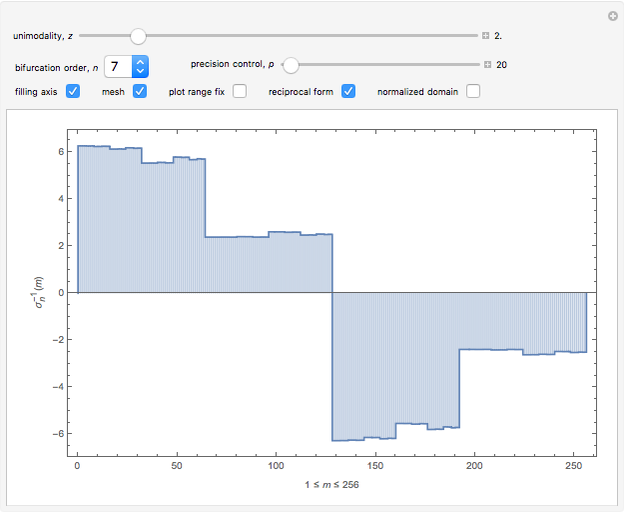
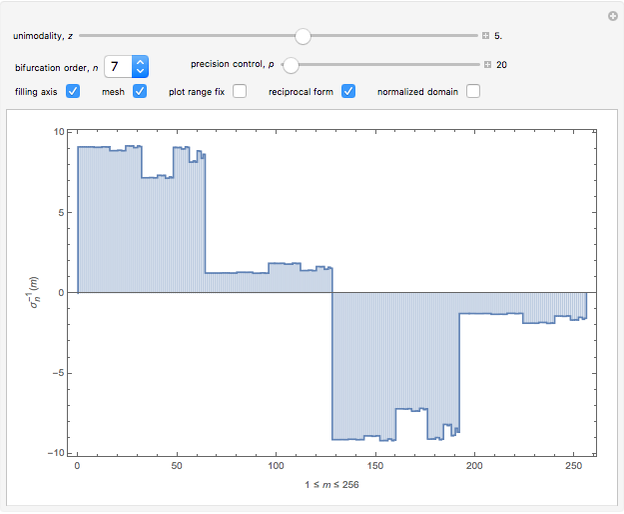
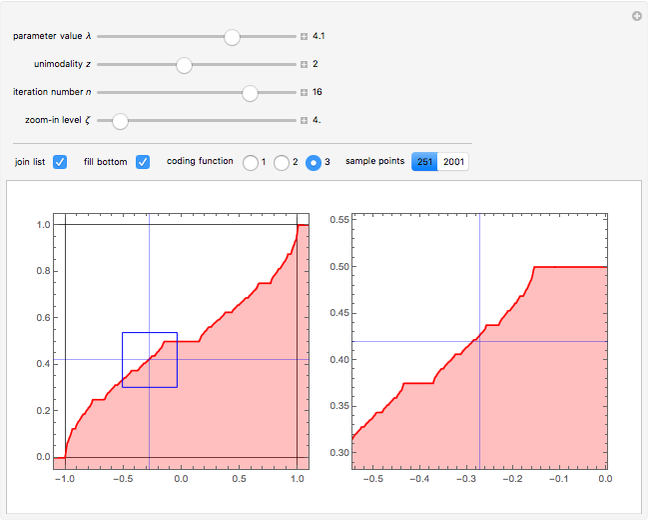
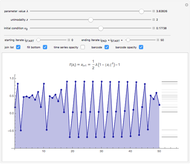
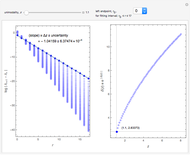
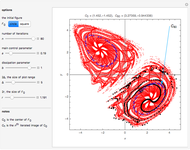
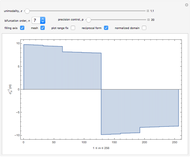
This Demonstration shows Feigenbaum's trajectory scaling function (TSF) [1–6],
[more]
Contributed by: Ki-Jung Moon (January 2014)
Based on a program by: Stephen Wolfram
Open content licensed under CC BY-NC-SA
Snapshots
Details
1. The test map used in this Demonstration, 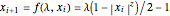 , generalizes the well-known logistic map
, generalizes the well-known logistic map 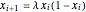 [7–14]. Here
[7–14]. Here 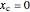 ;
;  is the subcontrol parameter (which determines the unimodality, the degree of the local maximum of
is the subcontrol parameter (which determines the unimodality, the degree of the local maximum of 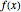 ).
).
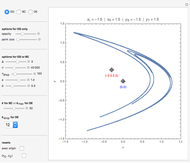
2. The program for this Demonstration presents a large collection of super-stable parameter values 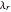 for period-doubling periodic attractors within
for period-doubling periodic attractors within 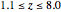 and
and 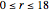 , which were obtained using a high-precision Newton algorithm with fixed precision 50. These values are accurate up to 45 decimal places, so you can use them for your own research or study.
, which were obtained using a high-precision Newton algorithm with fixed precision 50. These values are accurate up to 45 decimal places, so you can use them for your own research or study.
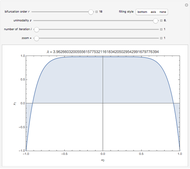
3. For 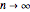 , the lower bound of the domain of
, the lower bound of the domain of 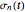 approaches
approaches  (i.e.
(i.e. 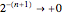 ) and
) and 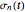 approaches the universal scaling function
approaches the universal scaling function 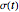 (i.e.
(i.e. 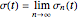 ) satisfying the following properties:
) satisfying the following properties:
universality:  ,
,  ,
, 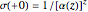 ,
,  ,
,
symmetry: 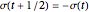 for
for 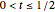 ,
, 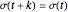 for
for 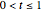 and
and 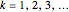 ,
,
where 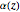 is the second Feigenbaum constant as a function of
is the second Feigenbaum constant as a function of  [1–6,15].
[1–6,15].
4. For the practical purpose of numerical simulation, 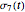 is good enough to observe the universality [1, 3]. In 1988, Andrew L. Belmonte et al. used
is good enough to observe the universality [1, 3]. In 1988, Andrew L. Belmonte et al. used 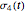 (i.e.
(i.e. 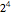 cycles obtained from the logistic map) for comparison with their experimental data [4].
cycles obtained from the logistic map) for comparison with their experimental data [4].
References
[1] M. J. Feigenbaum, "The Transition to Aperiodic Behavior in Turbulent Systems," Communications in Mathematical Physics, 77, 1980 pp. 65–86.
[2] M. J. Feigenbaum, "The Metric Universal Properties of Period Doubling Bifurcations and the Spectrum for a Route to Turbulence," Annals of the New York Academy of Sciences, 357, 1980 pp. 330–336.
[3] M. J. Feigenbaum, "Universal Behavior in Nonlinear Systems," Los Alamos Sciences, 1, 1980 pp. 4–27.
[4] A. L. Belmonte, M. J. Vinson, J. A. Glazier, G. H. Gunaratne, and B. G. Kenny, "Trajectory Scaling Functions at the Onset of Chaos: Experimental Results," Physical Review Letters, 61(5), 1988 pp. 539–542.
[5] M. C. de S. Vieira and G. H. Gunaratne, "The Trajectory Scaling Function for Period Doubling Bifurcations in Flows," Journal of Statistical Physics, 58, 1990 pp. 1245–1256.
[6] E. Mayoral and A. Robledo, "Tsallis'  Index and Mori's
Index and Mori's  Phase Transitions at the Edge of Chaos," Physical Review E, 72(2), 2005 p. 026209.
Phase Transitions at the Edge of Chaos," Physical Review E, 72(2), 2005 p. 026209.
[7] S. H. Strogatz, Nonlinear Dynamics and Chaos, New York: Perseus Books Publishing, 1994.
[8] K. T. Alligood, T. D. Sauer, and J. A. Yorke, Chaos: An Introduction to Dynamical Systems, New York: Springer, 1996.
[9] H.-O. Peitgen, H. Jürgens, and D. Saupe, Chaos and Fractals: New Frontiers of Science, 2nd ed., New York: Springer, 2004.
[10] S. Wolfram, A New Kind of Science, Champaign, IL: Wolfram Media, 2002.
[11] M. J. Feigenbaum, "Quantitative Universality for a Class of Non-Linear Transformations," Journal of Statistical Physics, 19, 1978 pp. 25–52.
[12] M. J. Feigenbaum, "The Universal Metric Properties of Nonlinear Transformations," Journal of Statistical Physics, 21, 1979 pp. 669–706.
[13] K.-J. Moon, "Reducible Expansions and Related Sharp Crossovers in Feigenbaum's Renormalization Field," Chaos: An Interdisciplinary Journal of Nonlinear Science, 18, 2008 p. 023104.
[14] K.-J. Moon, "Erratum: Reducible Expansions and Related Sharp Crossovers in Feigenbaum's Renormalization Field," Chaos: An Interdisciplinary Journal of Nonlinear Science, 20, 2010 p. 049902.
[15] K. Briggs, "Feigenbaum Scaling in Discrete Dynamical Systems," Ph.D. thesis, Department of Mathematics, Melbourne University, Australia, 1997. keithbriggs.info/documents/Keith_Briggs_PhD.pdf.
Permanent Citation