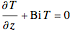Transient Cooling of Composite Solids
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
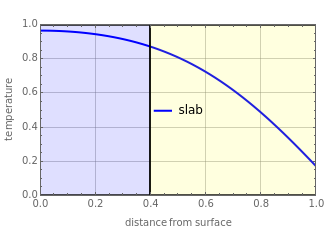
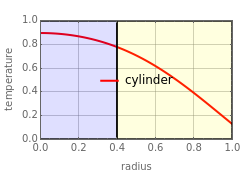
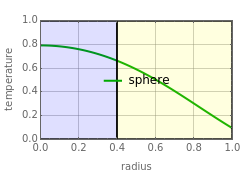
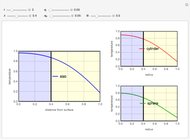
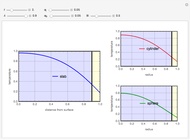
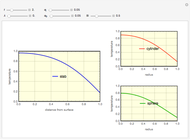
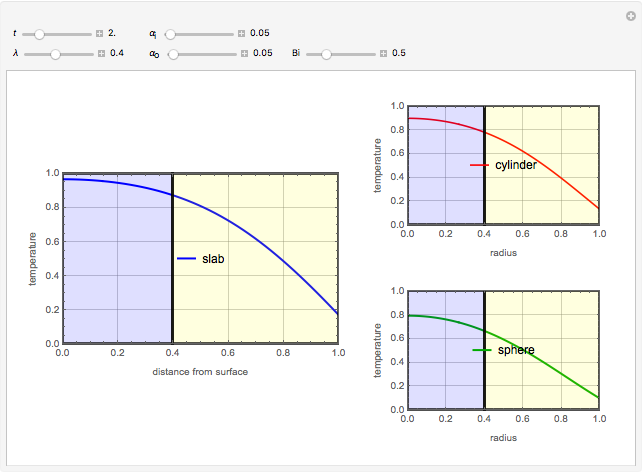
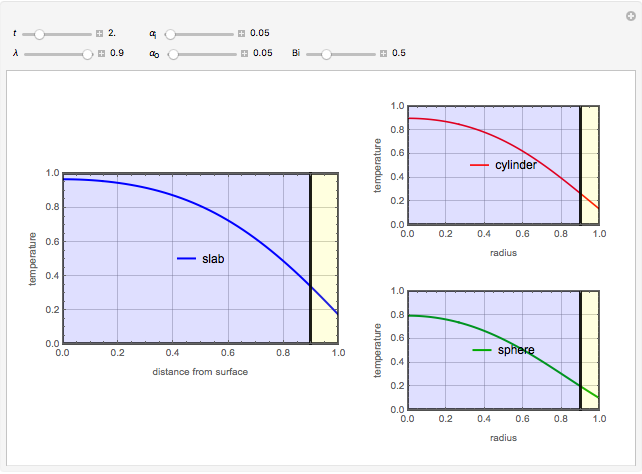
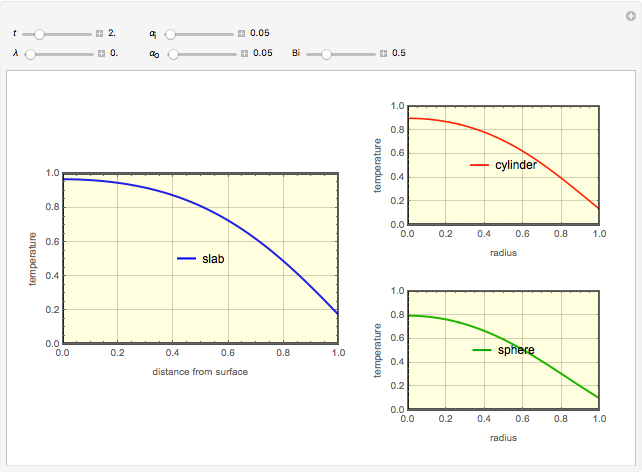
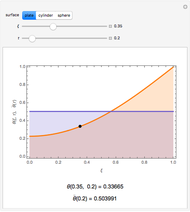
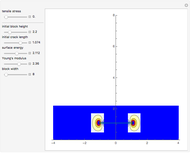
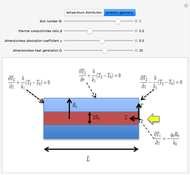
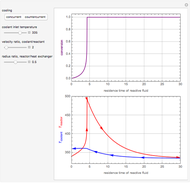
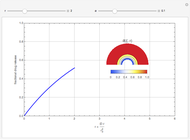
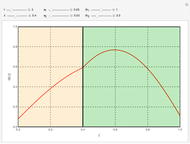
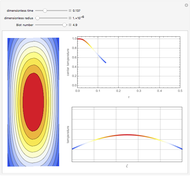
This Demonstration illustrates the transient cooling of a composite solid: a slab, cylinder or sphere that is suddenly immersed in a cooling bath. The slab consists of two parallel layers, while the cylinder and sphere consist of two concentric layers. The individual layers have different thermal properties and are in perfect thermal contact with one another.
[more]
Contributed by: Clay Gruesbeck (March 2017)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Permanent Citation