Two-Soliton Collision for the Gross-Pitaevskii Equation in the Causal Interpretation

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
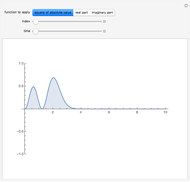
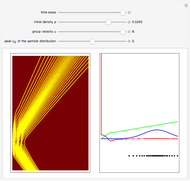
Under certain simplified assumptions (small amplitudes, propagation in one direction, etc.), various dynamical equations can be solved, for example, the well-known nonlinear Schrödinger equation (NLS), also known as the Gross–Pitaevskii equation. It has a soliton solution, whose envelope does not change in form over time. Soliton waves have been observed in optical fibers, optical solitons being caused by a cancellation of nonlinear and dispersive effects in the medium. When solitons interact with one another, their shapes do not change, but their phases shift. The two-soliton collision shows that the interaction peak is always greater than the sum of the individual soliton amplitudes. The causal interpretation of quantum theory is a nonrelativistic theory picturing point particles moving along trajectories, here, governed by the nonlinear Schrödinger equation. It provides a deterministic description of quantum motion by assuming that besides the classical forces, an additional quantum potential acts on the particle and leads to a time-dependent quantum force 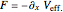 . When the quantum potential
. When the quantum potential 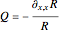 in the effective potential
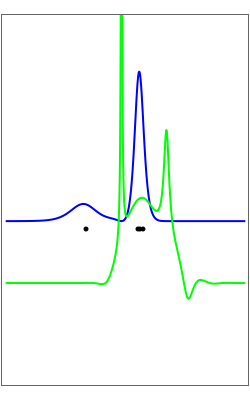
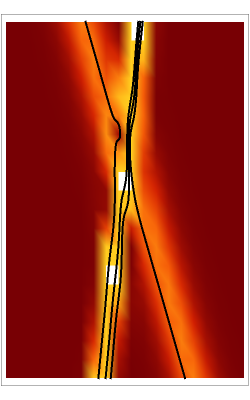
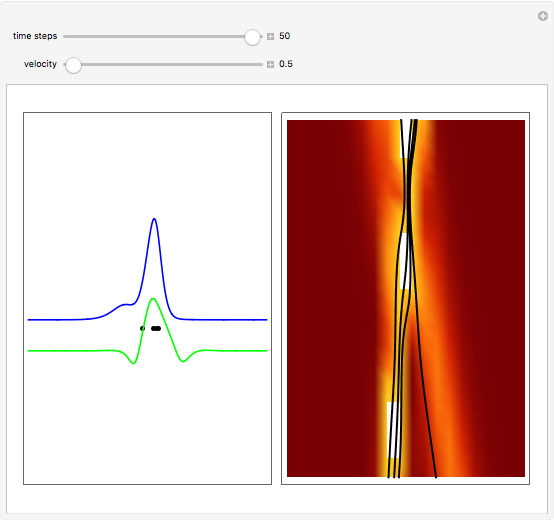
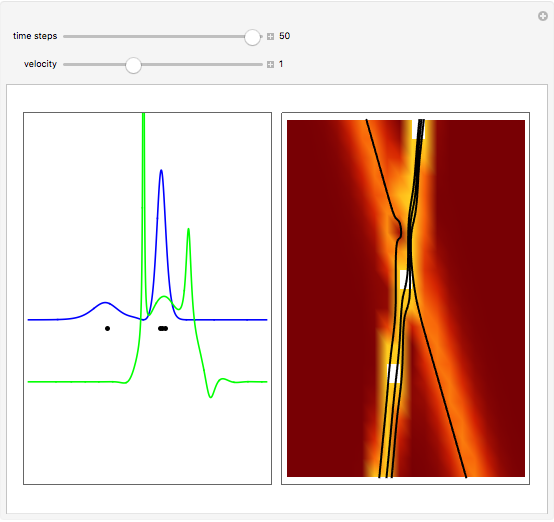
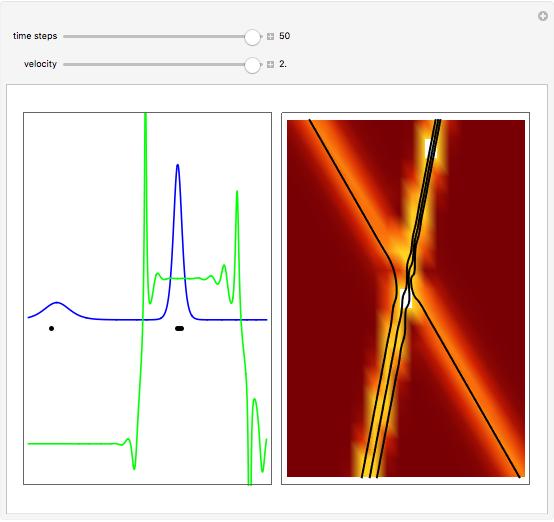
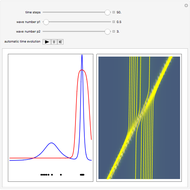
in the effective potential 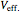 is negligible, the equation for the force will reduce to the standard Newtonian equations of classical mechanics. In the two-soliton case, only two of the Bohmian trajectories correspond to reality; all the others represent possible alternative paths depending on the initial configuration. The trajectories of the individual solitons show that in the two-soliton collision, amplitude and velocity are exchanged, rather than passing through one another. On the left you can see the position of the particles, the wave amplitude (blue), and the velocity (green). On the right the graphic shows the wave amplitude and the complete trajectories in (
is negligible, the equation for the force will reduce to the standard Newtonian equations of classical mechanics. In the two-soliton case, only two of the Bohmian trajectories correspond to reality; all the others represent possible alternative paths depending on the initial configuration. The trajectories of the individual solitons show that in the two-soliton collision, amplitude and velocity are exchanged, rather than passing through one another. On the left you can see the position of the particles, the wave amplitude (blue), and the velocity (green). On the right the graphic shows the wave amplitude and the complete trajectories in ( ,
,  ) space.
) space.
Contributed by: Klaus von Bloh (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
With the potential  , the Gross–Pitaevskii equation
, the Gross–Pitaevskii equation 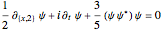 is the nonlinear version of the Schrödinger equation
is the nonlinear version of the Schrödinger equation 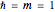 , where
, where  is the complex conjugate and
is the complex conjugate and  the density of the wavefunction.
the density of the wavefunction.
The exact two-soliton solution is:
 , with
, with
 ,
,
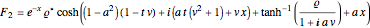 ,
,
and
 and
and  , and here
, and here 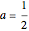 .
.
There are two ways to derive the velocity equation: (1) directly from the continuity equation, where the motion of the particle is governed by the current flow; and (2) from the eikonal representation of the wave,  , where the gradient of the phase
, where the gradient of the phase 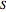 is the particle velocity. Therefore, the quantum wave guides the particles. The origin of the motion of the quantum particle is the effective potential
is the particle velocity. Therefore, the quantum wave guides the particles. The origin of the motion of the quantum particle is the effective potential 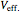 , which is the quantum potential
, which is the quantum potential  plus the potential
plus the potential  ,
, 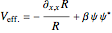 . The effective potential
. The effective potential 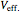 is a generalization of the quantum potential in the case of the Schrödinger equation for a free quantum particle, where
is a generalization of the quantum potential in the case of the Schrödinger equation for a free quantum particle, where  . The system is time reversible. In the source code the quantum potential is deactivated, because of the excessive computation time.
. The system is time reversible. In the source code the quantum potential is deactivated, because of the excessive computation time.
References:
J. P. Gordon, "Interaction Forces among Solitons in Optical Fibers", Optics Letters, 8(11), 1983 pp. 596–598.
P. Holland, The Quantum Theory of Motion, Cambridge: Cambridge University Press, 1993.
Permanent Citation