Vapor Pressures of Binary Solutions

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
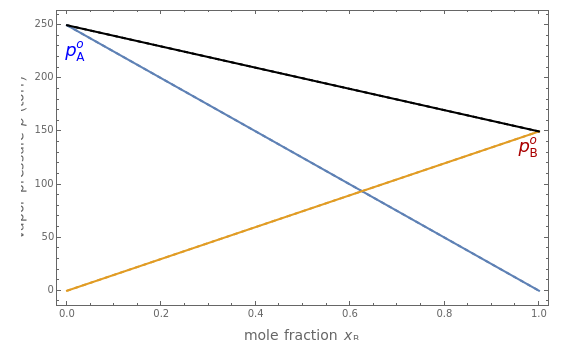
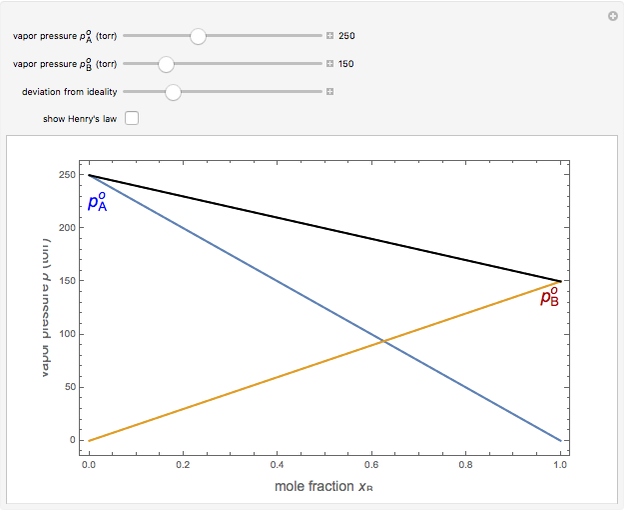
An ideal solution of two liquids  and
and  obeys Raoult's law, which states that the partial vapor pressure of each component is proportional to its mole fraction:
obeys Raoult's law, which states that the partial vapor pressure of each component is proportional to its mole fraction:  and
and  , where
, where  and
and  are the vapor pressures of the pure components at a given temperature (very often 25 °C). The total vapor pressure above the solution is then given by
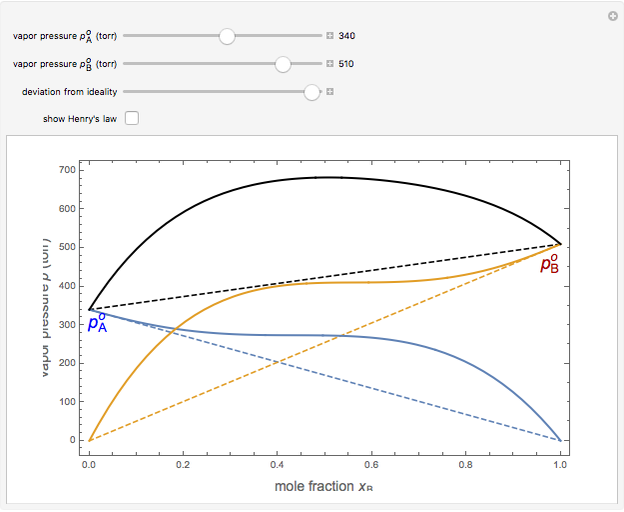
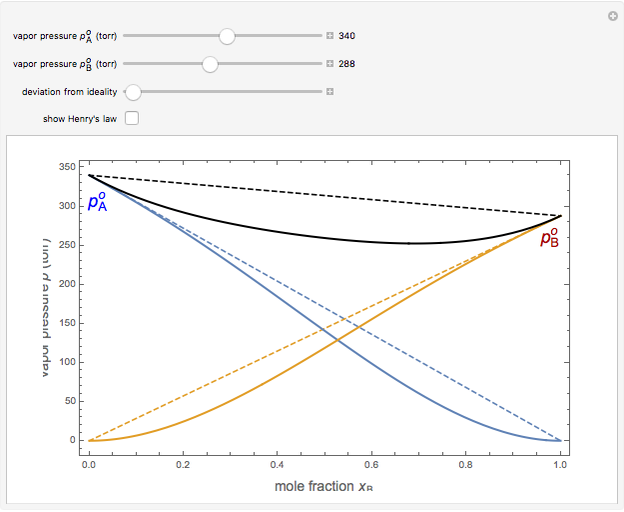
are the vapor pressures of the pure components at a given temperature (very often 25 °C). The total vapor pressure above the solution is then given by  , assuming Dalton's law. Ideal solutions are fairly uncommon but serve as a convenient reference system to describe nonideal solutions. Pairs of liquids that are well approximated by Raoult's law usually contain molecules of similar size, shape, and chemical structure. Some well-known examples are benzene and toluene, chlorobenzene and bromobenzene, and carbon tetrachloride and silicon tetrachloride.
, assuming Dalton's law. Ideal solutions are fairly uncommon but serve as a convenient reference system to describe nonideal solutions. Pairs of liquids that are well approximated by Raoult's law usually contain molecules of similar size, shape, and chemical structure. Some well-known examples are benzene and toluene, chlorobenzene and bromobenzene, and carbon tetrachloride and silicon tetrachloride.
Contributed by: S. M. Blinder (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
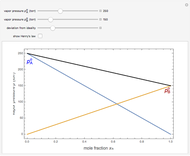
Snapshot 1: an ideal solution
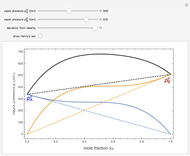
Snapshot 2: acetone-carbon disulfide solution, showing strong positive deviation from ideality
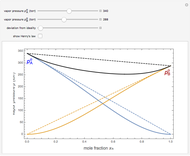
Snapshot 3: acetone-chloroform solution, showing negative deviation from ideality
Reference: P. Atkins and J. de Paula, Physical Chemistry 7th ed., New York: W. H. Freeman and Co., 2002, pp. 168–172.
Permanent Citation