Visualizing Correlations

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
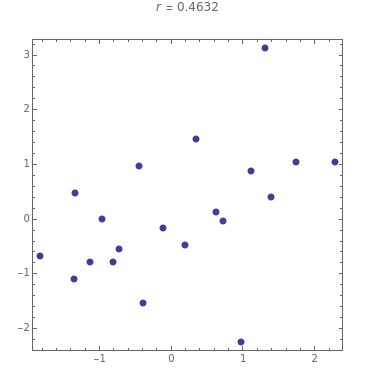
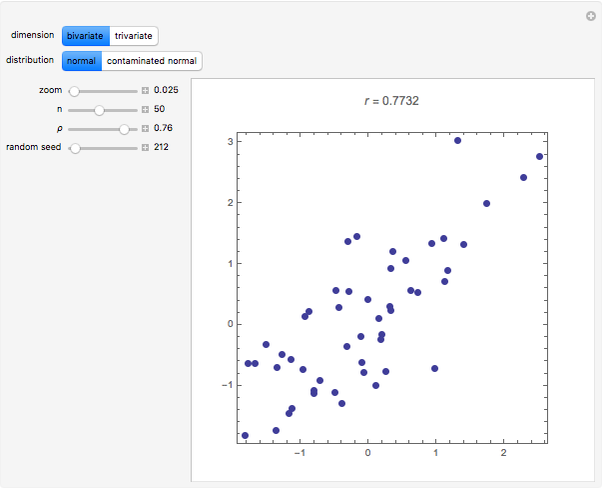
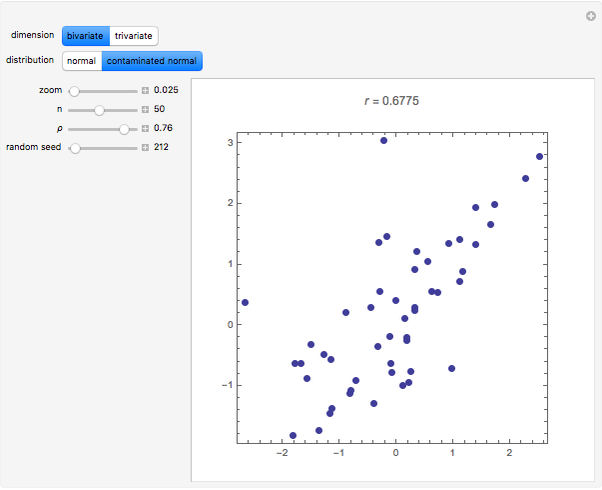
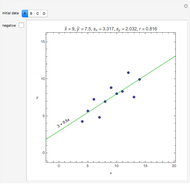
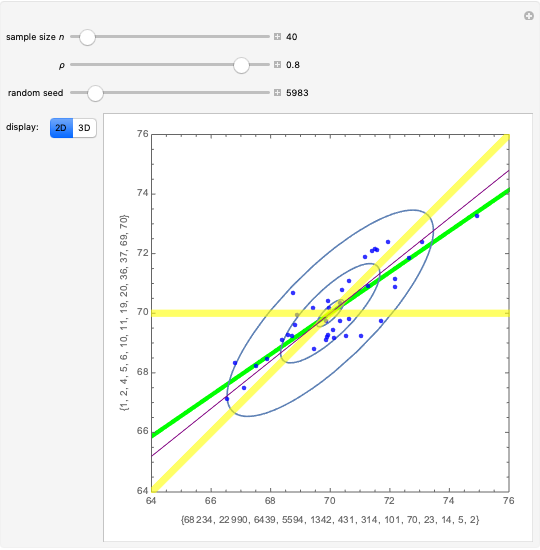
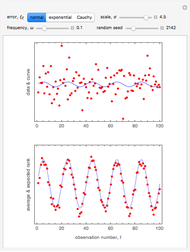
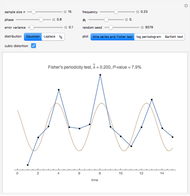
For dimension two, we have either the bivariate normal with unit variances, mean zero, and correlation parameter  , or, in the contaminated case (with a 10% probability), the observation is replaced with one from the same distribution but multiplied by 3. The contaminated distribution is sometimes used to describe non-normal data with a higher proportion of outliers than the normal. The estimated correlation
, or, in the contaminated case (with a 10% probability), the observation is replaced with one from the same distribution but multiplied by 3. The contaminated distribution is sometimes used to describe non-normal data with a higher proportion of outliers than the normal. The estimated correlation  is shown and reflects the pattern seen in the data, but it may not be an accurate estimator of
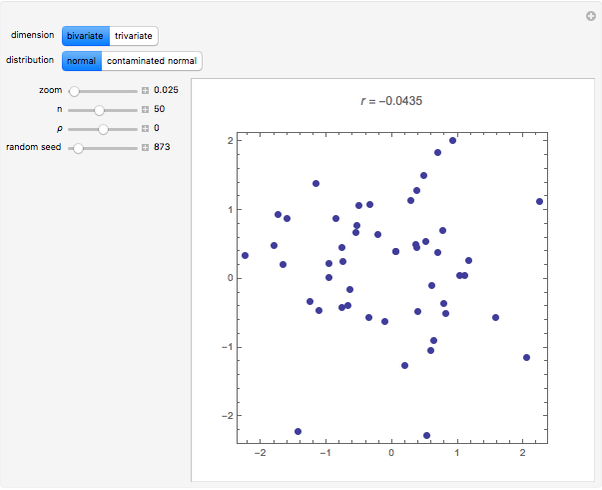
is shown and reflects the pattern seen in the data, but it may not be an accurate estimator of  for small
for small  even in the normal case. Increasing
even in the normal case. Increasing  increases the accuracy of the estimator
increases the accuracy of the estimator  . If
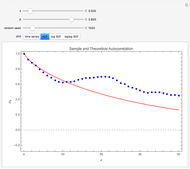
. If  is kept fixed, the variability of the estimator
is kept fixed, the variability of the estimator  decreases as the absolute magnitude of
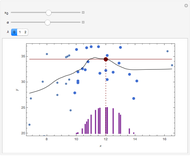
decreases as the absolute magnitude of  is increased. This is seen by varying the seed and then experimenting with different
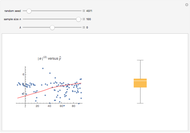
is increased. This is seen by varying the seed and then experimenting with different  . As we zoom out, our perception may spuriously suggest that the association between the variables increases. Using the contaminated normal distribution increases the variability in our estimate
. As we zoom out, our perception may spuriously suggest that the association between the variables increases. Using the contaminated normal distribution increases the variability in our estimate  and the likelihood of an apparent spurious association when
and the likelihood of an apparent spurious association when  .
.
Contributed by: Ian McLeod (March 2011)
Open content licensed under CC BY-NC-SA
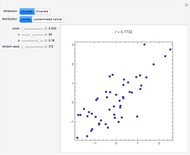
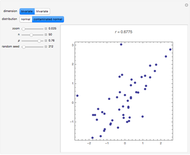
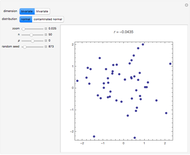
Snapshots
Details
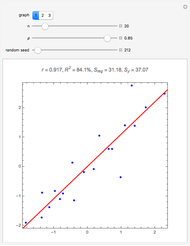
In the bivariate normal case, this Demonstration provides a dynamic and more accurate visualization of figure 4.5 in [1]. The illusion that the degree of association increases when we zoom out was discussed in [2]. The contaminated normal distribution was proposed as a realistic model for outliers in [3].
[1] D. S. Moore, The Basic Practice of Statistics, New York: W. H. Freeman and Company, 2010.
[2] W. S. Cleveland, P. Diaconis, and R. McGill, "Variables on Scatterplots Look More Highly Correlated When the Scales Are Increased," Science, 216(4550), 1982 pp. 1138–1141.
[3] J. W. Tukey, "A Survey of Sampling from Contaminated Distributions," Contributions to Probability and Statistics (I. Olkin, ed.), Stanford: Stanford University Press, 1960 pp. 448–485.
Permanent Citation