Volume of a Cylinder Cut from a Rectangle

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
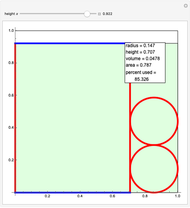
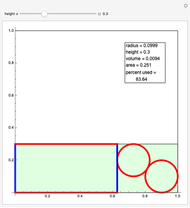
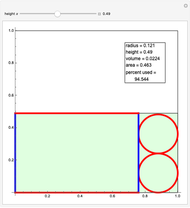
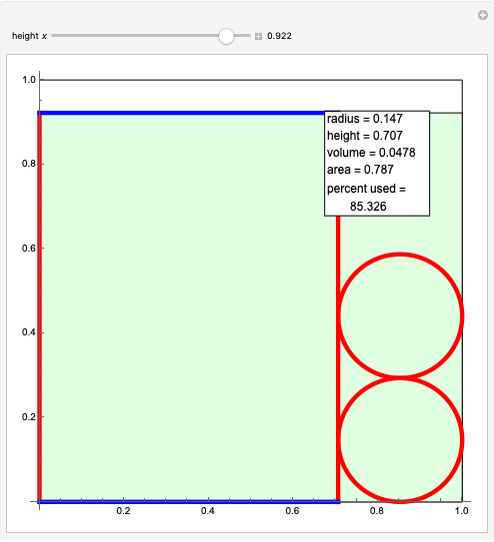
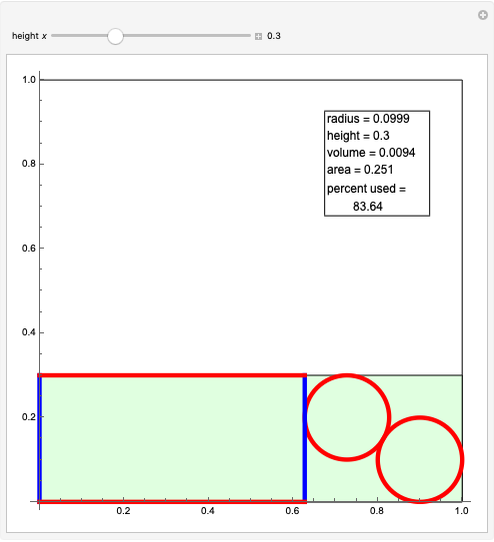
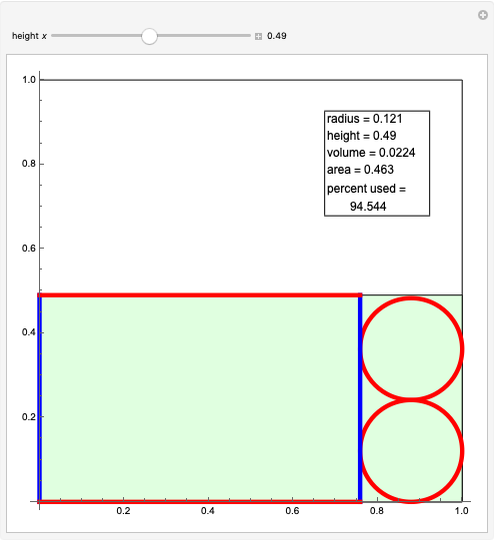
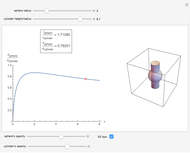
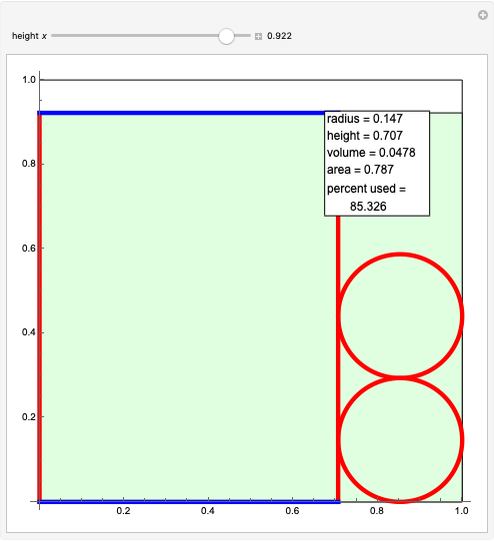
Maximize the volume of the cylinder cut from a 1×x rectangle ( ) with no more than three pieces.
) with no more than three pieces.
Contributed by: Carl P. McCarty (August 2022)
Open content licensed under CC BY-NC-SA
Snapshots
Details
A standard problem in elementary calculus is to maximize the volume of a cylinder for a fixed surface area. It is assumed that there is no wasted area. Suppose, however, that the two circular caps and body of the cylinder must be cut from a rectangular piece of metal. This Demonstration shows how to maximize the volume of the cylinder. The problem can be simplified by dividing the sides of the rectangle by the longer dimension and considering instead a 1×x rectangle with  .
.
Use the slider "height  " to set the smaller side and display how to cut the metal. The caps and circumference are outlined in red, while the height is shown in blue. The inset shows the radius, height, volume, area and the percentage of the metal used in the construction. The maximum volume is obtained with
" to set the smaller side and display how to cut the metal. The caps and circumference are outlined in red, while the height is shown in blue. The inset shows the radius, height, volume, area and the percentage of the metal used in the construction. The maximum volume is obtained with  .
.
Permanent Citation