Wolfram Demonstrations Project

12,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

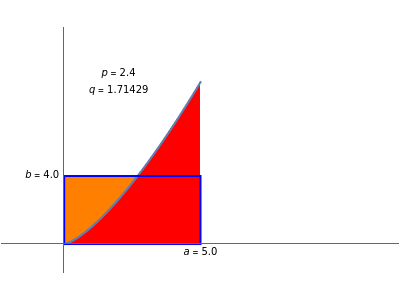
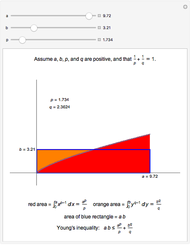
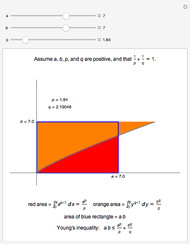
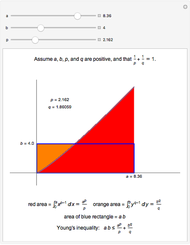
Young's Inequality

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

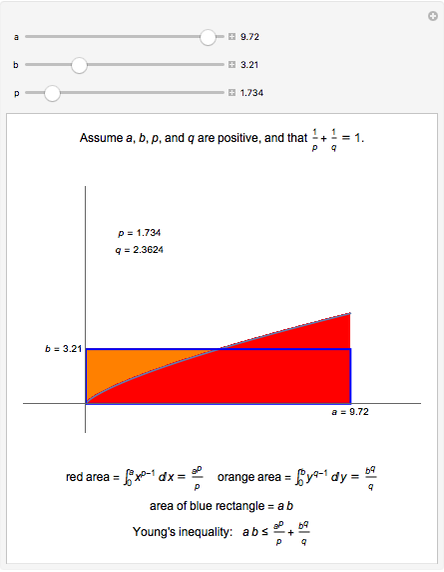
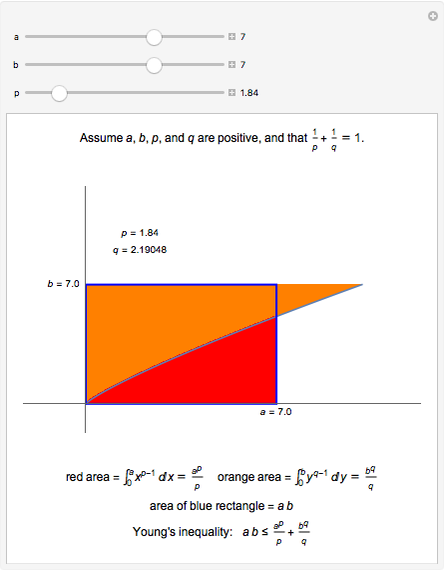
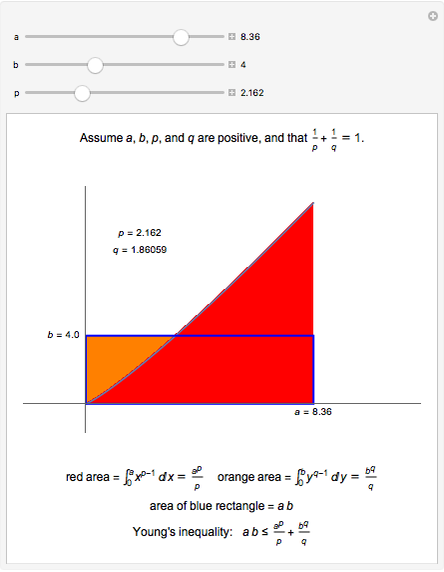
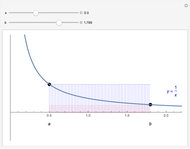
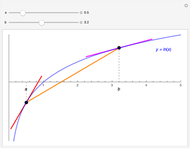
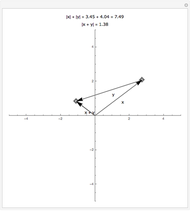
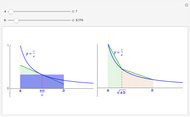
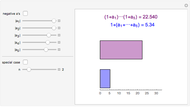
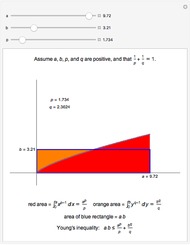
Given positive numbers and whose reciprocals add up to one, the product 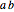of two positive real numbers and is less than or equal to a weighted sum of 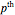and 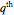powers of and .

Contributed by: Ken Levasseur (December 2013)
(UMass Lowell)
Open content licensed under CC BY-NC-SA

Snapshots

Details

References

[1] R. B. Nelson, Proofs without Words II, More Exercises in Visual Thinking, Washington, DC: Mathematical Association of America, 2000.

[2] W. H. Young, "On Classes of Summable Functions and Their Fourier Series," Proceedings of the Royal Society A, 87, 1912 pp. 225–229.

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send