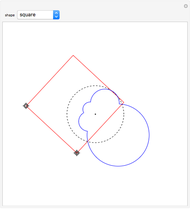
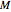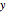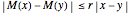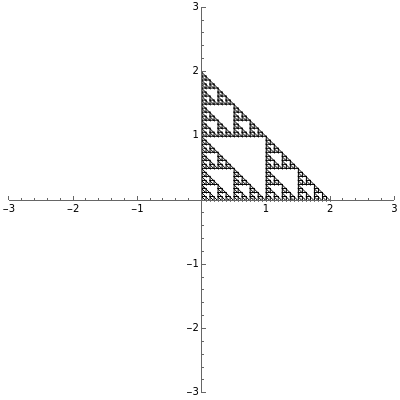
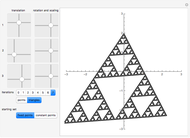
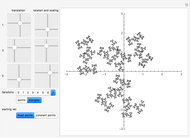
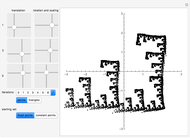
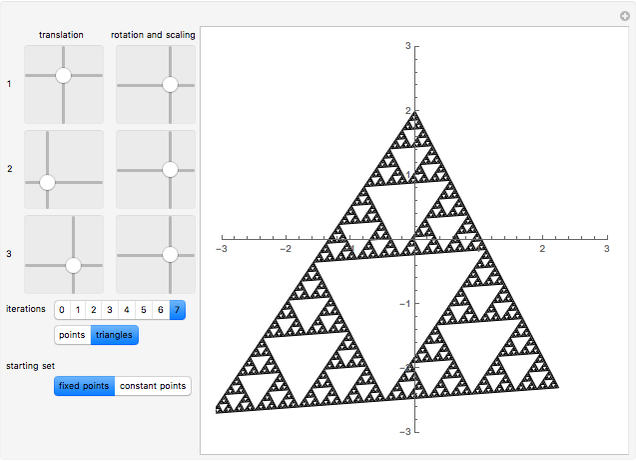
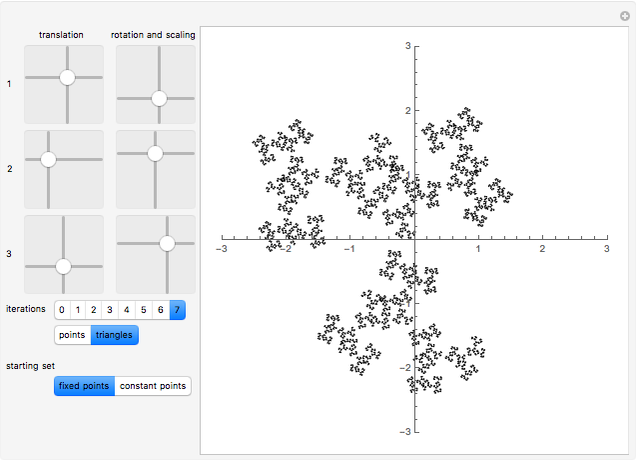
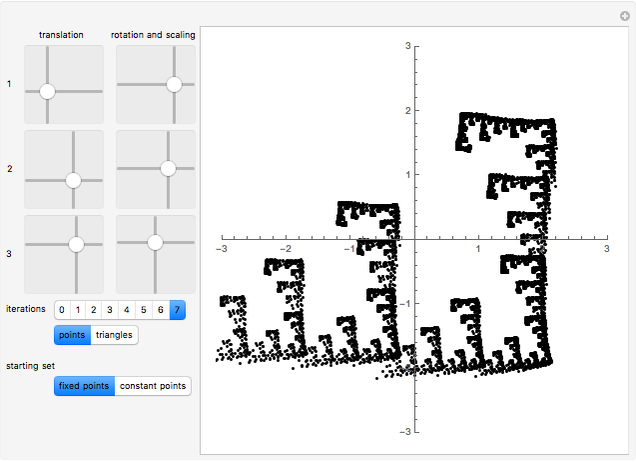
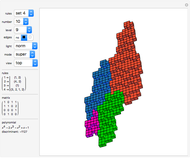
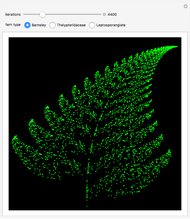
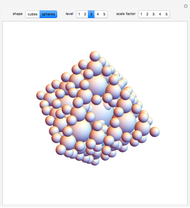
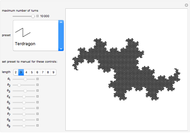
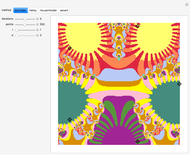
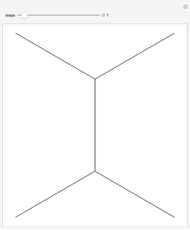
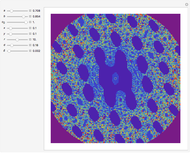
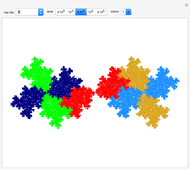
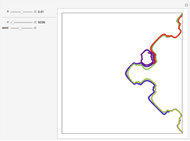
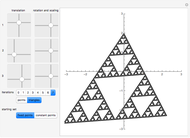
A map is a contraction mapping if for all points , , , where 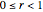. A similitude is a contraction mapping that is a composition of dilations, rotations, translations, and reflections. A two-dimensional Hutchinson operator maps a plane figure to the union of its images under a finite collection of similitudes. The orbit of a plane figure under such an operator can form a self-similar fractal. In this Demonstration you can vary three similitudes (without reflection) to see what self-similar fractals are possible.