Gabor 3D

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
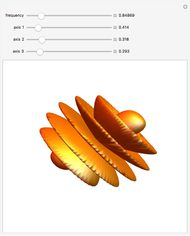
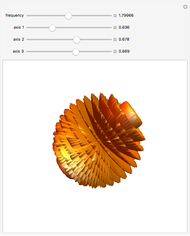
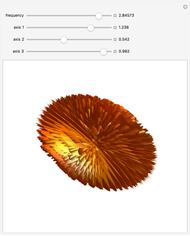
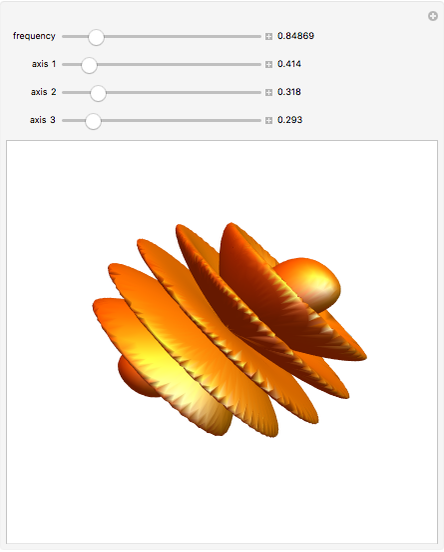
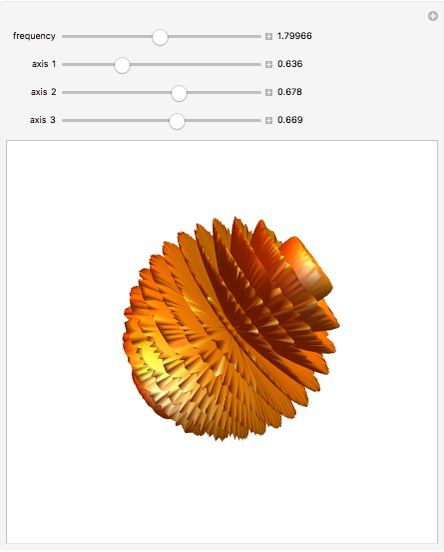
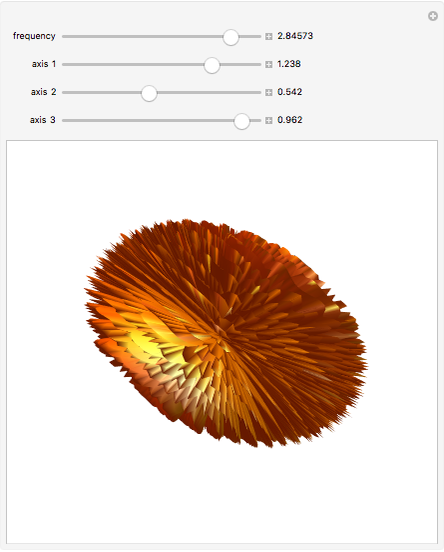
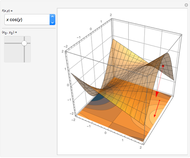
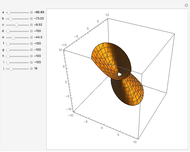
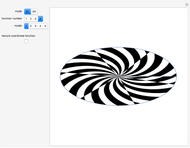
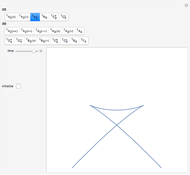
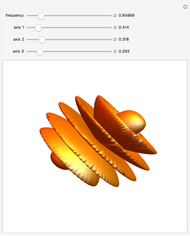
A 3D Gabor is the product of a 3D Gaussian and a 3D harmonic function. The length of the axes is controlled by the Gaussian and the frequency is controlled by the harmonic function. 3D Gabor wavelets are used for spatio-temporal analysis of a three-dimensional signal, like a video sequence, to extract motion energy features.
Contributed by: Fiora Pirri (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
References
D. Gabor, "Theory of Communication," J. IEE, Part III, 93(26), 1946 pp. 429–457.
J. G. Daugman, "Complete Discrete 2D Gabor Transforms by Neural Networks for Image Analysis and Compression,” IEEE Transaction on Acoustics, Speech, and Signal Processing, 36(7), 1988 pp. 1169–1179.
I. R. Fasel, M. S. Bartlett, and J. R. A. Movellan,"A Comparison of Gabor Filter Methods for Automatic Detection of Facial Landmarks," in 5th IEEE International Conference on Automatic Face and Gesture Recognition (FGR 2002), Washington, DC: IEEE Computer Society pp. 242–248.
B. MacLennan, "Gabor Representations of Spatiotemporal Visual Images," Technical Report CS-91-144, Knoxville, TN: University of Tennessee, 1991.
D. J. Heeger, "Model for the Extraction of Image Flow," Journal of the Optical Society of America A: Optics, Image Science, and Vision, 4(8), 1987 pp. 1455–1471.
Permanent Citation
"Gabor 3D"
http://demonstrations.wolfram.com/Gabor3D/
Wolfram Demonstrations Project
Published: March 7 2011