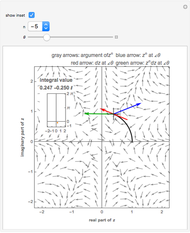
Integration using Hermite Reduction

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
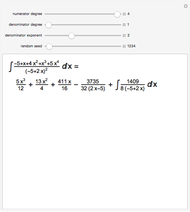
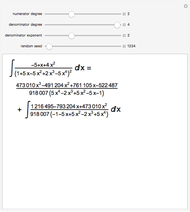
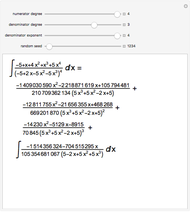
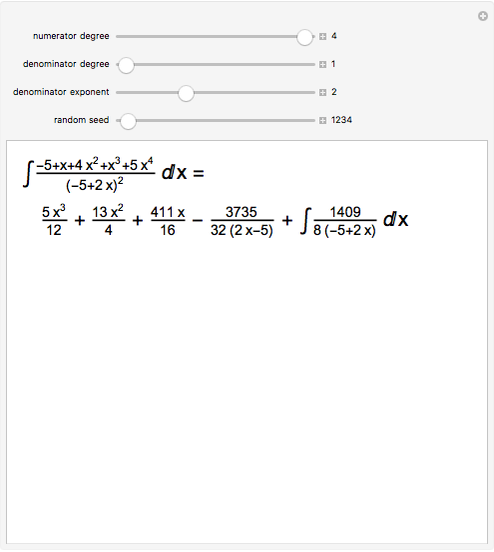
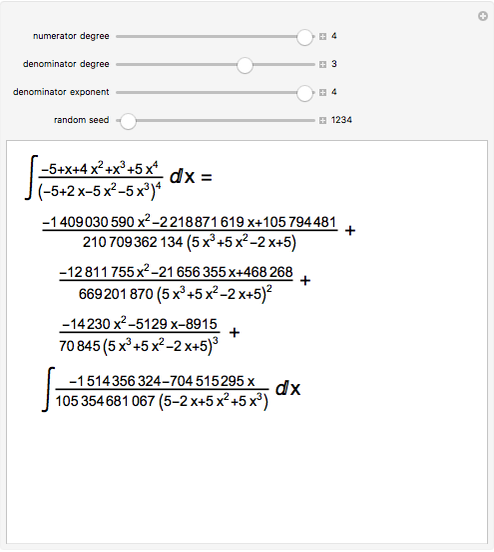
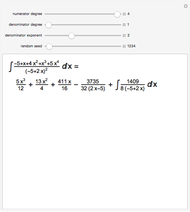
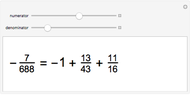
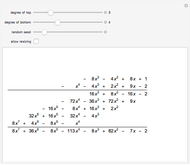
From Liouville's theorem, every rational function  is integrable in terms of elementary functions and takes the form
is integrable in terms of elementary functions and takes the form  , where
, where  and
and  for
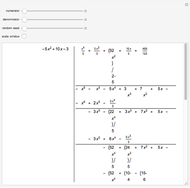
for  . Mack's linear version of Hermite reduction is a factor-free method for finding the rational part
. Mack's linear version of Hermite reduction is a factor-free method for finding the rational part  of an integral. This is a major computational advantange over the partial fraction method (Bernoulli algorithm) taught to calculus students, as it requires no knowledge of the roots of the integrands' denominator. Hermite reduction can be generalized to transcendental and algebraic functions.
of an integral. This is a major computational advantange over the partial fraction method (Bernoulli algorithm) taught to calculus students, as it requires no knowledge of the roots of the integrands' denominator. Hermite reduction can be generalized to transcendental and algebraic functions.
Contributed by: Sam Blake (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
detailSectionParagraphPermanent Citation
"Integration using Hermite Reduction"
http://demonstrations.wolfram.com/IntegrationUsingHermiteReduction/
Wolfram Demonstrations Project
Published: March 7 2011