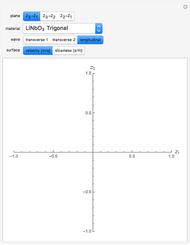
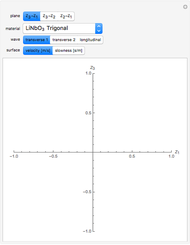
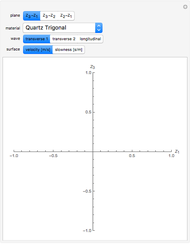
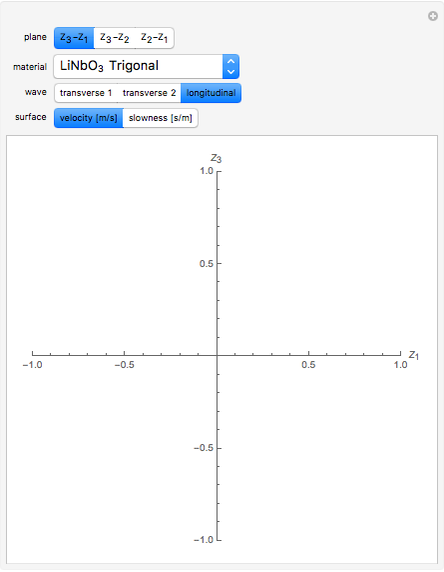
2D Acoustic Velocities in Crystalline Materials

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
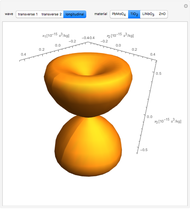
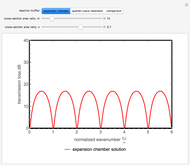
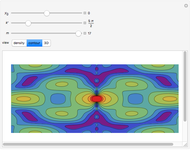
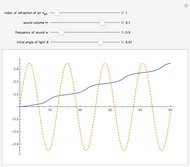
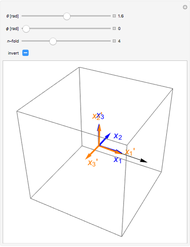
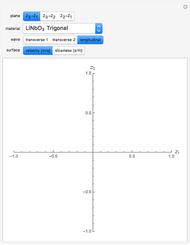
The velocity of sound in anisotropic materials is directionally dependent. Three wave velocities may exist in any given direction: two transverse waves (primary oscillation perpendicular to the wave propagation direction) and one longitudinal wave (polarization in the same direction as the propagation). You can select the plane on which to view the velocity and whether to view the velocity surface or the inverse velocity (slowness).
Contributed by: Robert McIntosh (March 2014)
Open content licensed under CC BY-NC-SA
Snapshots
Details
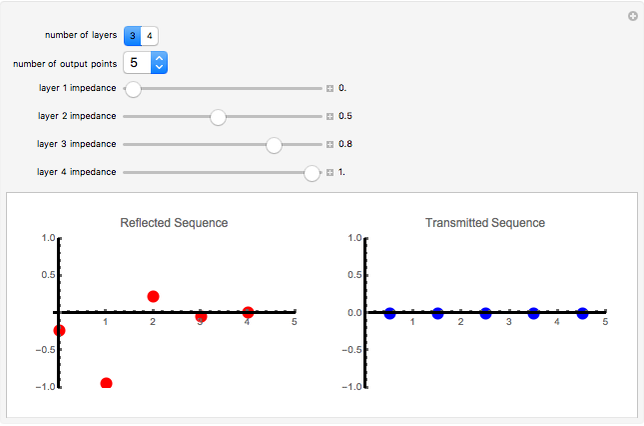
The velocity  of sound in materials as a function of the elastic constant
of sound in materials as a function of the elastic constant  and the density
and the density  is given by
is given by  . Velocity calculations can often be very simple, but because of the anisotropy of many materials the elastic constant
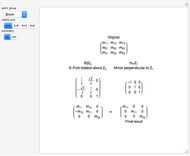
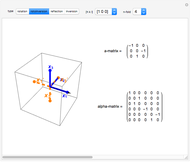
. Velocity calculations can often be very simple, but because of the anisotropy of many materials the elastic constant  is actually a 6×6 matrix. Solving for the velocity of the wave is done by using the Christoffel equation. This Demonstration also considers the contribution from the piezoelectric coupling. An excellent treatment of solving the Christoffel equation can be found in [4].
is actually a 6×6 matrix. Solving for the velocity of the wave is done by using the Christoffel equation. This Demonstration also considers the contribution from the piezoelectric coupling. An excellent treatment of solving the Christoffel equation can be found in [4].
The coefficients used are from a variety of sources:
References
[1] M. J. Weber, Handbook of Optical Materials, Boca Raton, FL: CRC Press, 2003.
[2] Z. Rui, J. Bei, W. Jiang, and W. Cao, "Anisotropy in Domain Engineered 0.92Pb(
 )
) -0.08PbTi
-0.08PbTi Single Crystal and Analysis of Its Property Fluctuations," Ultrasonics, Ferroelectrics and Frequency Control, 49(12), 2002 pp. 1622–1627. doi:10.1109/TUFFC.2002.1159841.
Single Crystal and Analysis of Its Property Fluctuations," Ultrasonics, Ferroelectrics and Frequency Control, 49(12), 2002 pp. 1622–1627. doi:10.1109/TUFFC.2002.1159841.
[3] R. Zhang, W. Jiang, B. Jiang, and W. Cao, "Elastic, Dielectric and Piezoelectric Coefficients Domain Engineered 0.70Pb(
 )
) -0.30PbTi
-0.30PbTi Single Crystals," in AIP Conference Proceedings, 626, 2002 pp. 188–197. doi:10.1063/1.1499567.
Single Crystals," in AIP Conference Proceedings, 626, 2002 pp. 188–197. doi:10.1063/1.1499567.
[4] R. E. Newnham, Properties of Materials, New York: Oxford University Press, 2005.
[5] COMSOL Multiphysics, Materials Database Version 4.3, Burlington, MA: COMSOL, Inc., 2012.
[6] L. Liang, Y. L. Li, S. Y. Hu, L-Q. Chen, and G-H. Lu, "Piezoelectric Anisotropy of a KNb Single Crystal," Journal of Applied Physics, 108(9), 2010 pp. 094111–094119. doi:10.1063/1.3511336.
Single Crystal," Journal of Applied Physics, 108(9), 2010 pp. 094111–094119. doi:10.1063/1.3511336.
[7] J. Yin, B. Jiang, and W. Cao, "Elastic, Piezoelectric, and Dielectric Properties of 0.955Pb(
 )
) -0.45PbTi
-0.45PbTi Single Crystal with Designed Multidomains," IEEE Transactions on Ultrasonics, Ferroelectrics and Frequency Control, 47(1), 2000 pp. 285–291. doi:10.1109/58.818772.
Single Crystal with Designed Multidomains," IEEE Transactions on Ultrasonics, Ferroelectrics and Frequency Control, 47(1), 2000 pp. 285–291. doi:10.1109/58.818772.
[8] W. Jiang, R. Zhang, B. Jiang, and W. Cao, "Characterization of Piezoelectric Materials with Large Piezoelectric and Electromechanical Coupling Coefficients," Ultrasonics, 41(2), 2003 pp. 55–63. doi:10.1016/S0041-624X(02)00436-5.
[9] E. Wiesendanger, "Optical Properties of KNb ," Ferroelectrics, 1(1), 1970 pp. 141–148. doi:10.1080/00150197008241478.
," Ferroelectrics, 1(1), 1970 pp. 141–148. doi:10.1080/00150197008241478.
Permanent Citation