Contours of Constant Principal Angle in the Complex Dielectric Plane

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
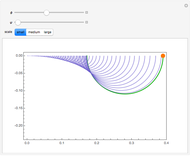
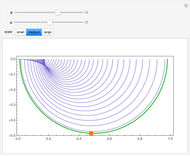
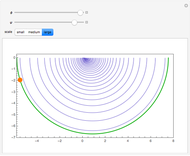
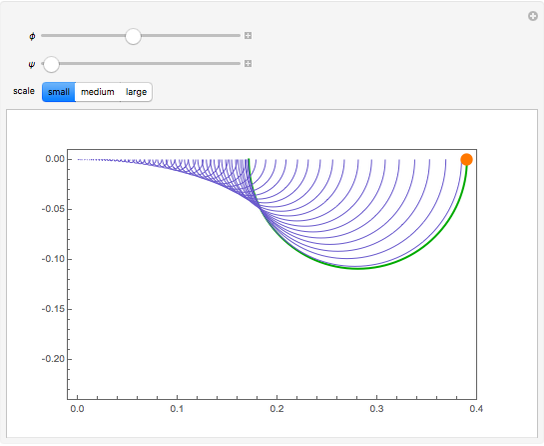
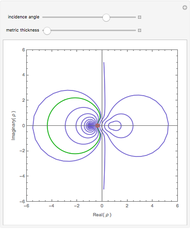
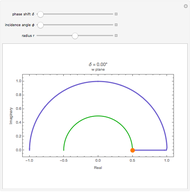
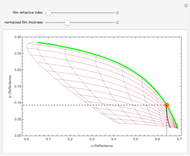
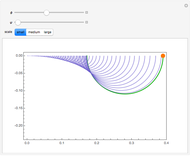
The contours of the constant principal angle  in the complex dielectric
in the complex dielectric  plane can be determined by eliminating the principal azimuth
plane can be determined by eliminating the principal azimuth  from the equation
from the equation 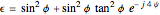 . This gives
. This gives  , which is a circle with center at
, which is a circle with center at 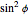 on the real axis and radius
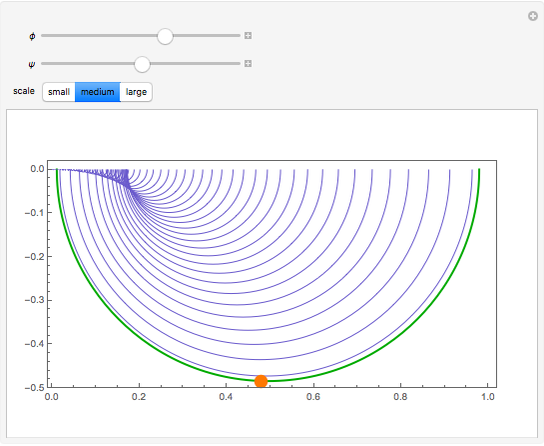
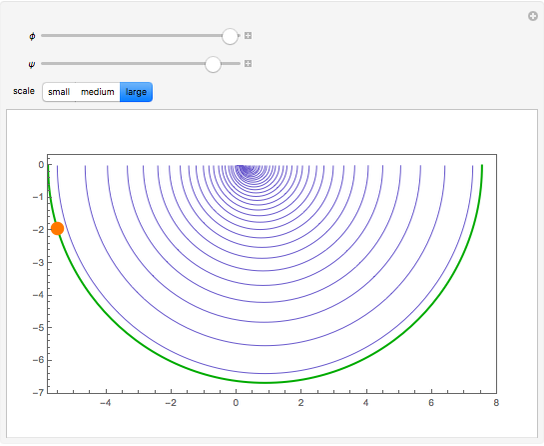
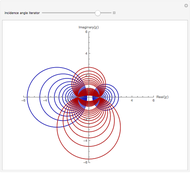
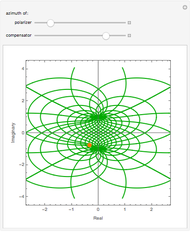
on the real axis and radius  . The figure shows constant principal-angle contours for
. The figure shows constant principal-angle contours for 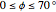 . As
. As  approaches
approaches 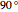 , these contours approach concentric circles with center at
, these contours approach concentric circles with center at  .
.
Contributed by: Siva Perla (March 2011)
Open content licensed under CC BY-NC-SA
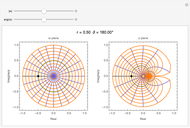
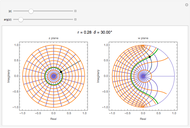
Snapshots
Details
The usual notation for the principal angle,  , and principal azimuth,
, and principal azimuth,  , has been modified for visual clarity.
, has been modified for visual clarity.
R. M. A. Azzam, "Contours of constant principal angle and constant principal azimuth in the complex ϵ plane," Journal of the Optical Society of America, 71(12), 1981 pp. 1523-1528.
Permanent Citation