Effects of a Force on the Orbit of an Artificial Satellite

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
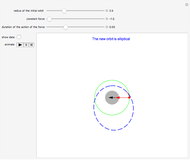
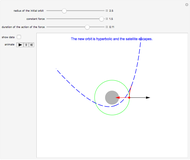
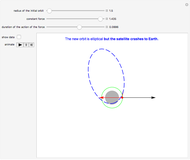
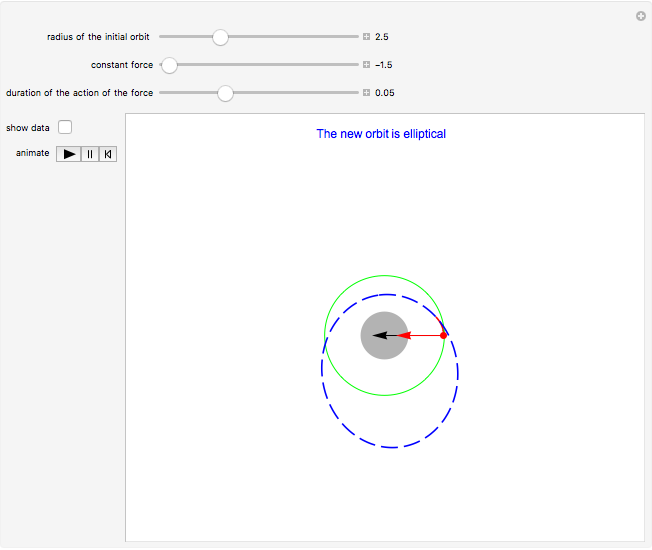
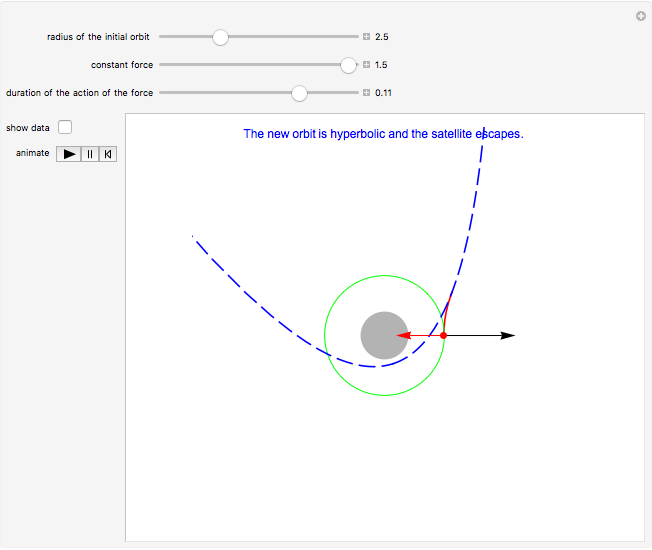
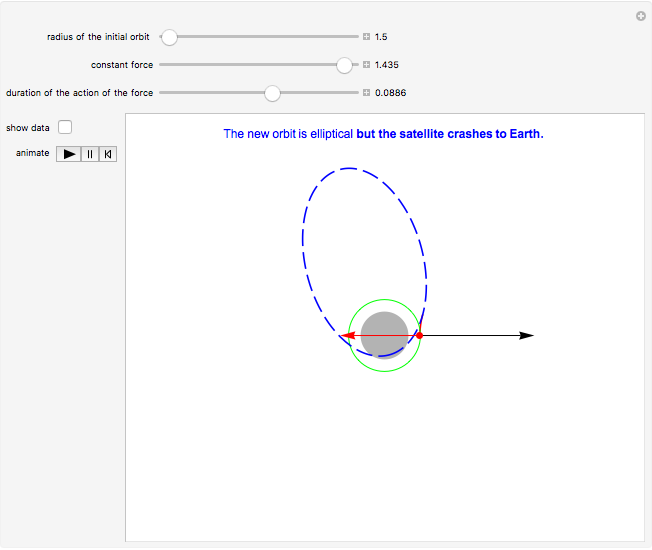
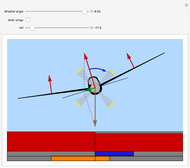
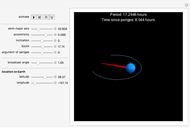
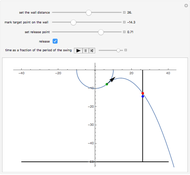
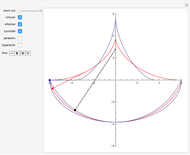
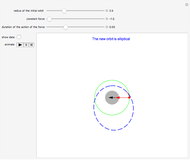
A 10,000 kg artificial satellite is initially rotating around Earth in a circular orbit. Then a force, such as that provided by a rocket attached to the satellite, acts on it for a certain amount of time. We assume that the force is always directed along the line connecting the centers of gravity of Earth and the satellite, it has a constant magnitude, and it is directed either toward or away from Earth. During this time the satellite exits its original orbit and follows a path that is determined by Earth's gravitational force on the satellite, displayed as a red arrow, and the force of the rocket, displayed as a black arrow. When the force of the rocket is turned off, the satellite is then in a new orbit that is either elliptical or hyperbolic. Depending on the strength and the duration of the action of the force of the rocket, the new orbit of the satellite may cross Earth's surface causing the satellite to crash on the Earth surface. This may happen when the force of the rocket is pointing toward Earth or away from Earth.
Contributed by: Stelios Kapranidis (May 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
The motion of a particle under the action of a conservative force that always points toward a fixed point (i.e. a conservative central force) is governed by two conservation principles. Conservation of energy is implied because the force is conservative; conservation of angular momentum holds because the torque of the force with respect to the fixed point is zero. The following formulas use polar coordinates; see [1].
Conservation of angular momentum gives:
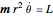 , (1)
, (1)
where  is the constant angular momentum of the particle. Conservation of energy gives:
is the constant angular momentum of the particle. Conservation of energy gives:
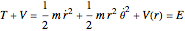 , (2)
, (2)
where 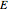 is the constant energy of the particle,
is the constant energy of the particle, 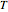 is the kinetic energy, and
is the kinetic energy, and  is the potential energy, which is a function of only the distance
is the potential energy, which is a function of only the distance  . The conservative force acting on the particle is
. The conservative force acting on the particle is 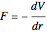 .
Equation (2) gives:
.
Equation (2) gives:
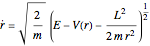
and
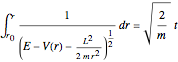 . (3)
. (3)
From equation (3) we can obtain 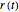 , which, used in equation (1), gives:
, which, used in equation (1), gives:
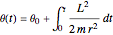 . (4)
. (4)
The constants 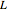 and
and 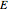 are evaluated from the initial position and velocity of the particle.
are evaluated from the initial position and velocity of the particle.
These equations can be used to calculate the motion of an artificial satellite of mass 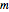 in orbit around Earth. If the only force acting on the satellite is Earth's gravitational force, we have:
in orbit around Earth. If the only force acting on the satellite is Earth's gravitational force, we have:
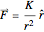 ,
,
where 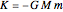 ,
,  is the mass of Earth, and
is the mass of Earth, and 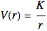 .
.
It is well known that in this case the orbit of the satellite is a conic section. The orbit is elliptical, parabolic, or hyperbolic according to whether the energy 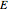 is negative, zero, or positive, respectively. Parabolic orbits are highly unlikely, because the energy
is negative, zero, or positive, respectively. Parabolic orbits are highly unlikely, because the energy 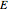 must be exactly zero. In the case of an elliptical or hyperbolic orbit, the equation of the orbit is:
must be exactly zero. In the case of an elliptical or hyperbolic orbit, the equation of the orbit is:
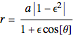 , (5)
, (5)
where 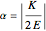 is the semimajor axis of the orbit and
is the semimajor axis of the orbit and 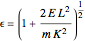 is the eccentricity of the orbit. Then
is the eccentricity of the orbit. Then
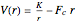 (6)
(6)
and we have:
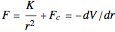 .
.
In this Demonstration the satellite is originally moving in a circular orbit of radius  . In this case the gravitational force of Earth acts as a centripetal force and the speed of the satellite
. In this case the gravitational force of Earth acts as a centripetal force and the speed of the satellite  is constant with
is constant with 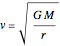 . A force
. A force 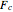 constant in magnitude and directed along the line that connects the centers of gravity of Earth and the satellite is activated when the satellite is at position
constant in magnitude and directed along the line that connects the centers of gravity of Earth and the satellite is activated when the satellite is at position 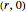 . This force can be chosen to point either toward or away from Earth.
. This force can be chosen to point either toward or away from Earth. 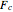 acts on the satellite for a finite amount of time. During this time the position of the satellite is calculated from equations (3) and (4) using equation (6) for
acts on the satellite for a finite amount of time. During this time the position of the satellite is calculated from equations (3) and (4) using equation (6) for 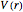 . When the force
. When the force 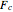 is turned off,
is turned off, 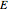 and
and 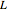 are evaluated at that time and the new orbit is calculated using equation (5).
are evaluated at that time and the new orbit is calculated using equation (5).
Reference
[1] K. R. Symon, Mechanics, 3rd. ed., Reading, MA: Addison–Wesley, 1971.
Permanent Citation