Integrating a Vector Field along a Curve

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
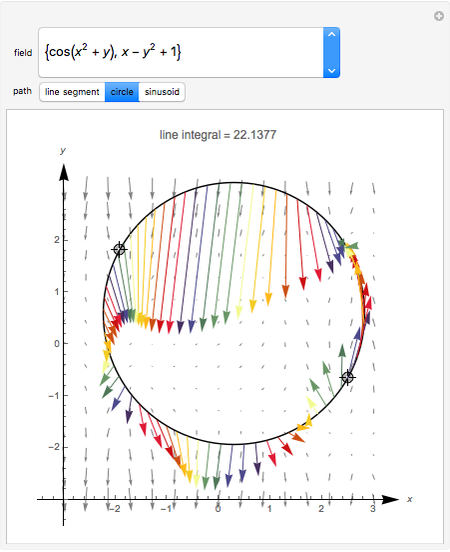
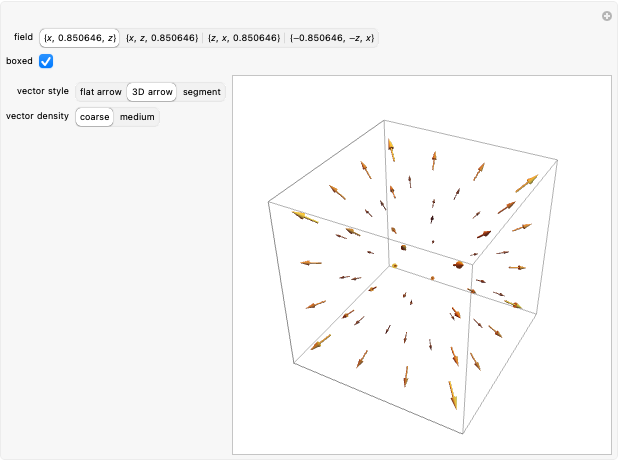
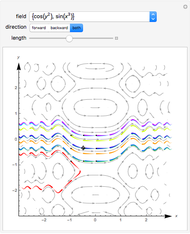
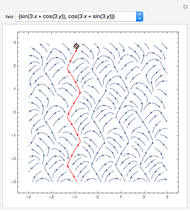
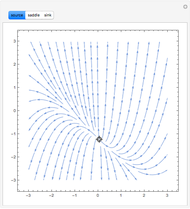
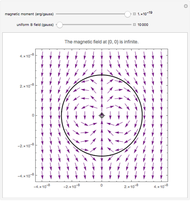
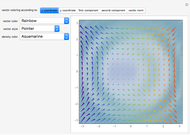
The line integral 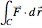 of the vector field
of the vector field 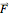 along the curve
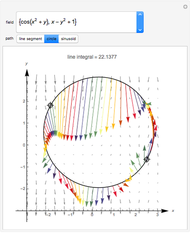
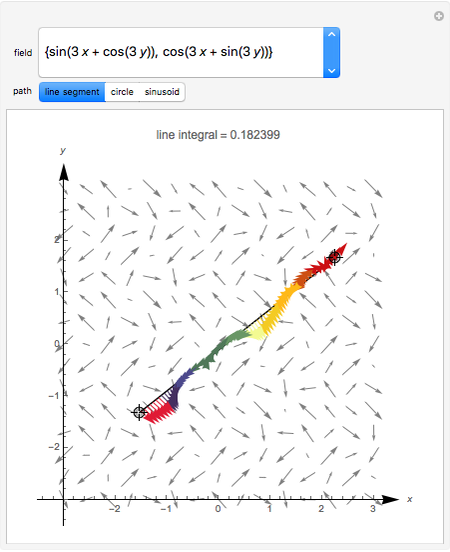
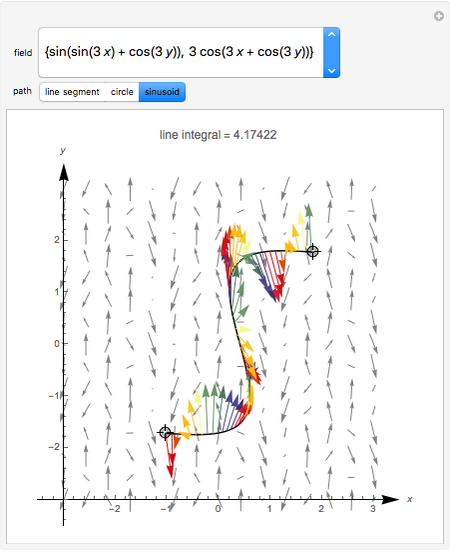
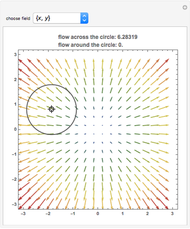
along the curve 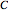 gives the work done by the field on an object moving along the curve through the field. A field is called conservative if only the starting and ending points matter; in a conservative field the work done around a closed curve is zero. The first two fields in the popup menu are conservative.
gives the work done by the field on an object moving along the curve through the field. A field is called conservative if only the starting and ending points matter; in a conservative field the work done around a closed curve is zero. The first two fields in the popup menu are conservative.
Contributed by: Gosia Konwerska (December 2008)
Open content licensed under CC BY-NC-SA
Snapshots
Details
detailSectionParagraphPermanent Citation
"Integrating a Vector Field along a Curve"
http://demonstrations.wolfram.com/IntegratingAVectorFieldAlongACurve/
Wolfram Demonstrations Project
Published: December 7 2008