Limit Pricing

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
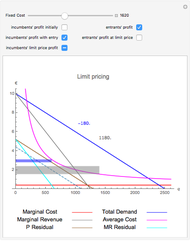
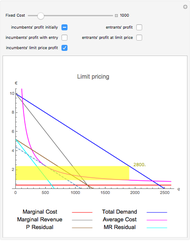
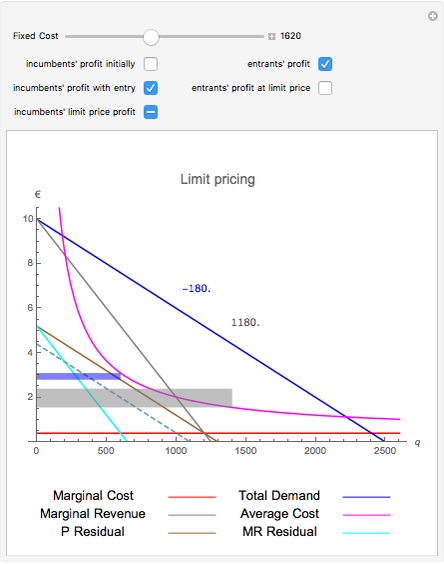
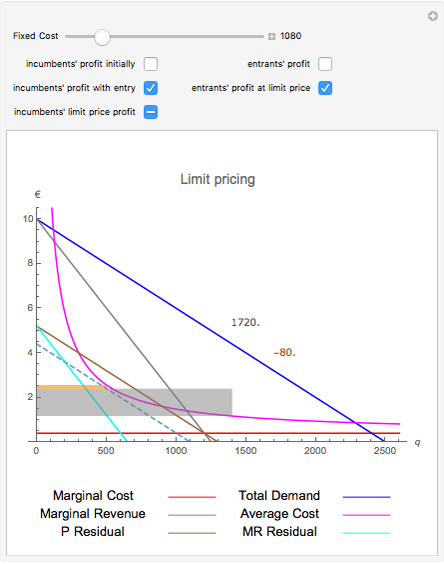
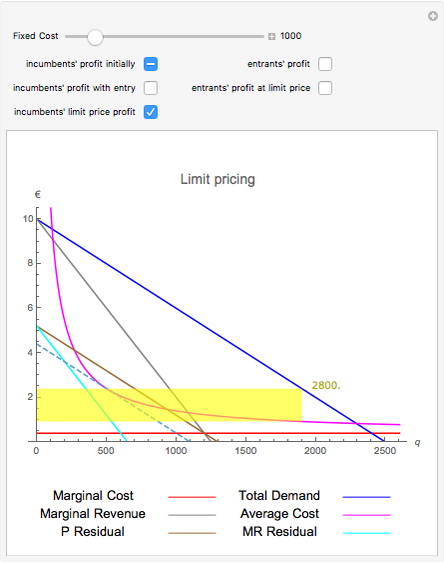
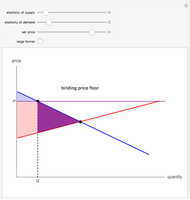
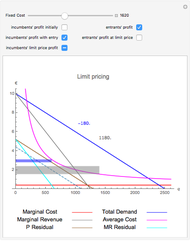
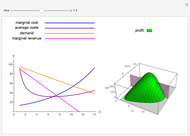
An incumbent is limit pricing if it produces more than its optimal quantity, so that there is not sufficient demand for a potential entrant. The lowest price that it sets is the "limit price" that excludes a profitable entry.
[more]
Contributed by: Christos Papahristodoulou (January 2014)
(Mälardalen University, Sweden)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Reference
[1] D. Carlton and J. Perloff, Modern Industrial Organization, 4th int. ed., Pearson Addison–Wesley, 2005.
Permanent Citation
"Limit Pricing"
http://demonstrations.wolfram.com/LimitPricing/
Wolfram Demonstrations Project
Published: January 10 2014