Particle in a Box: Probability Density and Positional Variance

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
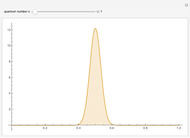
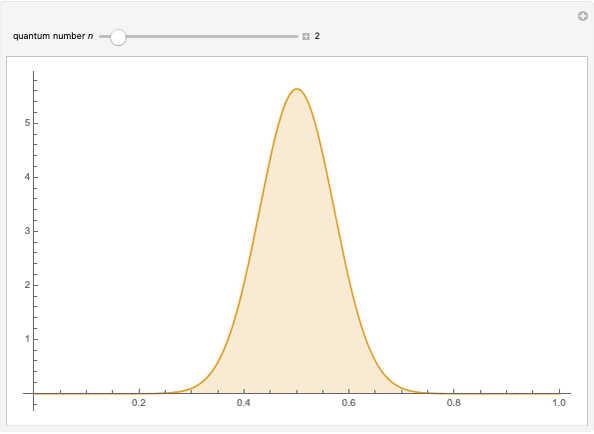
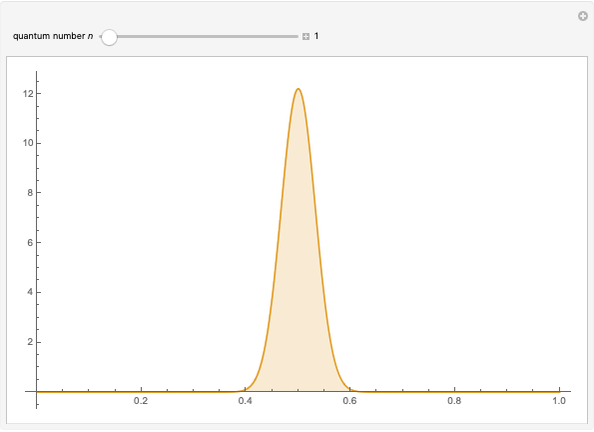
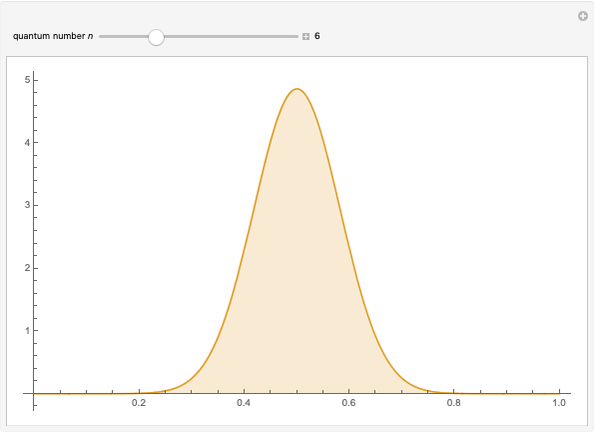
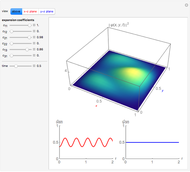
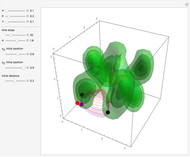
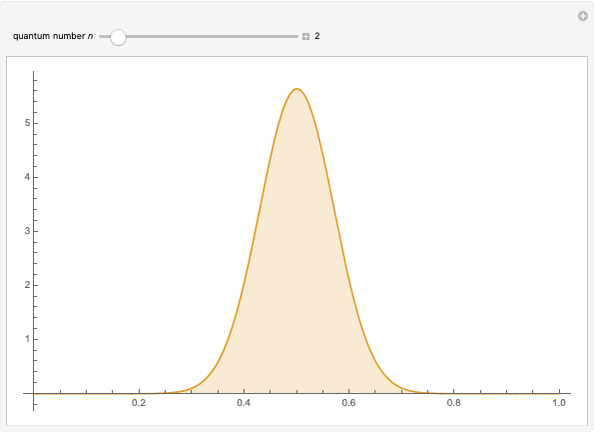
The square of the absolute value of the Schrödinger wavefunction equals the probability density of finding a particle at any point in a box. It is relatively straightforward to show that for all values of  , the average or expectation value of the position of the particle will be at the exact midpoint of the box (
, the average or expectation value of the position of the particle will be at the exact midpoint of the box ( , where
, where  is the length of the box) [1]. The variance, however, increases with increasing quantum number, to a limiting value of
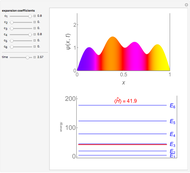
is the length of the box) [1]. The variance, however, increases with increasing quantum number, to a limiting value of  . This Demonstration plots the probability density (in blue) and a normal distribution (in yellow) with mean
. This Demonstration plots the probability density (in blue) and a normal distribution (in yellow) with mean  and variance
and variance  to represent the change in the variance with increasing
to represent the change in the variance with increasing  .
.
Contributed by: Daniel Barr (December 2020)
Open content licensed under CC BY-NC-SA
Snapshots
Details
The variance is  , where the expectation values
, where the expectation values  and
and  are obtained from
are obtained from  and
and  , respectively, where
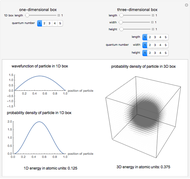
, respectively, where  is the length of the box. The (normalized) probability function for the one-dimensional particle in a box is given by [1]:
is the length of the box. The (normalized) probability function for the one-dimensional particle in a box is given by [1]:
 .
.
Reference
[1] D. A. McQuarrie and J. D. Simon, Physical Chemistry: A Molecular Approach, Sausalito, CA: University Science Books, 1997.
Permanent Citation