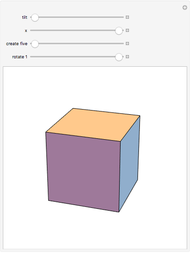
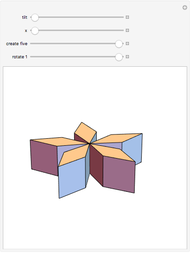
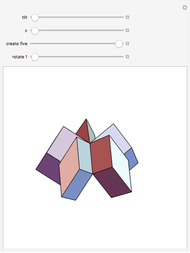
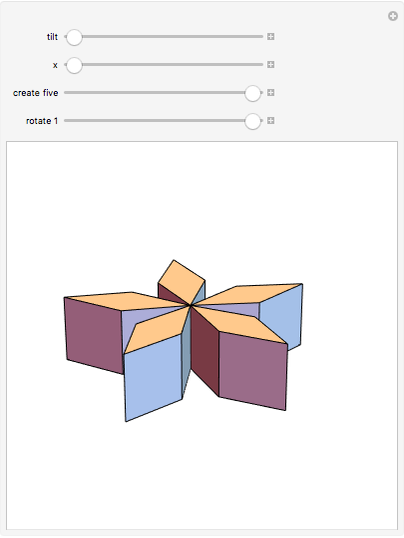
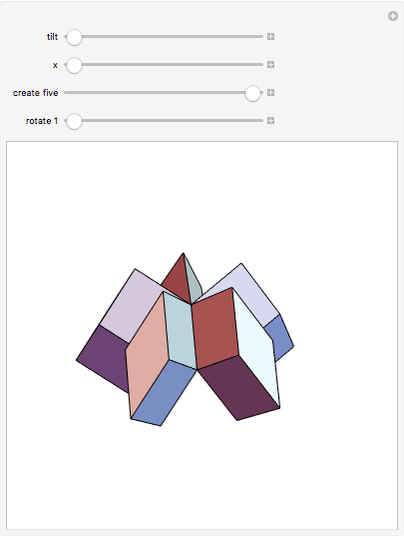
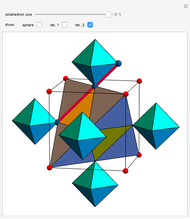
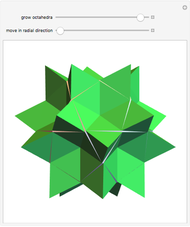
You can create various rhombohedra by tilting the four parallel edges of a cube or varying the face diagonals. This Demonstration focuses on the special case where the rhombohedron has four faces with diagonal ratio and two faces with diagonal ratio , where is the golden ratio. Let the edge of a cube be . Tilt four parallel edges by degrees, then reduce the diagonal of two faces to . One interesting feature of this polyhedron is that the distance between the two thin rhombi is the golden ratio. The half-diagonals of the thin rhombus are and 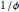. This illustrates an interesting relationship: , that is, .
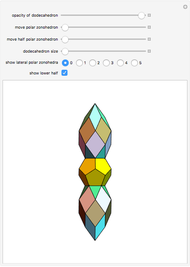
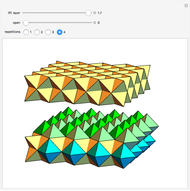
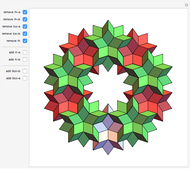
This rhombohedron could be considered as a building block in structures where rhombi with diagonal ratios or occur. The rhombic enneacontahedron is an example of a rhombus and a rhombus; the rhombic dodecahedron is a rhombus and the median rhombic triacontahedron is a rhombus.
![[Snapshot]](HTMLImages/index.en/thumbnail_1.gif)
![[Snapshot]](HTMLImages/index.en/thumbnail_2.gif)
![[Snapshot]](HTMLImages/index.en/thumbnail_3.gif)
Embed Interactive Demonstration 
Browse all topics
