Wolfram Demonstrations Project

13,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

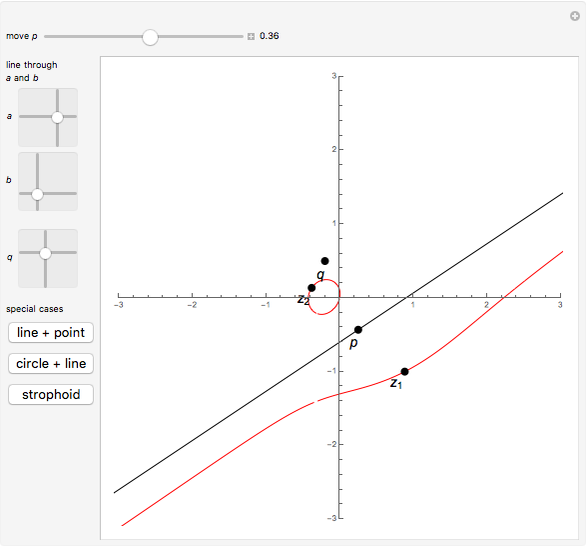
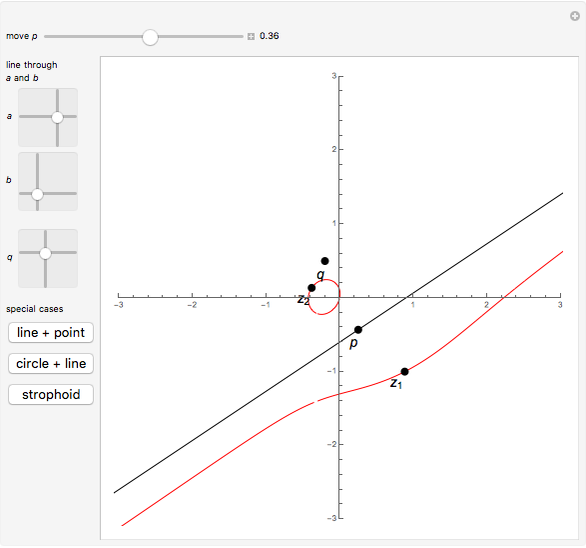
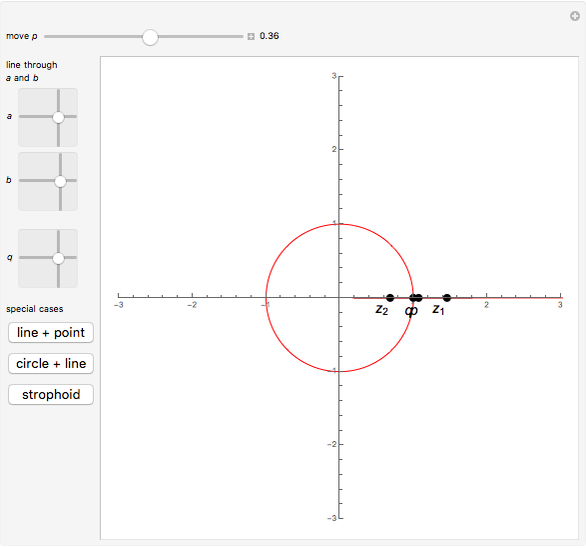
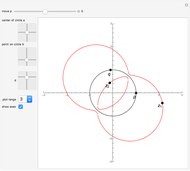
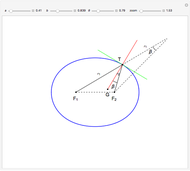
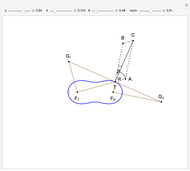
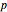4. Locus of the Solutions of a Complex Quadratic Equation

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

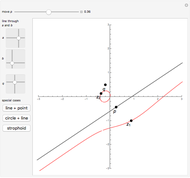
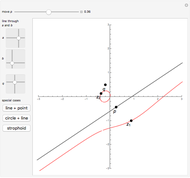
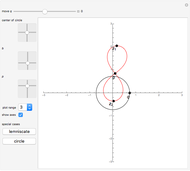
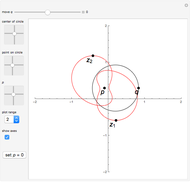
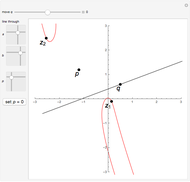
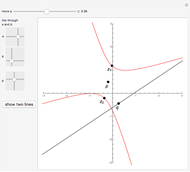
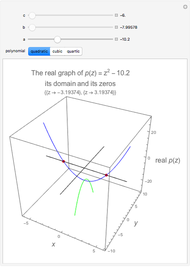
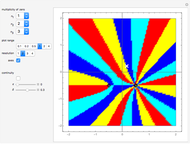
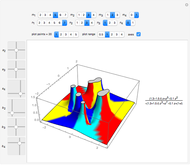
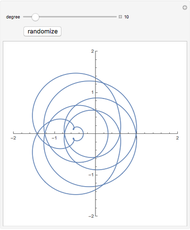
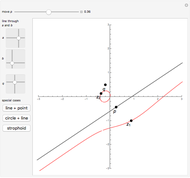
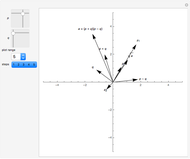
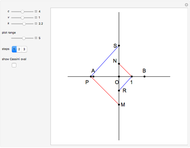
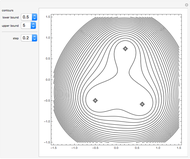
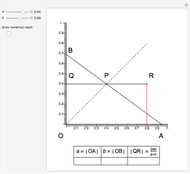
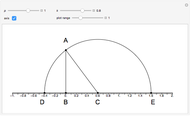
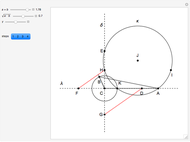
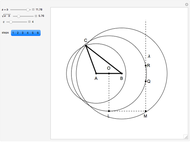
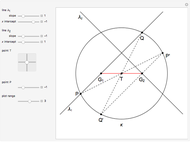
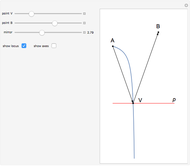
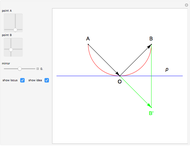
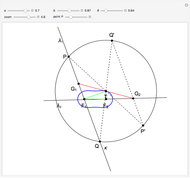
This Demonstration constructs the locus of the solutions of a complex quadratic equation 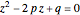, where is fixed and 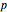moves along a line through and .

[more]

The locus is an algebraic curve of degree three. Special cases are the union of a circle and a line or a line and a point. If is a positive real number and moves on the vertical line through , the locus is a strophoid.

[less]

Contributed by: Marko Razpet and Izidor Hafner (October 2018)
Open content licensed under CC BY-NC-SA

Details

Snapshots

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send