Capacity Planning for Short Life Cycle Products: The Newsvendor Model

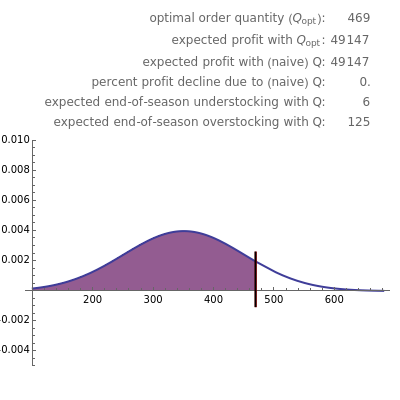
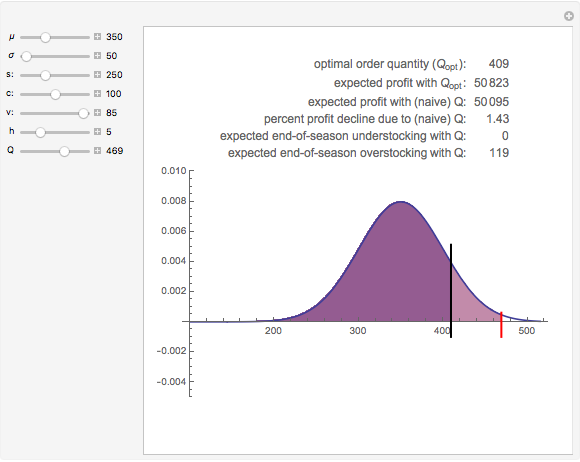
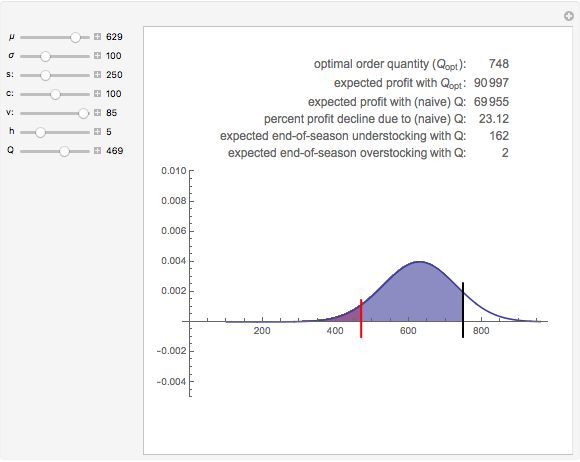
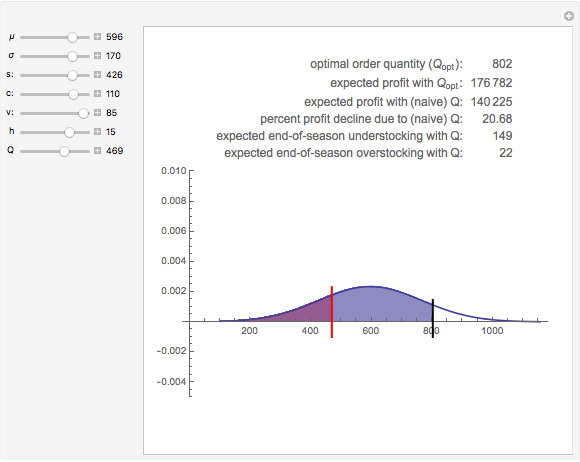
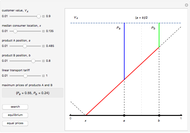
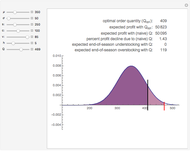
Although the newsvendor model in its very basic form involves a single product and a single period, it has been extended to multiple products, multiple processing points, and multiple periods. It is then referred to as a "newsvendor network". In addition, the risk aversion of decision makers can be incorporated into the basic and extended models. All these extensions, though, come at the expense of analytic tractability. However, numerical solutions are not too difficult to calculate even for large newsvendor networks, given the large-scale optimization engines available today. The analytic formula for the basic newsvendor model (for a continuous demand distribution) is to order at the critical fractile that equals . Note that in the example presented here this works out to be 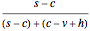==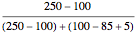0.88235. Upon inverting the cumulative distribution function of the distribution (N(350, 100)) at this critical fractile we get the optimal order quantity of 469 as depicted in the Demonstration. When the demand distribution is discrete, one should order the smallest quantity at which the cumulative distribution equals or exceeds the critical fractile.

: average (mean) demand

: standard deviation of demand

: unit selling price

: unit purchase cost

: unit markdown price for unsold items

: unit holding cost for unsold items

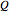: naive (or heuristic) order quantity

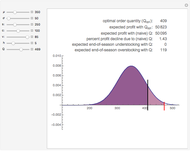
Snapshot 1: notice what happens when the demand distribution has less variance

Example from: S. Chopra and P. Meindl, Supply Chain Management, Pearson Higher Education, 2007.