3. Ambiguous Rings Based on a Rose Curve

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
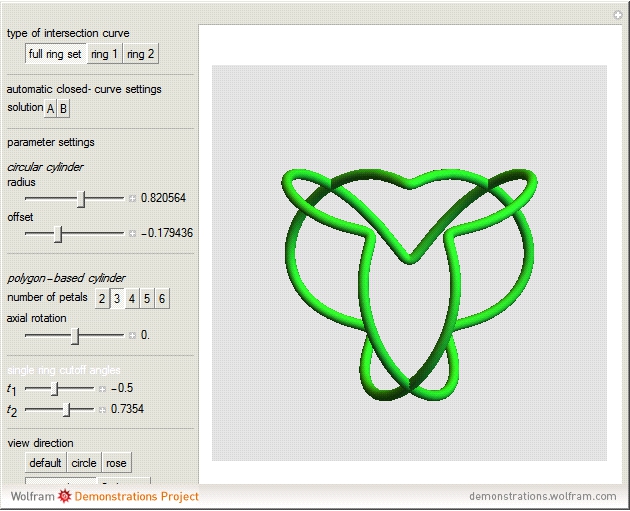
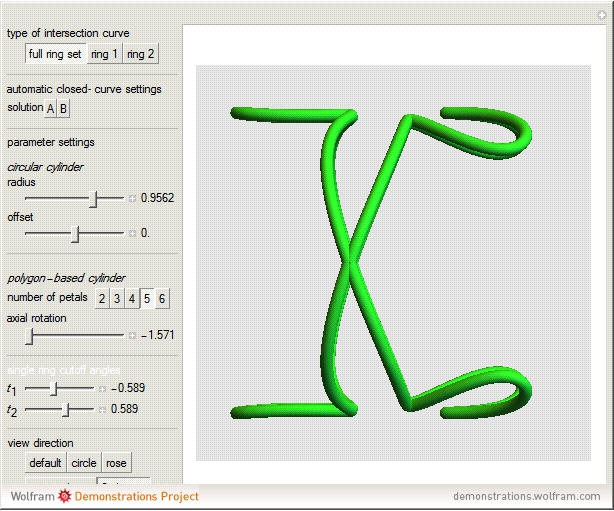
This Demonstration further explores ambiguous rings.
[more]
Contributed by: Erik Mahieu (May 2018)
Open content licensed under CC BY-NC-SA
Snapshots
Details
The parametric equation for  with radius
with radius  inclined at an angle
inclined at an angle  from the vertical is given by:
from the vertical is given by:
 .
.
The parametric equation of  in the
in the  -
- plane with
plane with  petals and an angular offset of
petals and an angular offset of  from the
from the  axis is given by:
axis is given by:
 .
.
To find the intersection, set the corresponding components equal. This gives three equations in four unknowns:  .
.
Eliminating  ,
,  and
and  by solving the equations gives the parametric curve of the intersection with
by solving the equations gives the parametric curve of the intersection with  as the only parameter:
as the only parameter:
 ,
,

with
 .
.
This composite curve (ring set) can be split into two rings. Therefore, the parameter range for  , from
, from  to
to  , is divided into sections using the cutoff angles
, is divided into sections using the cutoff angles  and
and  .
.
Reference
[1] E. Chicurel–Uziel, "Single Equation without Inequalities to Represent a Composite Curve," Computer Aided Geometric Design, 21(1), 2004 pp. 23–42. doi:10.1016/j.cagd.2003.07.011.
Permanent Citation