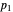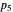Noncircular Roller Drive

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
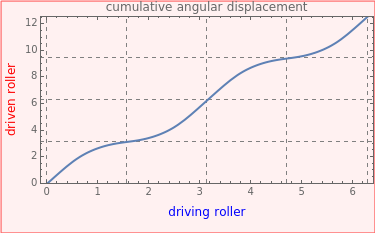
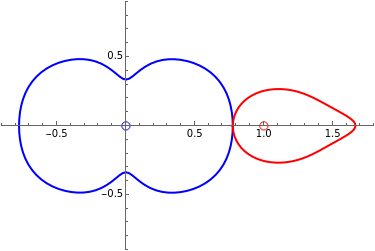
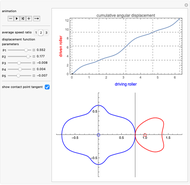
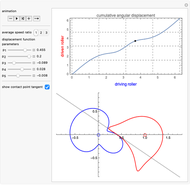
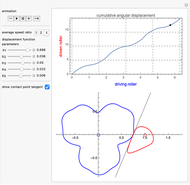
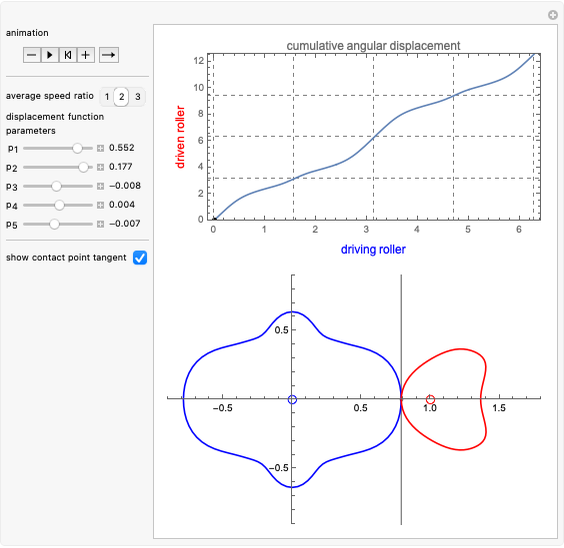
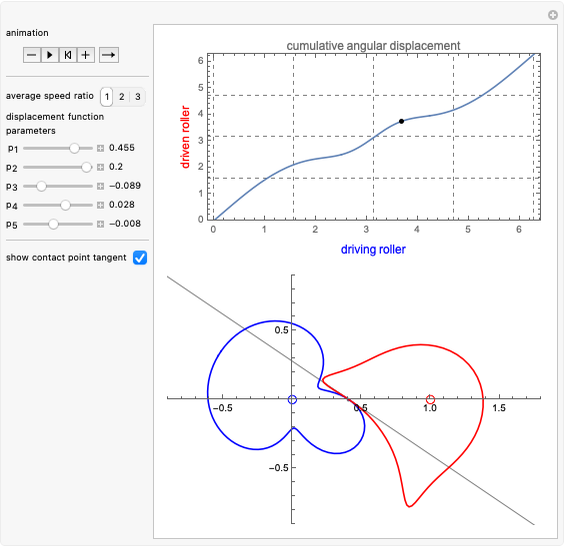
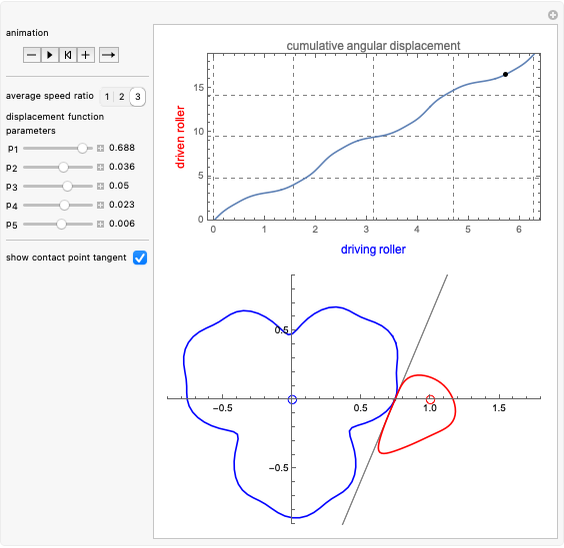
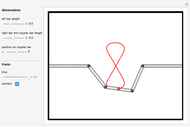
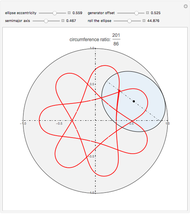
In this Demonstration, a roller drive is simulated by two curves rolling along each other without slipping. The curve on the left is the driving roller and turns at a constant angular speed. The curve on the right is the driven roller and turns at an angular speed proportional to the ratio between the radii of both rollers at their contact point.
[more]
Contributed by: Erik Mahieu (April 2013)
Open content licensed under CC BY-NC-SA
Details
For a good article about curves rolling along other curves, see [1].
Take the cumulative angular displacement of the driving roller to be  and that of the driven roller to be
and that of the driven roller to be 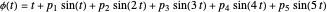 .
.
The radius at the mutual contact point of the driving roller is 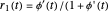 , and that of the driven roller is
, and that of the driven roller is 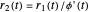 . These are effectively the polar equations of both curves.
. These are effectively the polar equations of both curves.
Reference
[1] J. Bloom and L. Whitt, "The Geometry of Rolling Curves," The American Mathematical Monthly, 88(6), 1981 pp. 420–426. http://www.jstor.org/discover/10.2307/2321826.
Snapshots
Permanent Citation