Adding Points on an Elliptic Curve

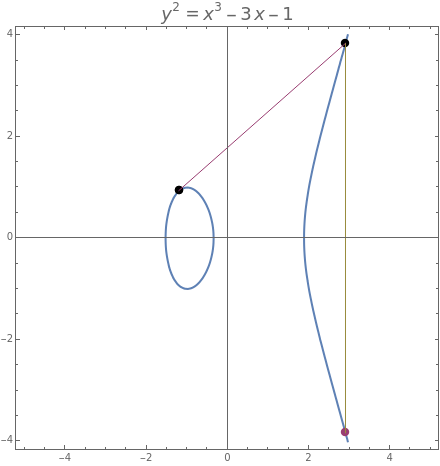
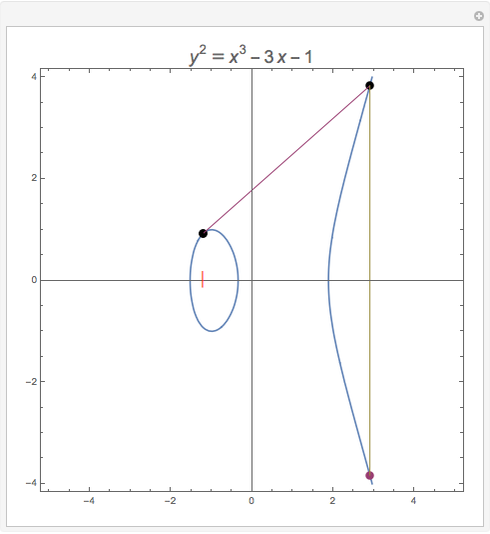
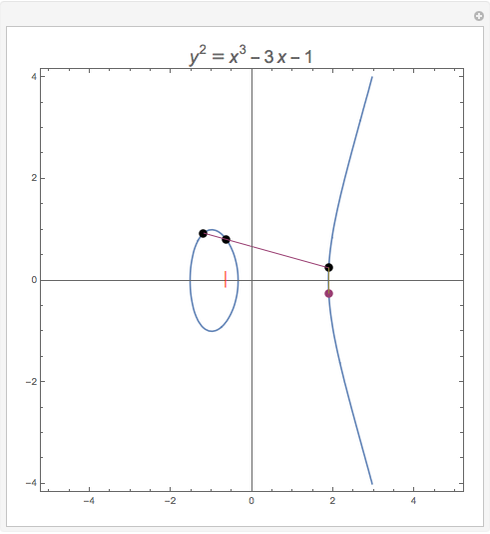
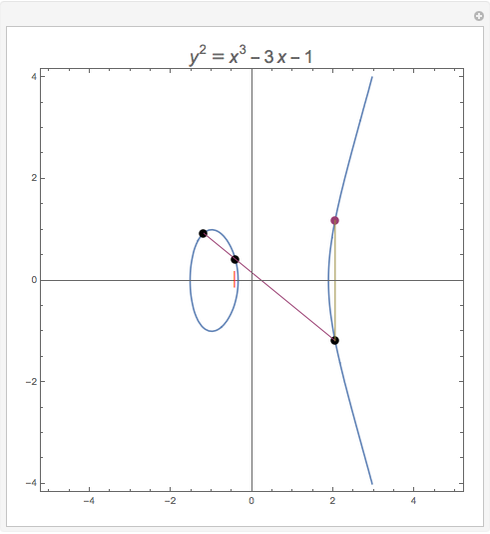
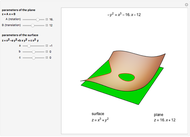
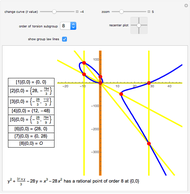
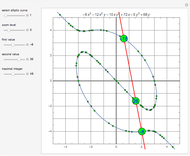
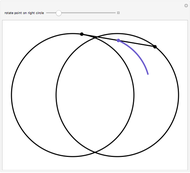
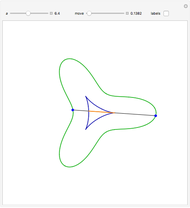
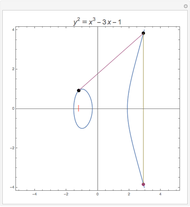
Elliptic curves are the solutions sets of nonsingular cubic polynomials of degree three. It is possible to define an addition law for these points so that they form an abelian algebraic group. In order to add distinct points, construct the line between them and determine the third point of intersection with the curve. The sum of the two points is then the reflection of the third point about the axis of symmetry, which is the axis for the case illustrated here. In order to add a point to itself, use the tangent line to the curve at that point.