Comparing Binomial Generalized Linear Models

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
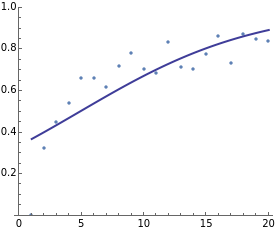
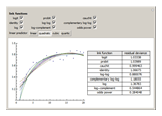
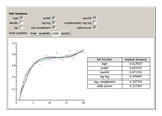
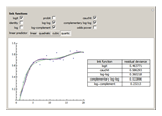
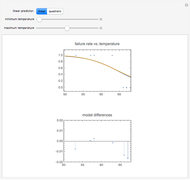
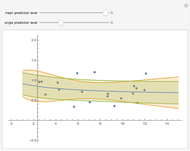
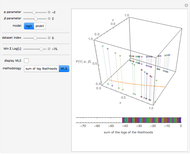
Generalized linear models are models of the form  , where
, where  is an invertible function called the link function and the
is an invertible function called the link function and the  are basis functions of one or more predictor variables. The term
are basis functions of one or more predictor variables. The term  is linear in the
is linear in the  and is referred to as the linear predictor. The value
and is referred to as the linear predictor. The value  is the predicted response for the
is the predicted response for the  observed response
observed response  , and the
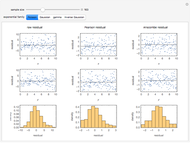
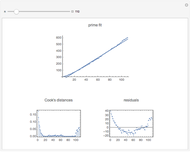
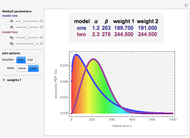
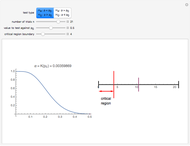
, and the  are assumed to be independent observations from the same exponential family of distributions. When the exponential family is the binomial family, the success probability
are assumed to be independent observations from the same exponential family of distributions. When the exponential family is the binomial family, the success probability  is modeled.
is modeled.
Contributed by: Darren Glosemeyer (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
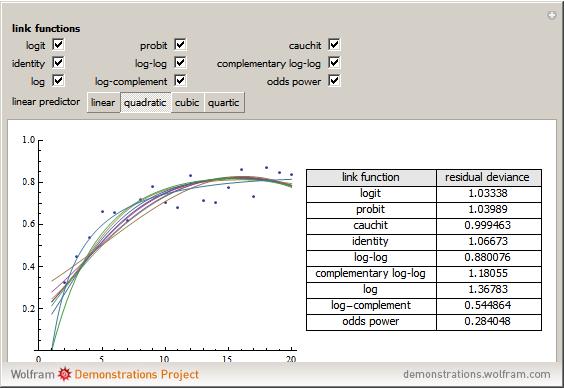
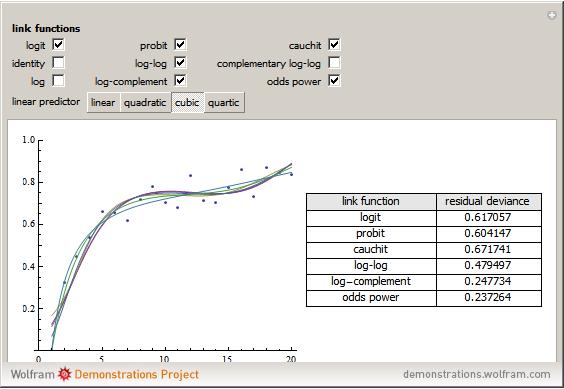
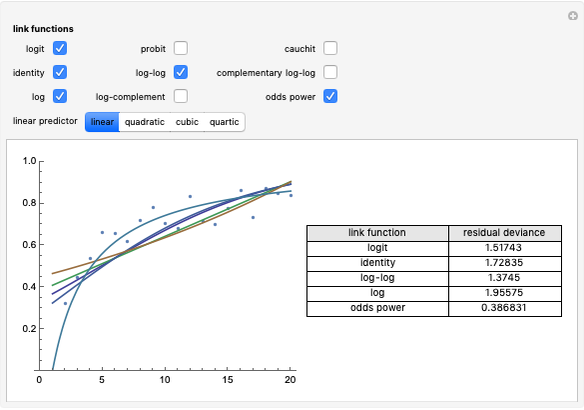
The logit link function is  .
.
The probit link is the inverse CDF for a standard normal distribution, and the cauchit link is the inverse CDF for a standard Cauchy distribution.
The identity function for  is
is  .
.
The log‐log and complementary log-log links are  and
and  , respectively.
, respectively.
The log and log complement links are  and
and  , respectively.
, respectively.
The odds power link is the odds power link  , with
, with  taken to be 1.
taken to be 1.
Permanent Citation