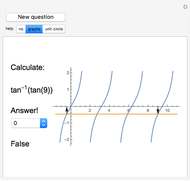
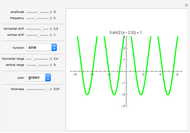
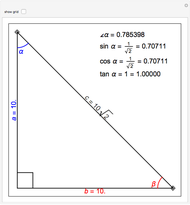
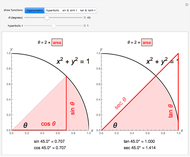
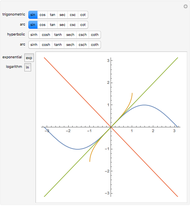
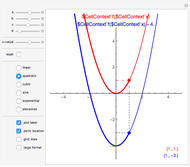
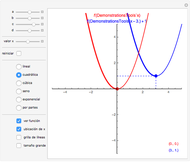
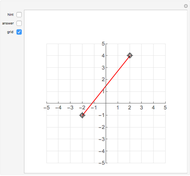
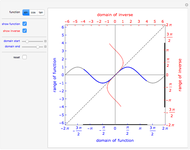
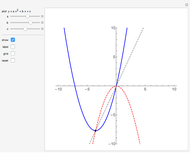
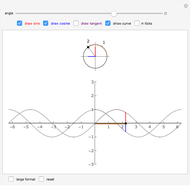
The sine, cosine, and tangent functions are not invertible on their natural domains. In order to define the arcsine, arccosine, and arctangent functions, the domains of the sine, cosine, and tangent functions must be restricted to intervals on which each of these functions are one-to-one and thereby invertible. The sliders allow you to restrict their domain while watching the corresponding inverse plots. This approach emphasizes that the inverse plots are functions when the original functions are one-to-one. The range of the inverse trigonometric functions arcsine, arccosine, and arctangent are shown corresponding to the restricted domains of the sine, cosine, and tangent.