Wolfram Demonstrations Project

12,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

Dynamics of Automobile Suspension Systems

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

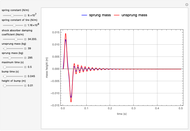
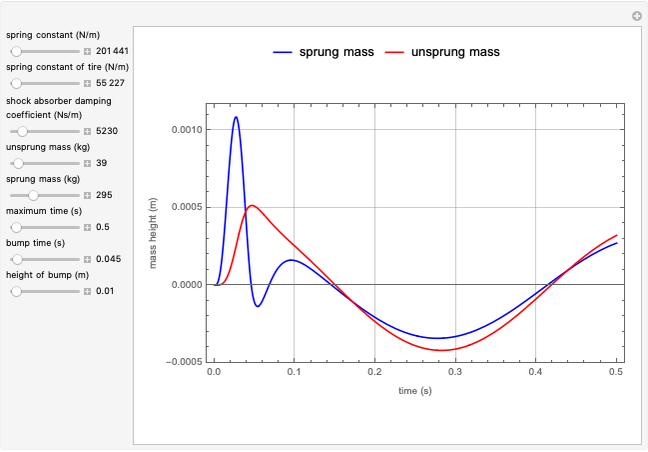
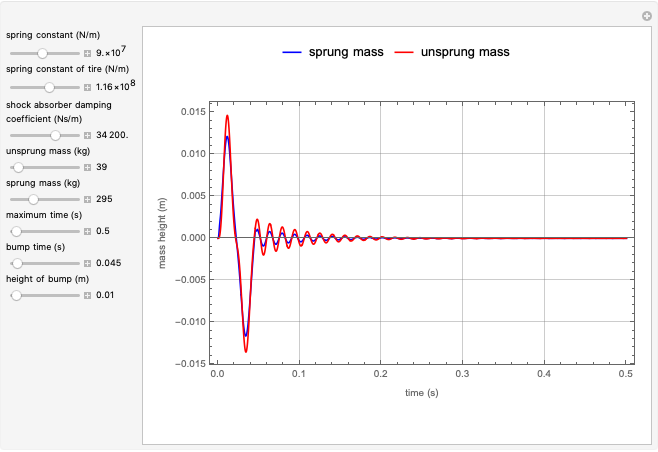
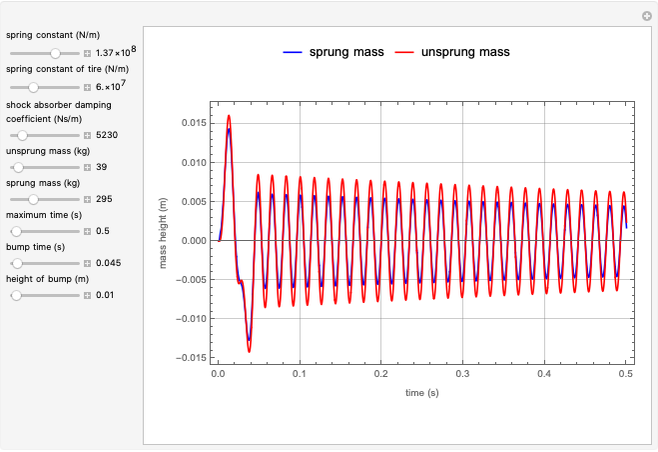
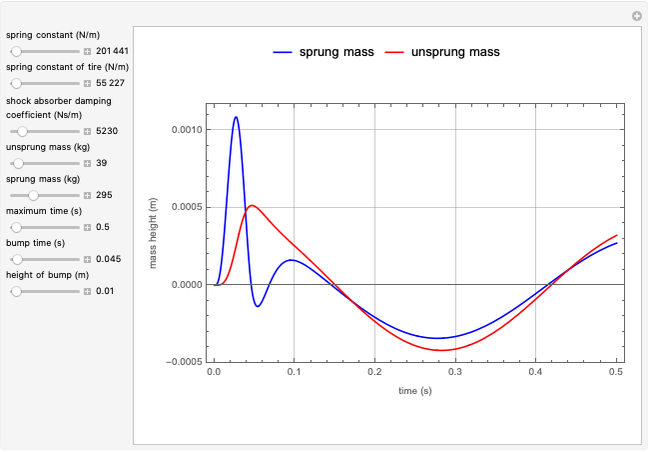
This Demonstration considers a quarter vehicle model comprising a tire, spring, damper and body mass. The plots represent the vertical displacements (m) of both the body mass and the unsprung mass (tire).

[more]

The system can be represented by two equations of motion:

and

.

As increases, the oscillating response increases in both magnitude and duration. Damping causes the system to eventually settle at 0. Specific parameters for a vehicle determine its response to various road conditions.

[less]

Contributed by: Amir Sweis and Joshua Steimel (August 2022)
Open content licensed under CC BY-NC-SA

Snapshots

Details

Snapshot 1: hard damping

Snapshot 2: weak dampening

Snapshot 3: long time behavior

Reference

[1] S. Lajqi and S. Pehan, "Designs and Optimizations of Active and Semi-active Nonlinear Suspension Systems for a Terrain Vehicle," Strojniški vestnik - Journal of Mechanical Engineering, 58(12), 2012 pp. 732–743. doi:10.5545/sv-jme.2012.776.

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send