Mathematica MathWorld Wolfram Science More »

HOME

TOPICS

LATEST

Fermat's Magic Cube

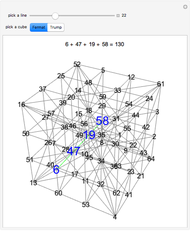
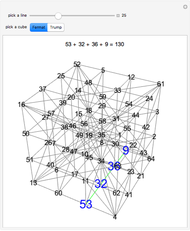
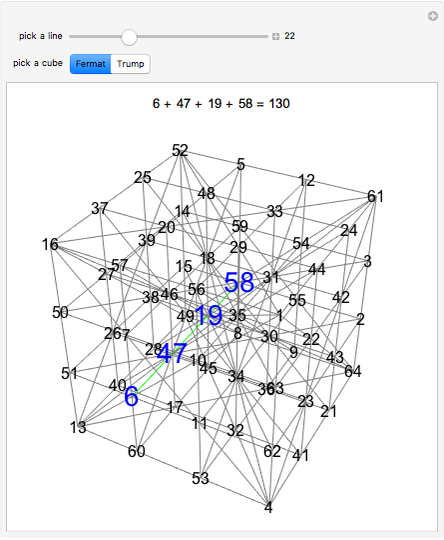
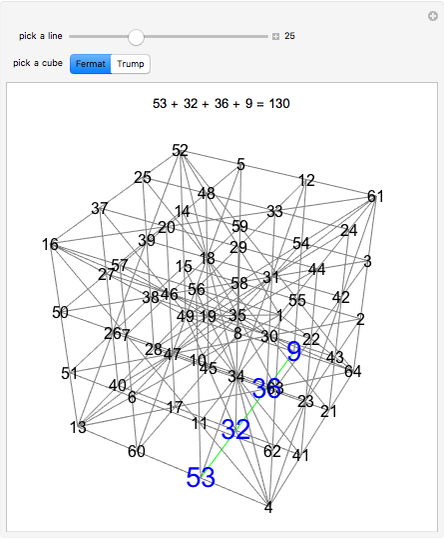
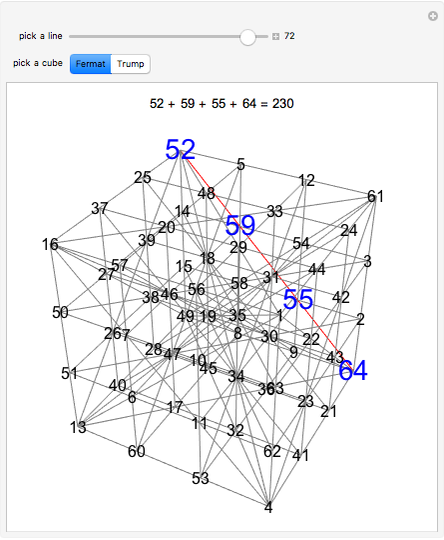
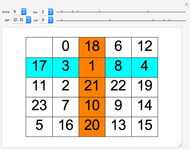
In 1640, Pierre de Fermat sent a letter to Marin Mersenne about an order-4 magic cube with 64 magic sums out of 76 possible. In a perfect magic cube the rows, columns, pillars, space diagonals, and the diagonals of each n×n orthogonal slice sum to the same number. For the order-4 case, numbers 1 to 64 are required. In 1972, Richard Schroeppel proved that a perfect order-4 magic cube was impossible (see Details).

In 2004, Walter Trump found an order-4 cube with eight more lines that summed to 130.

Contributed by: Ed Pegg Jr

Show Initialization Code

(16 lines omitted)

Lemma 1: In a magic square of order 4, the sum of the corners is

Proof: Add together each edge of the square and the two diagonals. This covers the square entirely, and each corner twice again. This adds to

, so twice the corner sum is

Lemma 2: In a magic cube of order 4, the sum of any two corners connected by an edge of the cube is

Proof: Call the corners

and

. Let

, and

be the corners of any two edges of the cube parallel to

. Then

, and

are all the corners of magic squares. So

;

Let

(= 130) be the sum of a row. Consider a corner

. There are three corners connected by an edge to

. Each must have the same value

, contradicting the requirement that all values are different. Thus, there is no magic cube of order 4.

QED. (Proof by Richard Schroeppel, 1972)

Perfect Magic Cube (Wolfram MathWorld)

"Fermat's Magic Cube" from The Wolfram Demonstrations Project
http://demonstrations.wolfram.com/FermatsMagicCube/

Contributed by: Ed Pegg Jr

Report an issue »

Interact with Demonstrations using the latest version of the free Mathematica Player—Download Now

Browse all topics

	Source page:
	Email	Name (optional)
	Occupation (optional)	Organization (optional)
	I use Mathematica often occasionally never
	Country (optional) Remember me
	Note: Please do not include anything you consider confidential or proprietary. Your message and contact information may be shared with the author of any specific Demonstration for which you give feedback, but will not otherwise be published or distributed. Privacy Policy »

Wolfram Research

Site Index

RSS

Atom