GL(3,n) Acting on 3D Points
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
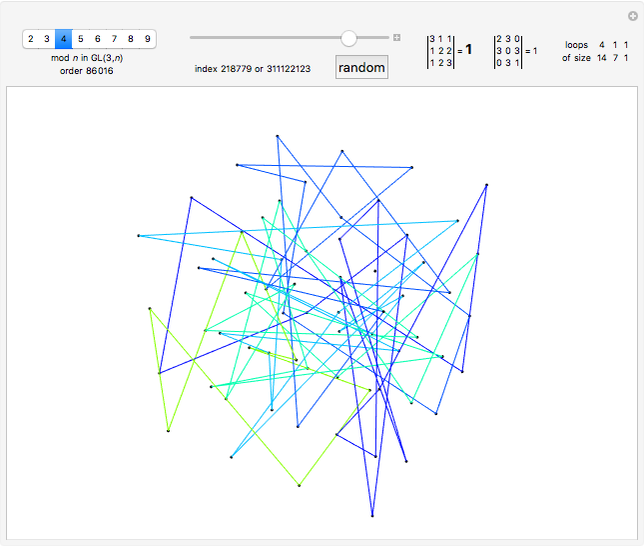
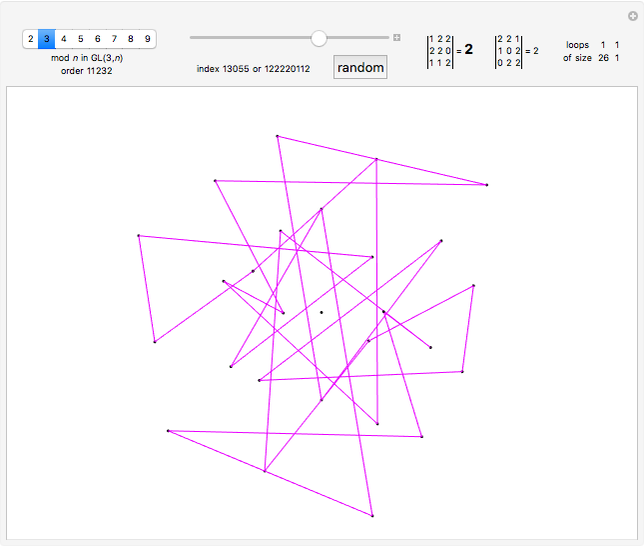
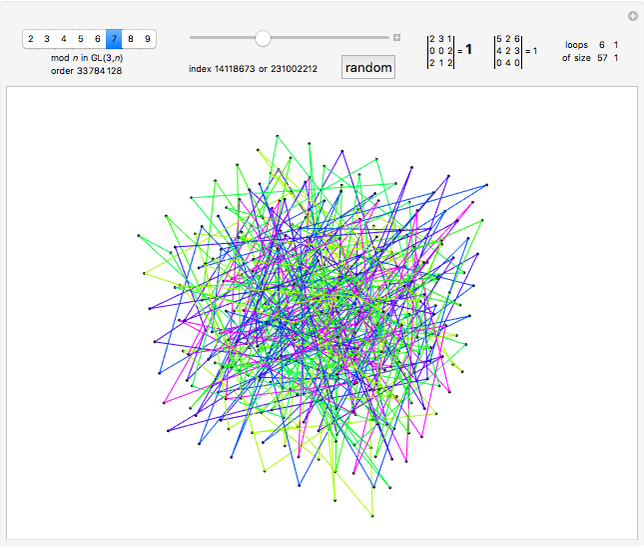
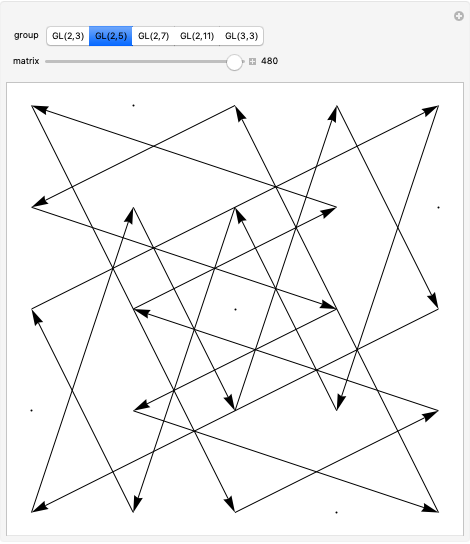
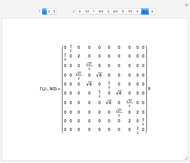
The three-dimensional space  contains 125 points:
contains 125 points:  ,
,  , … ,
, … , 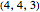 ,
,  . The 1,488,000 invertible 3×3 matrices over
. The 1,488,000 invertible 3×3 matrices over  form the general linear group known as
form the general linear group known as  . They act on
. They act on  by matrix multiplication modulo 5, permuting the 125 points.
by matrix multiplication modulo 5, permuting the 125 points.
Contributed by: Ed Pegg Jr (December 2017)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Permanent Citation