Measuring Flow Rates with a Pitot Tube

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
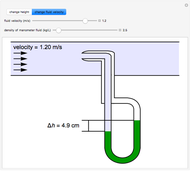
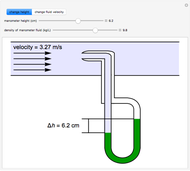
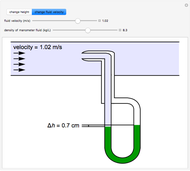
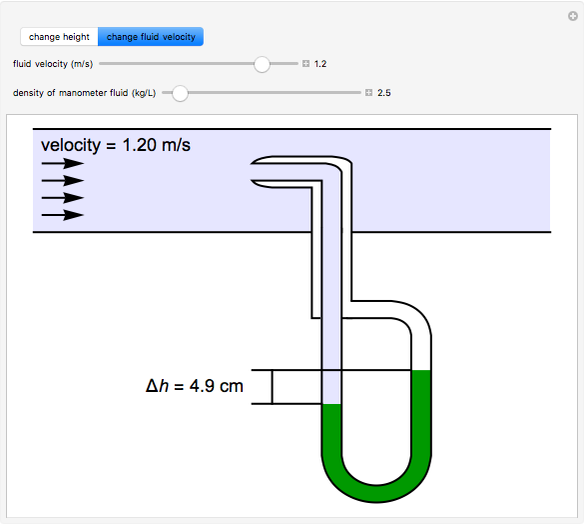
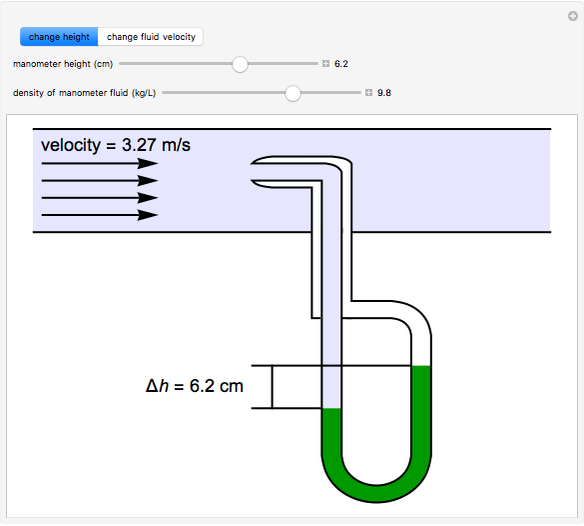
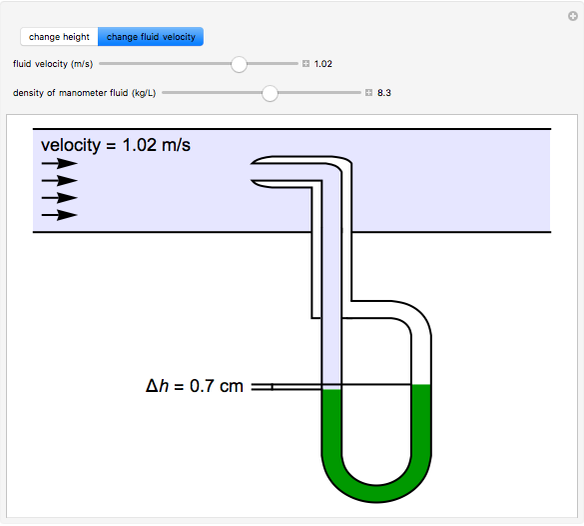
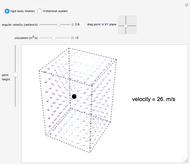
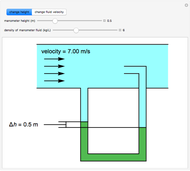
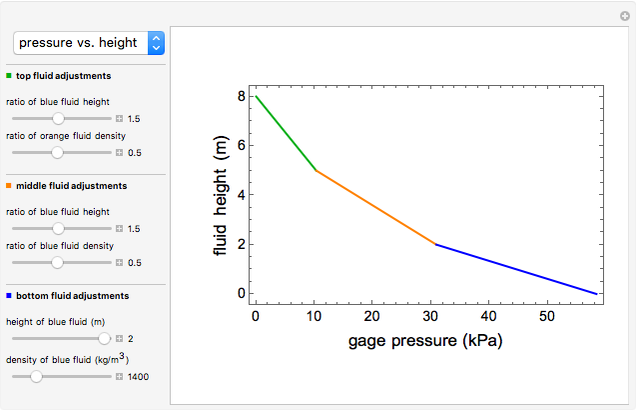
A pitot tube determines the velocity of a fluid by measuring the fluid's stagnation pressure. In this demonstration, stagnation pressure is measured with a manometer. The height differential in the manometer is a function of the manometer fluid density, and the flowing fluid's kinetic energy. Bernoulli's equation relates these terms, and is used to solve for velocity. Vary the manometer fluid (green) height or the velocity of the fluid in the pipe (blue) to see how each variable is related. Also, use a slider to vary the density of the fluid in the manometer.
Contributed by: Jon Barbieri and Rachael L. Baumann (September 2014)
Additional contributions by: Garret D. Nicodemus and Neil Hendren
(University of Colorado Boulder, Department of Chemical and Biological Engineering)
Open content licensed under CC BY-NC-SA
Details
Pitot tubes are used to measure the velocity of a fluid moving through a pipe by taking advantage of the fact that the velocity at the height of the bend in the tube (stagnation point) is zero. Some kinetic energy density of the fluid flowing through the pipe is converted into pressure, resulting in a change in manometer height. Bernoulli's equation is used to calculate the velocity of the bulk fluid in the pipe by using this pressure difference in the pitot tube:
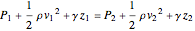 .
.
All terms on the left side represent the stagnation point (entrance of the pitot tube); here 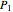 is the stagnation pressure and
is the stagnation pressure and  is the velocity of fluid in the pipe at point 1. All terms on the right side refer to point 2, a point upstream from the pitot tube. The two points that are being evaluated are at the same height, so
is the velocity of fluid in the pipe at point 1. All terms on the right side refer to point 2, a point upstream from the pitot tube. The two points that are being evaluated are at the same height, so 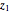 and
and 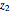 drop out. Thus we obtain the simplified form of Bernoulli's equation:
drop out. Thus we obtain the simplified form of Bernoulli's equation:
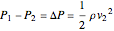 .
.
The equation for the difference in pressure in a manometer is substituted into the simplified Bernoulli equation:
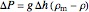 ,
,
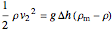 .
.
This equation can be rearranged and used to solve for fluid velocity or difference in height of the fluids in the manometer:
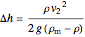 ,
,
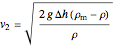 .
.
Where 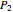 is the static pressure of fluid in the pipe,
is the static pressure of fluid in the pipe,  and
and 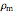 are the densities of the fluid in the pipe and manometer fluid,
are the densities of the fluid in the pipe and manometer fluid,  is specific gravity of fluid in the pipe,
is specific gravity of fluid in the pipe,  is the gravitational constant, and
is the gravitational constant, and 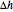 is the difference in height of the manometer fluid.
is the difference in height of the manometer fluid.
Snapshots
Permanent Citation