Details

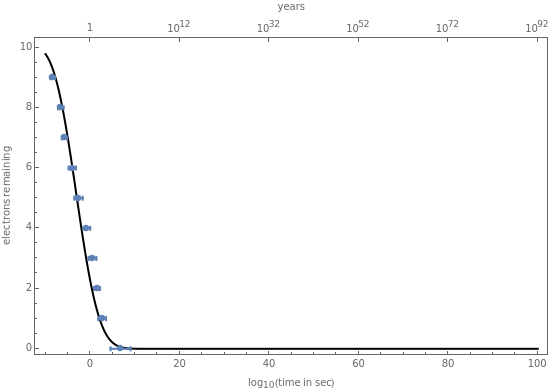
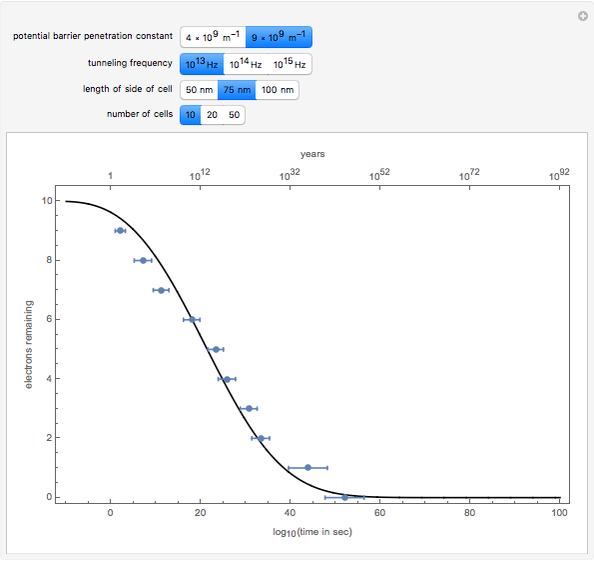
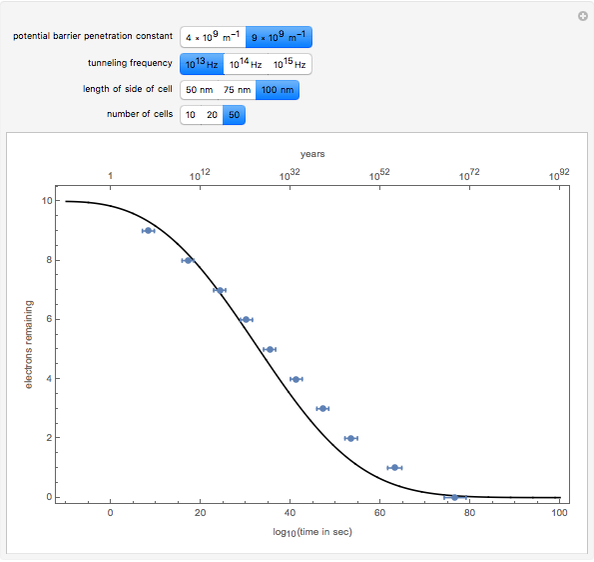
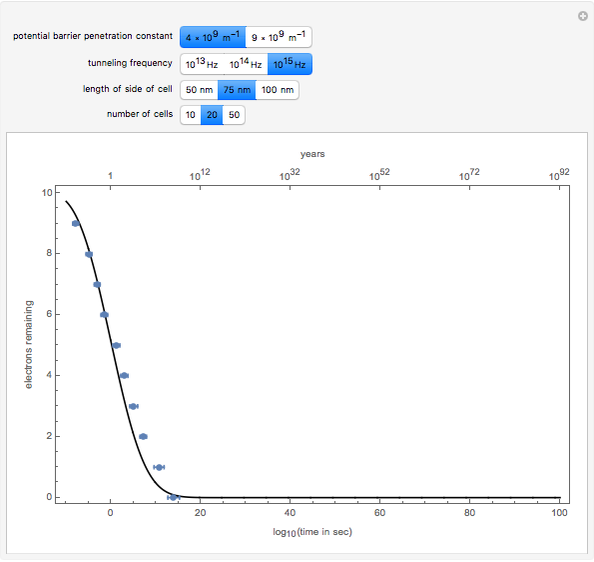
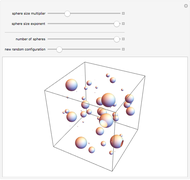
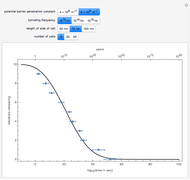
Use the buttons to set values for the potential barrier constant , the tunneling frequency , the size of the cubic cell and the number of cells.

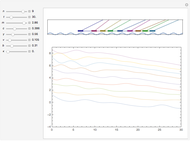
As the tunneling frequency increases or as the length of the side of the cube decreases, the charge recombination in the crystal increases. The process depends very strongly on the potential barrier penetration constant . The solid line in the graph represents an analytical solution [1]. For more details of the simulation, see [2].

Function Details

Pairing produces a list of times (in seconds) that it takes for each electron-hole pair to recombine. The arguments for Pairing include the list of initial locations of the electrons (epos0) and holes (hpos0), the tunneling frequency in Hz (stun) and the potential barrier penetration constant in (α).

ChargeLossSimulation performs the Monte Carlo simulation of the recombination of charge due to ground-state tunneling. The output of ChargeLossSimulation is the number of charge pairs in the cell as a function of time. In addition, ChargeLossSimulation computes the analytical equation for the number of pairs remaining as a function of time. The arguments for ChargeLossSimulation include the number of cells used in the simulation (nsolids), the tunneling frequency in Hz (stun), the potential barrier penetration constant in (α) and the length of the cell side in nanometers (sidelengthnm).

References

[1] G. Kitis and V. Pagonis, "Analytical Solutions for Stimulated Luminescence Emission from Tunneling Recombination in Random Distributions of Defects," Journal of Luminescence, 137, 2013 pp. 109–115. doi:10.1016/j.jlumin.2012.12.042.

[2] V. Pagonis and C. Kulp, "Monte Carlo Simulations of Tunneling Phenomena and Nearest Neighbor Hopping Mechanism in Feldspars," Journal of Luminesence, 181, 2017 pp. 114–120. doi:10.1016/j.jlumin.2016.09.014.