Normal Lines to a Parabola

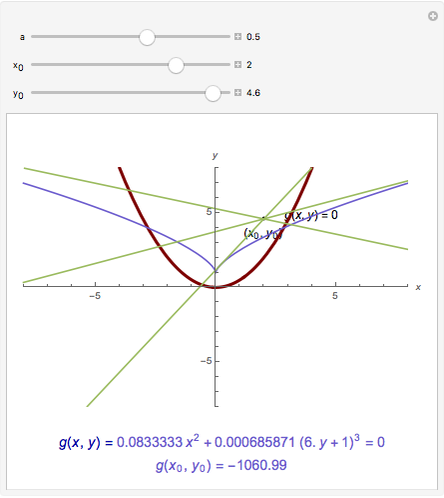
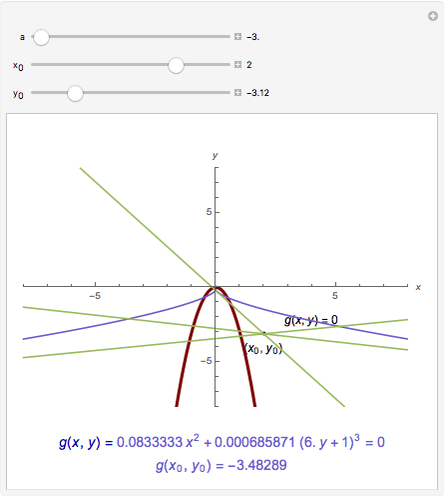
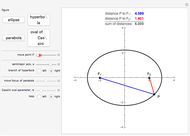
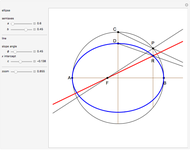
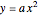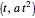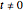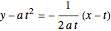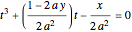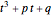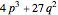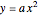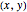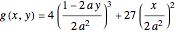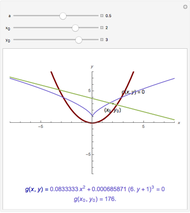
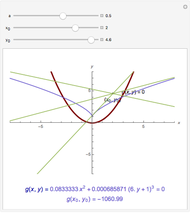
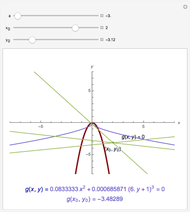
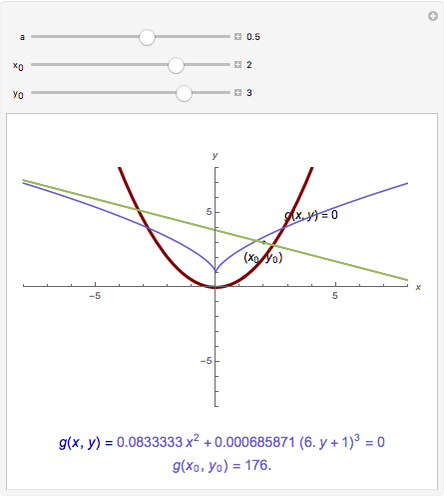
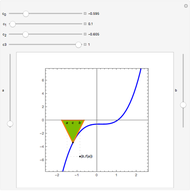
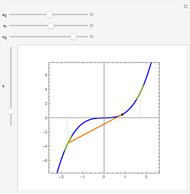
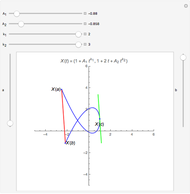
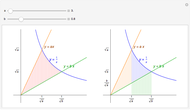
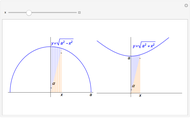
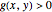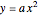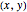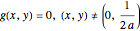The normal line to the graph of parabola at the point , is , or equivalently . The number of real roots of a reduced cubic depends on the sign of . So the number of normal lines to through depends on the sign of . If , there is one normal line to through ; if , there are two normal lines; and if 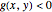, there are three. (For the cusp 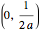, the only normal line is .)