Pentagonal Pyramid

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
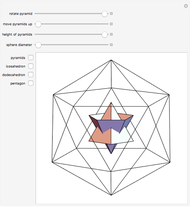
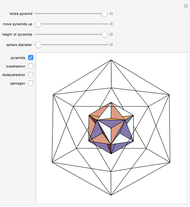
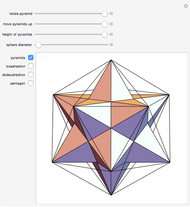
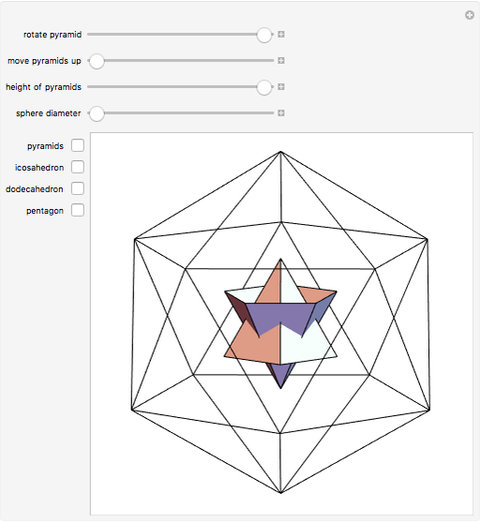
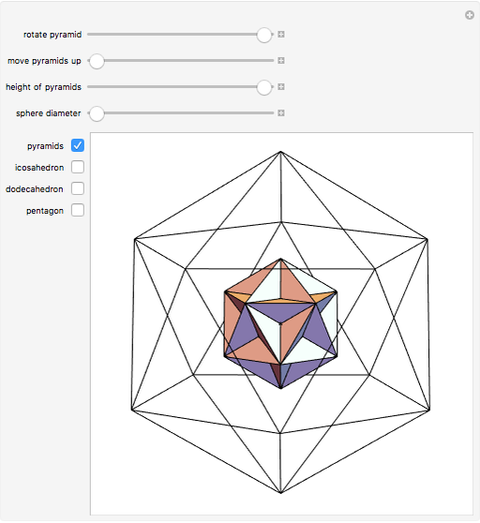
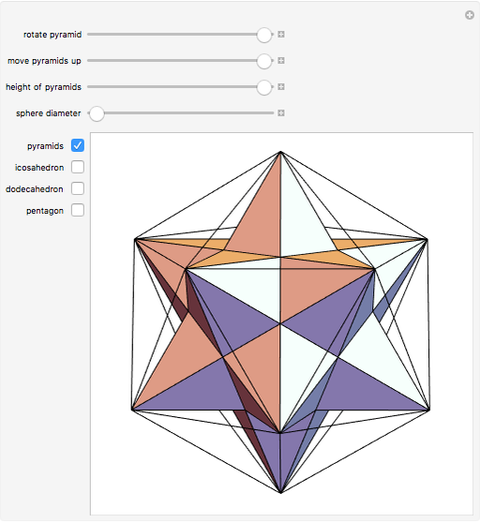
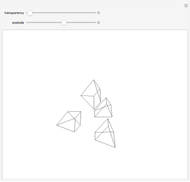
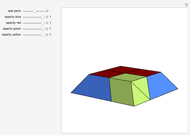
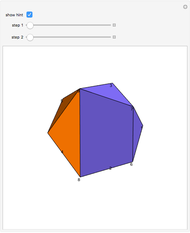
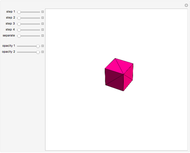
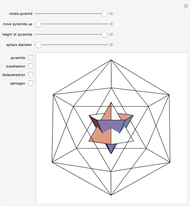
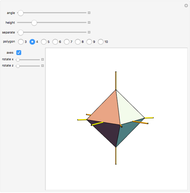
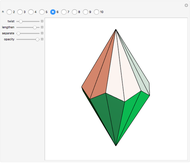
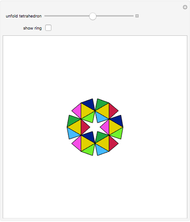
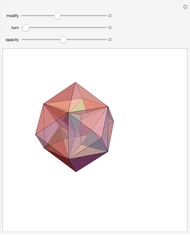
A pentagonal pyramid, having edge lengths 1 and &straightpi; (the golden ratio), can be used to study the relationships of various polyhedra. Two such pyramids, sharing the same axis but pointing in opposite directions, determine the vertices of an icosahedron. If such a pyramid is placed at the vertices of the icosahedron, then a great dodecahedron is obtained. A small stellated dodecahedron can be produced if the pyramids are moved radially until the pentagon edges meet. Further polyhedra can be made by varying the height of the pyramids.
Contributed by: Sándor Kabai and Gábor Gévay (May 2008)
Open content licensed under CC BY-NC-SA
Snapshots
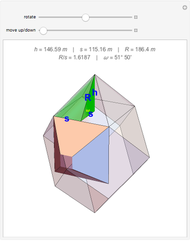
Details
detailSectionParagraphPermanent Citation
"Pentagonal Pyramid"
http://demonstrations.wolfram.com/PentagonalPyramid/
Wolfram Demonstrations Project
Published: May 13 2008