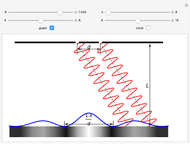
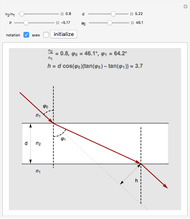
Rainbows of Different Order in Water Droplets and Glass Beads

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
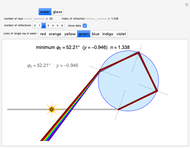
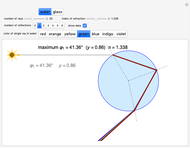
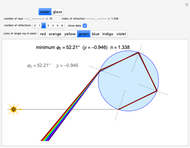
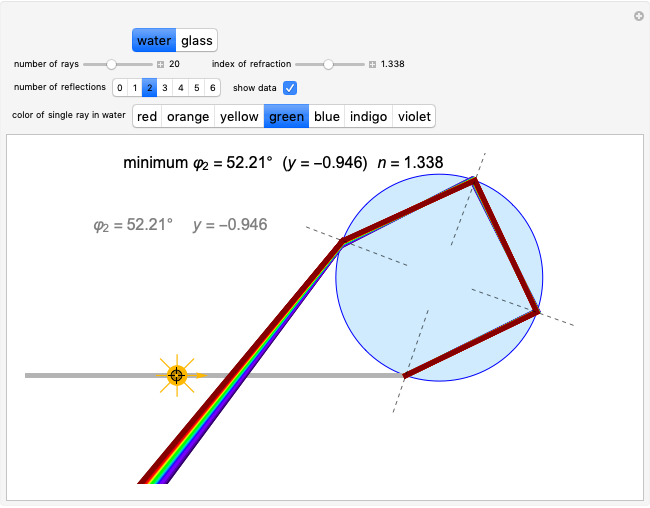
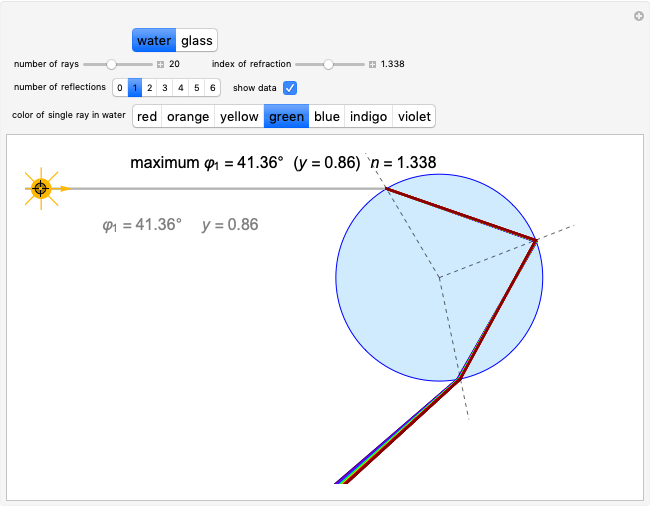
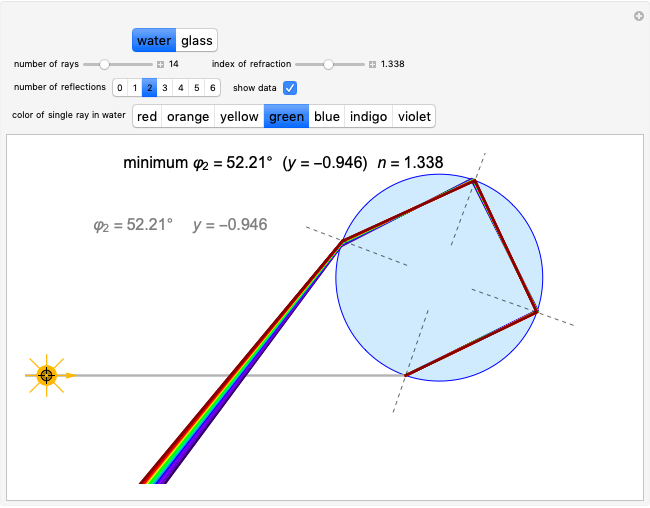
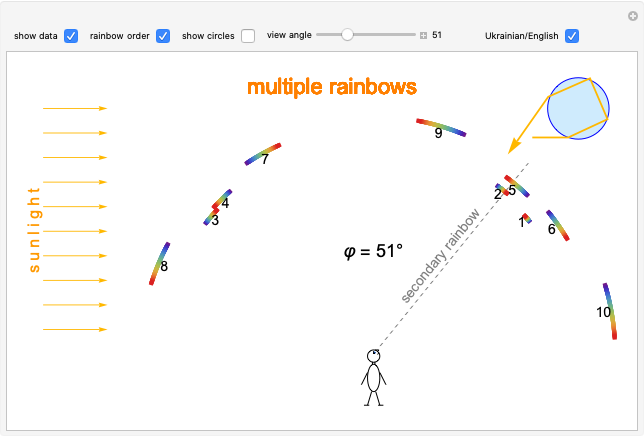
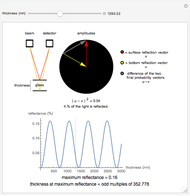
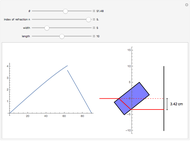
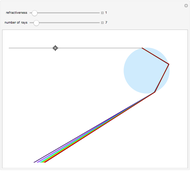
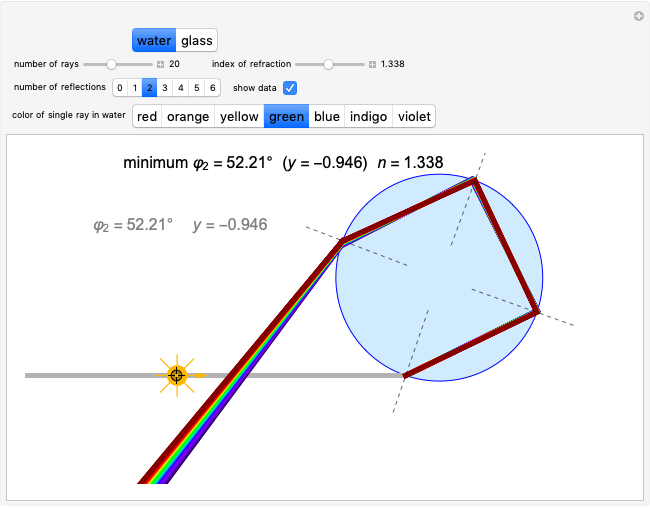
A single reflection of sunlight inside a falling raindrop produces the primary rainbow. Double reflection gives rise to the secondary rainbow. This Demonstration calculates the parameters for rainbows of the first six orders. You can follow the rays as they are refracted and reflected in water droplets and glass beads to form rainbows of different order. You can move the relative position of the white light source using the locator.
Contributed by: Volodymyr Holovatsky and Elizabeth Orynchuk (August 2022)
(Yuriy Fedkovych Chernivtsi National University, Ukraine, and Vyzhnytsia Gymnasium, Ukraine)
Open content licensed under CC BY-NC-SA
Snapshots
Details
 = impact parameter
= impact parameter
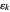 = angle for the
= angle for the 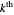 -order rainbow
-order rainbow
refraction indices for water:
red 1.3312
orange 1.3335
yellow 1.3342
green 1.3380
blue 1.3400
indigo 1.3415
violet 1.3435
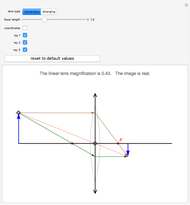
Snapshot 1: the primary rainbow
Snapshot 2: the secondary rainbow
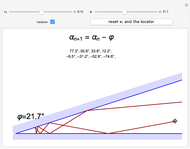
Snapshot 3: violet ray with three reflections
Snapshot 4: secondary rainbow for glass sphere
Snapshot 5: rays after two reflections in a water drop; no rainbow because intensity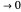 when
when 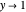
References
[1] J. D. Walker, "Multiple Rainbows from Single Drops of Water and Other Liquids," American Journal of Physics, 44(5), 1976 pp. 421–433. doi:10.1119/1.10172.
[2] R. L. Lee and P. Laven, "Visibility of Natural Tertiary Rainbows," Applied Optics, 50(28), 2011 pp. F152–F161. doi:10.1364/AO.50.00F152.
[3] M. Großmann, E. Schmidt and A. Haußmann, "Photographic Evidence for the Third-Order Rainbow," Applied Optics, 50(28), 2011 pp. F134–F141. doi:10.1364/AO.50.00F134.
[4] M. Theusner, "Photographic Observation of a Natural Fourth-Order Rainbow," Applied Optics, 50(28), 2011 pp. F129–F133. doi:10.1364/AO.50.00F129.
[5] H. E. Edens, "Photographic Observation of a Natural Fifth-Order Rainbow," Applied Optics, 54(4), 2015 pp. B26–B34. doi:10.1364/AO.54.000B26.
[6] D. T. Ivanov and S. N. Nikolov, "A New Way to Demonstrate the Rainbow," The Physics Teacher, 54(8), 2016 pp. 460–463. doi:10.1119/1.4965263.
[7] M. Welter, "Another Rainbow Demonstration with a Glass Sphere," The Physics Teacher, 57(5), 2019 p. 344. doi:10.1119/1.5098931.
Permanent Citation