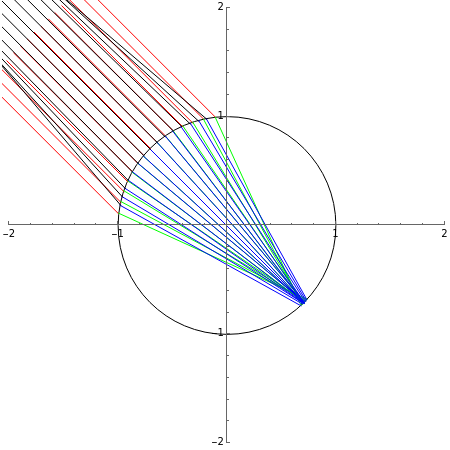
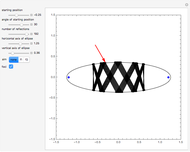
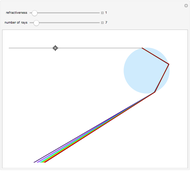
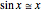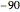Retroreflection is the ability of a material to reflect a beam of light back in the opposite direction, over a large range of incoming directions. Corner cubes and corner prisms achieve this, with applications in communication, surveying, and for other types of devices. It turns out that a transparent sphere is also retroreflective (with certain advantages over corner cubes, such as wider acceptance angles). This Demonstration provides insight as to why a transparent sphere is a good retroreflector, specifically by showing its interior ray geometry.
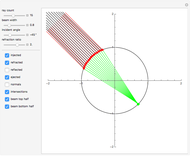
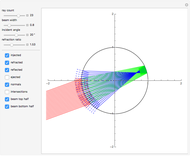
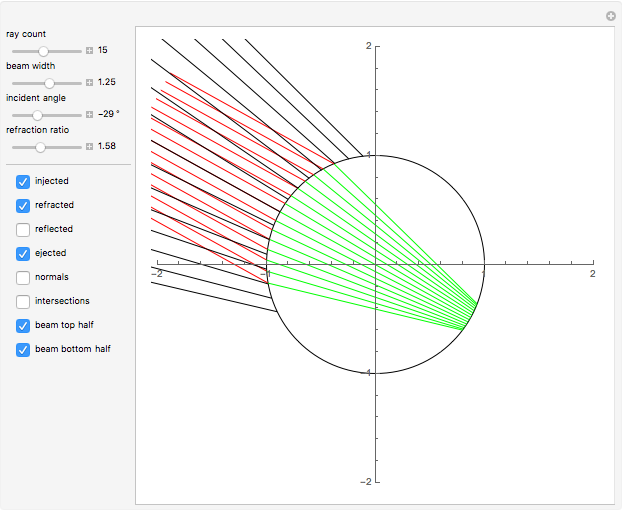
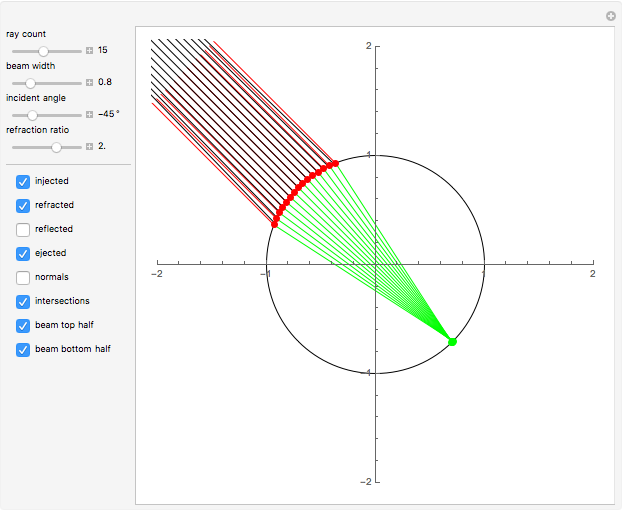
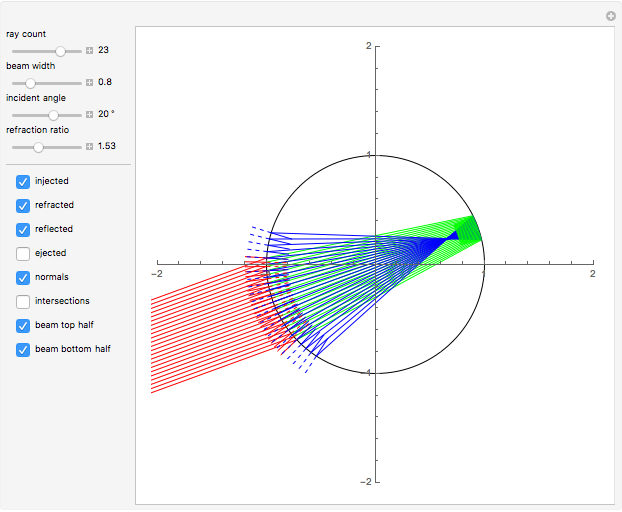
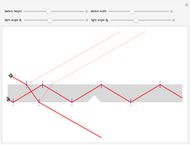
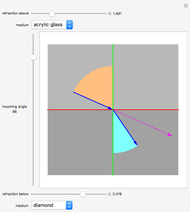
The graphic shows a circular cross section through the center of a unit sphere and an incoming beam of parallel rays (drawn in red) whose incident angle, number of rays, and width can be set with the controls. As the incoming rays enter the sphere they refract according to Snell's law; the resulting refracted rays are drawn in green. The index of refraction for the sphere and the environment leads to the refraction ratio. The higher this ratio, the more the incoming rays' directions are disrupted; in the case of a sphere, the more the refracted beam will converge. For example, if the sphere is made of glass and the environment is air, the refraction ratio is approximately 1.5. For a diamond, the ratio is approximately 2.41 (though very expensive for a retroreflector).
When the refracted rays hit the back of the sphere (presumed reflective), they reflect back toward the front. Reflected rays are drawn in blue. To achieve good reflection there, that part of the sphere could be mounted onto a reflective socket. Finally, the reflected rays refract from the sphere out to the environment, giving rise to the outgoing beam (drawn in black). Good retroreflection occurs if the outgoing rays (black) are roughly parallel to the incoming ones (red), over a wide range of incoming angles.
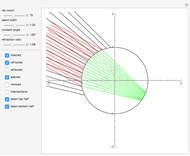
Changing the refraction ratio lets one quickly realize that good retroreflection occurs at a ratio around 1.9, for which the refracted beam focuses almost perfectly on the back of the sphere. Because reflection is symmetric, the order of reflected rays (blue) is reversed with respect to the refracted rays (green). If the beam width is not too large (with respect to the sphere's radius), then Snell's law is nearly linear (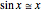 for small
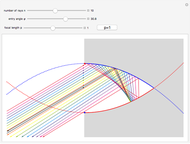
for small  ) and inward refraction is roughly the inverse of outward refraction. Choosing a larger beam width lets one visualize the effect of nonlinearity and how it decreases the device's performance by exacerbating spherical aberrations (a perfect focus is no longer possible, nor is a perfect refraction inversion).
) and inward refraction is roughly the inverse of outward refraction. Choosing a larger beam width lets one visualize the effect of nonlinearity and how it decreases the device's performance by exacerbating spherical aberrations (a perfect focus is no longer possible, nor is a perfect refraction inversion).
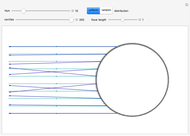
Most glasses have a refraction ratio of about 1.5; since the refractive index of air is around 1, setting the ratio to approximately 1.5 shows a glass sphere does not achieve sufficient convergence of the refracted beam (green) even when the beam width is small; if one desires to build a retroreflector out of glass, the solution (to be modeled in an upcoming Demonstration) is to use a compound device, comprising a unit radius front hemisphere glued back-to-back to a larger radius back hemisphere; such a device places the converging beam at a focal point coincident with the interior of the back hemisphere.
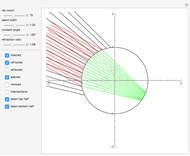
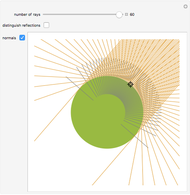
Checkboxes let you show or hide incoming, refracted, reflected, and outgoing beams, so you can more clearly visualize each effect separately. Checkboxes are also provided that reveal ray-sphere intersections as well as normals at every intersection point; the angle between rays and normals is used to compute both refractions and reflections.
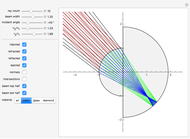
Finally, checkboxes are provided to show or hide the top or bottom halves of the incoming beam; in particular, by unchecking the "bottom beam" checkbox, setting the incoming angle to be 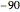 degrees and setting the refraction ratio to be about 1.33 (the index of refraction of water and ice), one can gain insight into the ray refraction/reflection geometry behind the formation of rainbows, as water droplets suspended in the air are roughly spherical.
degrees and setting the refraction ratio to be about 1.33 (the index of refraction of water and ice), one can gain insight into the ray refraction/reflection geometry behind the formation of rainbows, as water droplets suspended in the air are roughly spherical.
[less]