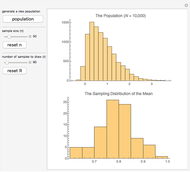
Sampling Distribution of a Positively Skewed Population

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
This Demonstration is meant to help students understand how, based on the central limit theorem, the sampling distribution of a skewed population distribution will become normally distributed.
Contributed by: Scott R. Colwell (June 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
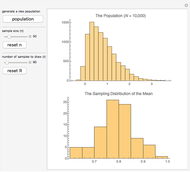
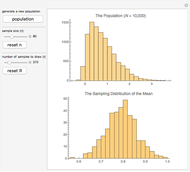
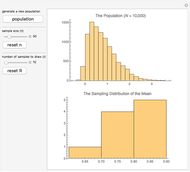
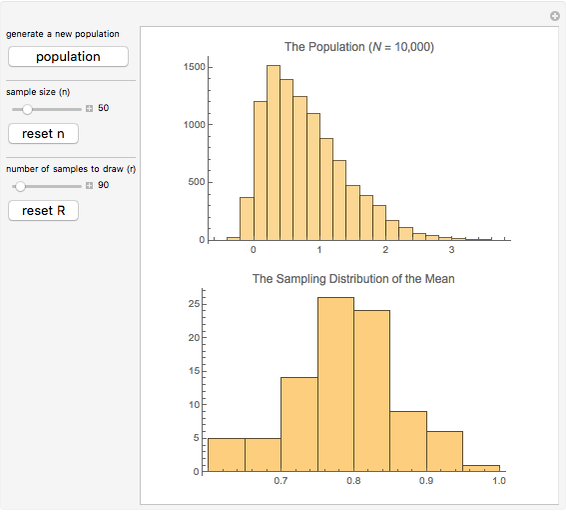
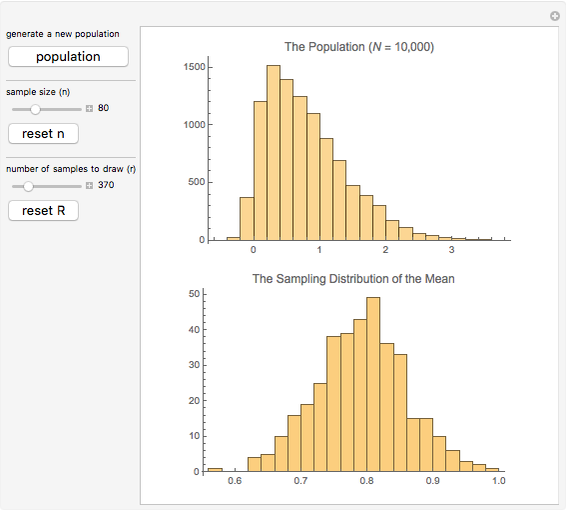
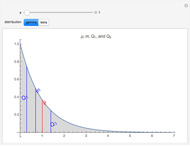
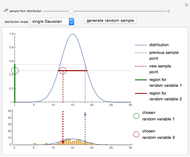
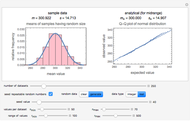
A population of the size 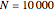 that is positively skewed is randomly generated when you click the "population" button. You can then change the "sample size",
that is positively skewed is randomly generated when you click the "population" button. You can then change the "sample size",  . This sets the size of a single sample that will be drawn from the population. You can then change the number of samples,
. This sets the size of a single sample that will be drawn from the population. You can then change the number of samples,  . This sets the number of samples that will be drawn (of size
. This sets the number of samples that will be drawn (of size  ) from the population. For example, setting
) from the population. For example, setting  to 10 and
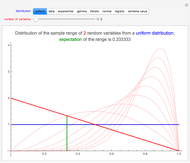
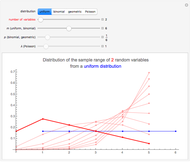
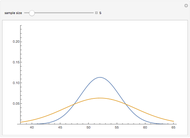
to 10 and  to 50 means that you are drawing a sample of 10, 50 times. The mean for each sample is then calculated (e.g. 50 means) and plotted on the histogram, which represents the sampling distribution of the means. As per central limit theorem, as the sample size (the number of means, i.e. the number of samples) increases, the sampling distribution of the means will become more normally distributed even though the population distribution is skewed.
to 50 means that you are drawing a sample of 10, 50 times. The mean for each sample is then calculated (e.g. 50 means) and plotted on the histogram, which represents the sampling distribution of the means. As per central limit theorem, as the sample size (the number of means, i.e. the number of samples) increases, the sampling distribution of the means will become more normally distributed even though the population distribution is skewed.
Permanent Citation
"Sampling Distribution of a Positively Skewed Population"
http://demonstrations.wolfram.com/SamplingDistributionOfAPositivelySkewedPopulation/
Wolfram Demonstrations Project
Published: June 24 2011