Two-Point Taylor Expansion

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
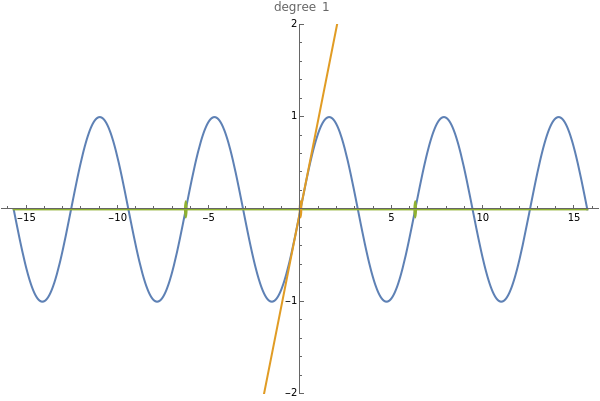
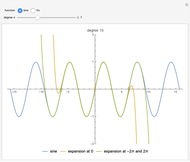
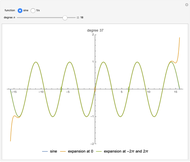
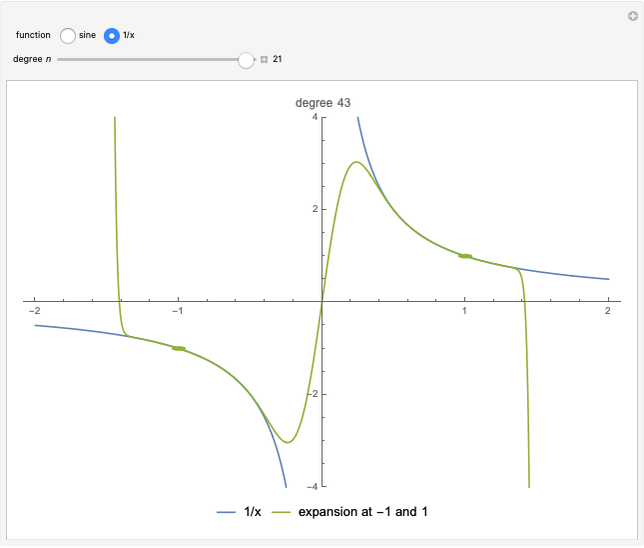
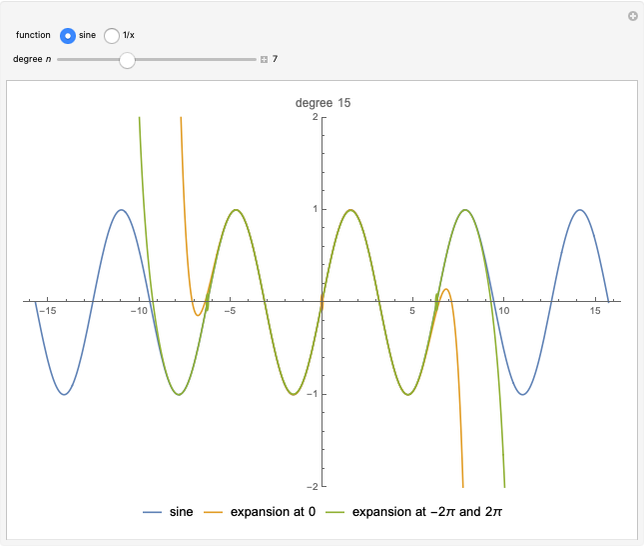
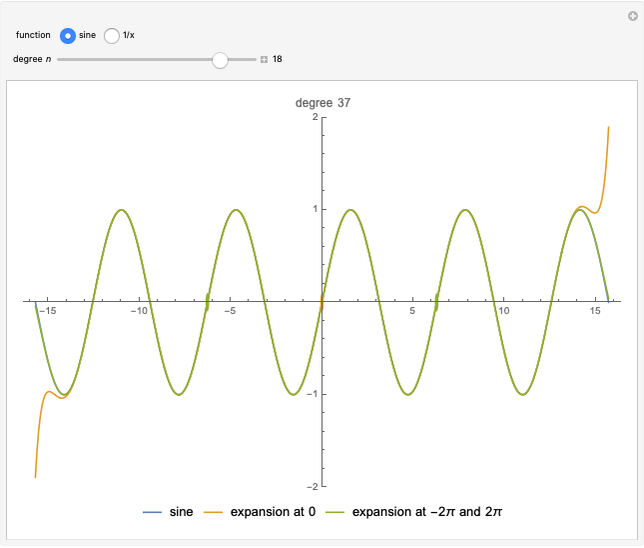
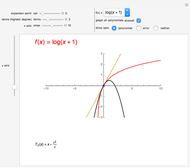
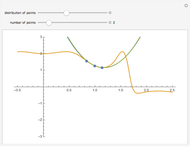
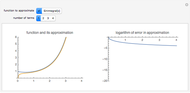
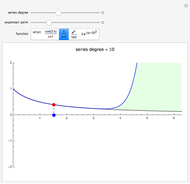
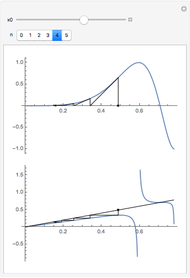
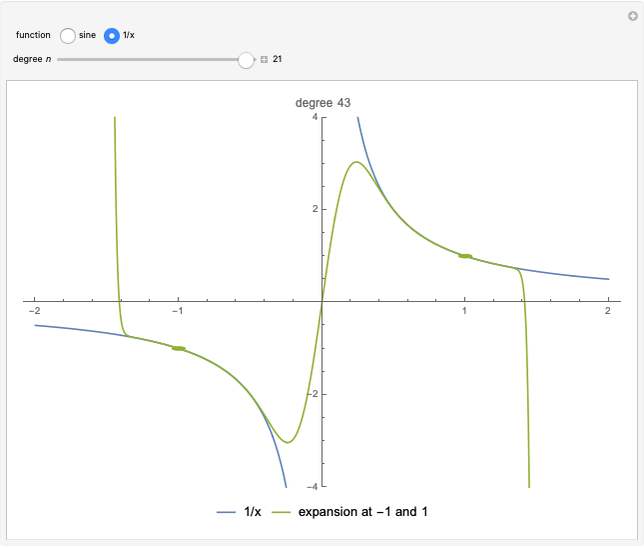
This Demonstration calculates recursively the two-point Taylor expansion (2pTE) of a function, which is a polynomial expansion around two reference points. This expansion is increasingly accurate as the number of steps  in the recursion progresses. This block shows two examples of the 2pTE applied to two different functions as the degree of the fitting polynomial increases: One is the expansion of the sine function at the points
in the recursion progresses. This block shows two examples of the 2pTE applied to two different functions as the degree of the fitting polynomial increases: One is the expansion of the sine function at the points  and
and  , while the standard one-point Taylor expansion around
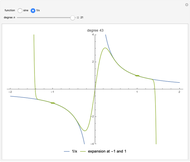
, while the standard one-point Taylor expansion around  is shown for comparison. The second example is the expansion of the reciprocal function
is shown for comparison. The second example is the expansion of the reciprocal function  at the points
at the points  and
and  .
.
Contributed by: Roland Hablützel (June 2020)
Open content licensed under CC BY-NC-SA
Snapshots
Details
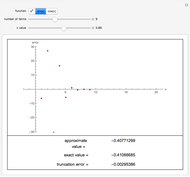
The two-point Taylor expansion can be used to provide an approximation of a function at two different points simultaneously. This two-point expansion generates a polynomial of degree  that is accurate to
that is accurate to  order at each of the two given reference points. The expansion can be computed even when the function being approximated is not analytic everywhere. See [1] for more details.
order at each of the two given reference points. The expansion can be computed even when the function being approximated is not analytic everywhere. See [1] for more details.
Reference
[1] R. Hablützel. "Polynomial Division Revisited:  -point Taylor Expansion." (Jun 19, 2020) underthemath.wordpress.com/2020/06/12/polynomial-division-revisited.
-point Taylor Expansion." (Jun 19, 2020) underthemath.wordpress.com/2020/06/12/polynomial-division-revisited.
Permanent Citation