Wave Packet Dynamics


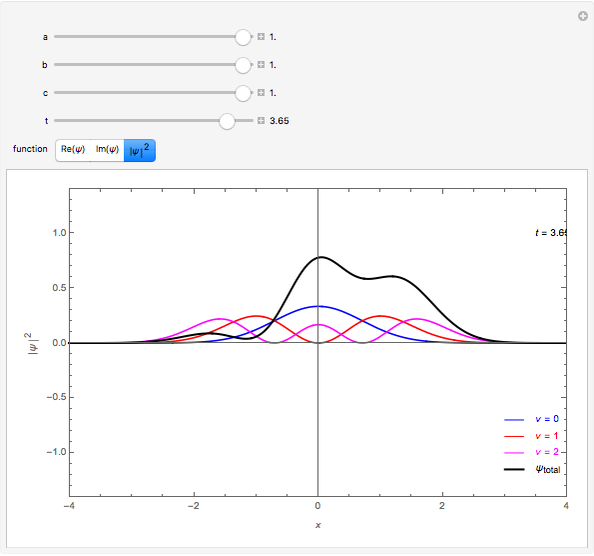
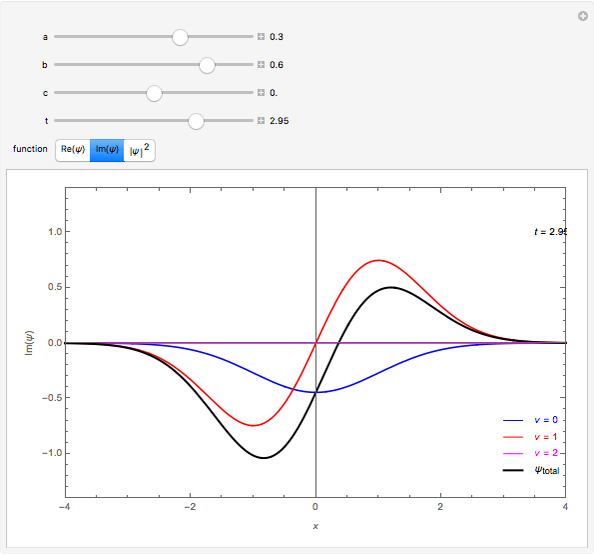
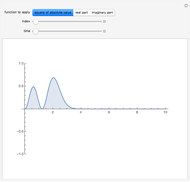
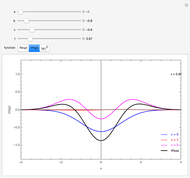
	Wave packet dynamics can be studied by pump-probe femtosecond spectroscopy of vibrations of molecules in excited states (see, e.g. A. Assion et al., Z. Phys. D, 36, 1996 pp. 265–271). As a simple example, consider a superposition of the lowest three eigenstates of the harmonic oscillator. The initial state is set by choosing the relative amplitudes a, b, and c of levels 0, 1, and 2. The Demonstration shows the system's time development in a static harmonic oscillator potential.


	Contributed by: Gerhard Schwaab and Chantal Lorbeer (Ruhr University, Bochum)

Mathematically, a wave packet is an integrable superposition of the wave functions of the stationary problem with time-dependent development coefficients,

, where

is an eigenfunction of the stationary Schrödinger equation. The time-development coefficient in the case of a stationary potential is given by

Based on the harmonic oscillator and a superposition of the lowest three eigenstates, this can be written as

, with

A simplified wave function is given using the appropriate Hermite polynomial

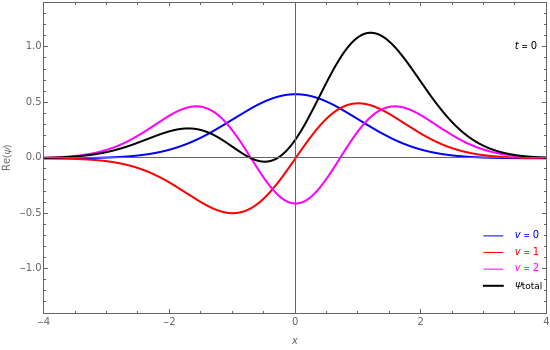
The recurrence times are determined by the lowest level of the harmonic oscillator that changes most slowly. Because the harmonic oscillator levels are equidistant, the example is especially simple, showing a strict periodic behavior. For the Demonstration real relative amplitudes

, and

were chosen for didactic reasons, although in general the relative amplitudes may be complex. In the figure, the real part, the imaginary part, or the probability density of the lowest three harmonic oscillator levels (

= 0, 1, 2) is shown, together with the resulting total wave function.