Weierstrass Approximation Theorem

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
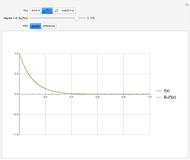
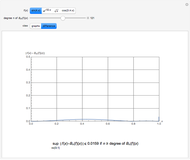
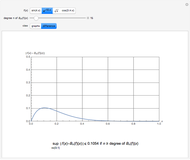
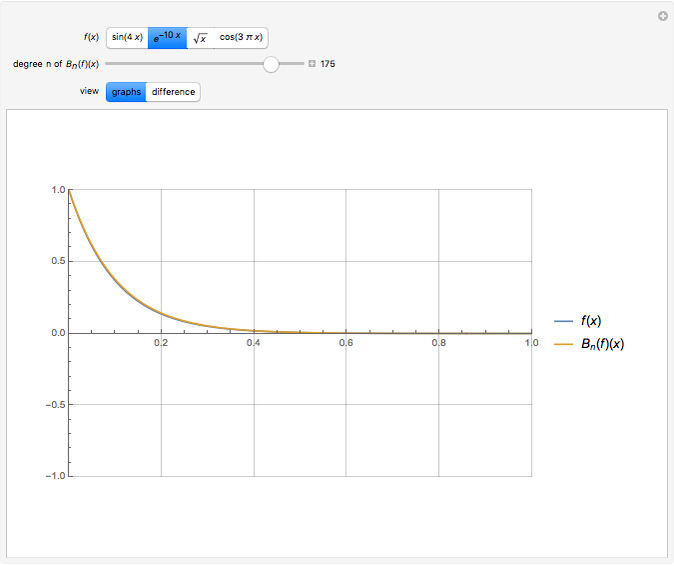
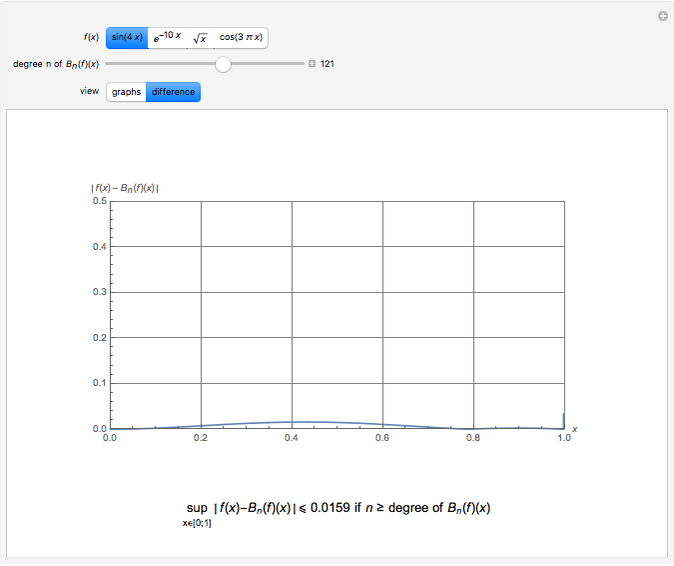
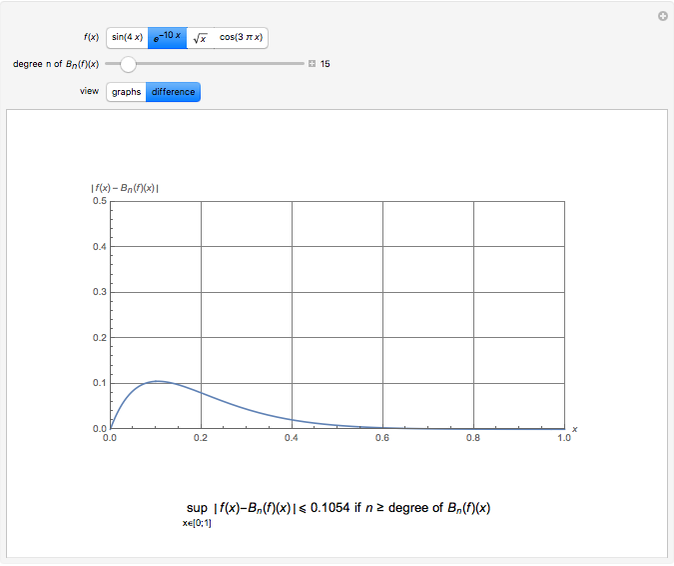
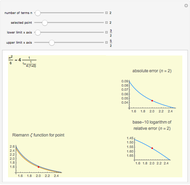
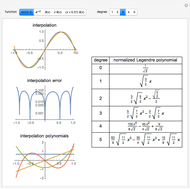
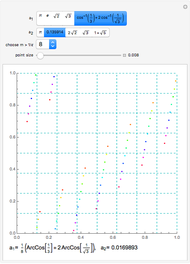
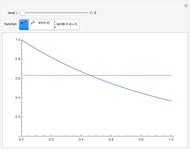
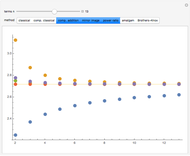
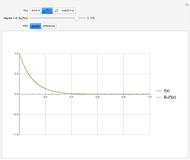
The Weierstrass approximation theorem states that polynomials are dense in the set of continuous functions. More explicitly, given a positive number  and a continuous real-valued function
and a continuous real-valued function 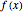 defined on
defined on 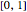 , there is a polynomial
, there is a polynomial 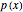 such that
such that 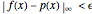 . Here
. Here 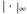 is the infinity (or supremum) norm, which in this case (because the closed unit interval is compact) can be taken to be the maximum. The proof of the theorem is based on Bernstein polynomials constructed from
is the infinity (or supremum) norm, which in this case (because the closed unit interval is compact) can be taken to be the maximum. The proof of the theorem is based on Bernstein polynomials constructed from 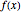 ,
, 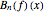 .
.
Contributed by: Fabián Alejandro Romero (January 2015)
Based on a program by: Michael Ford
Open content licensed under CC BY-NC-SA
Snapshots
Details
Permanent Citation