Confidence Intervals for the Binomial Distribution

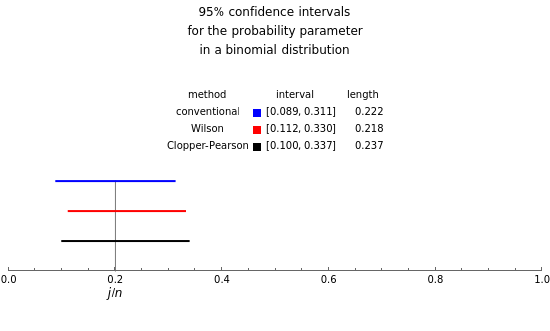
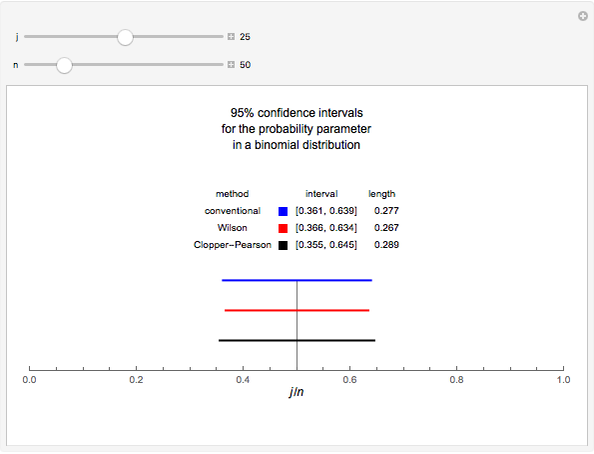
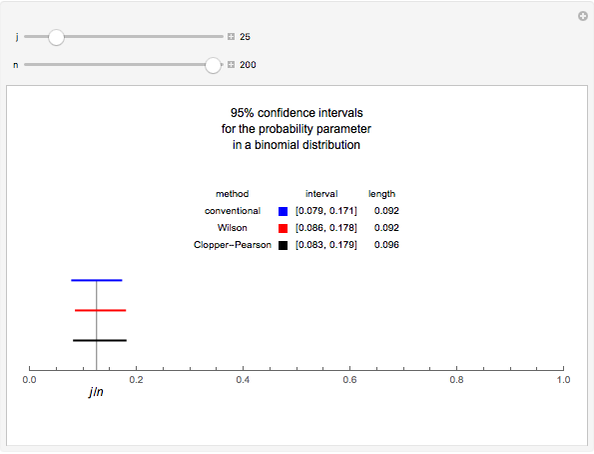
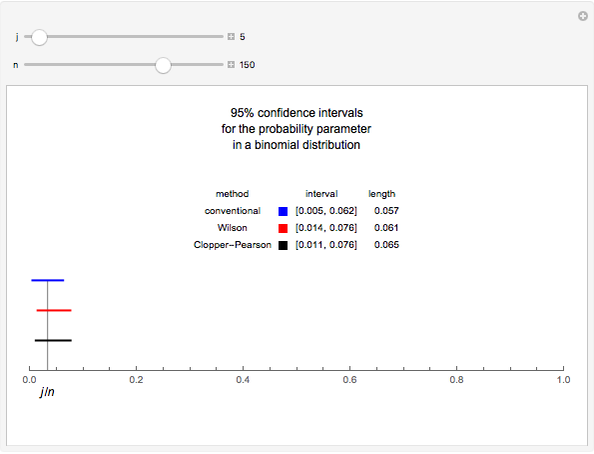
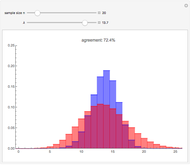
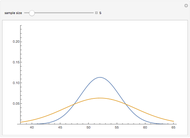
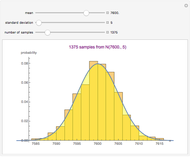
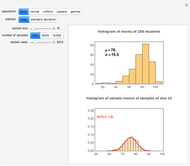
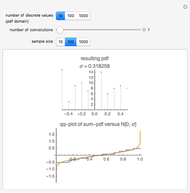
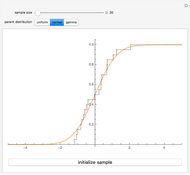
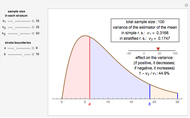
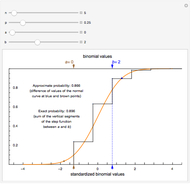
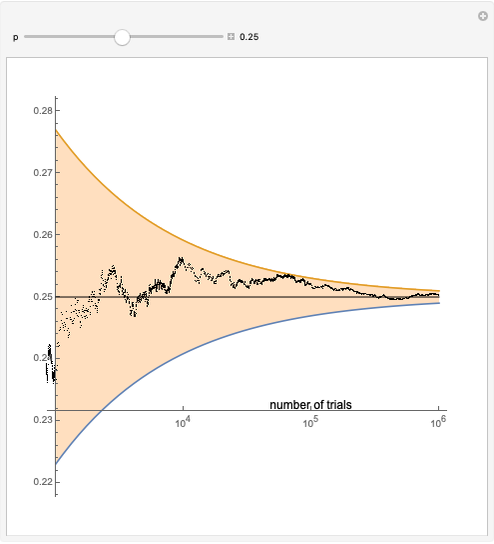
A confidence interval for estimating a parameter of a probability distribution must show two basic properties. First, it must contain the value of the parameter with a prescribed probability, and second, it must be as short as possible in order to be useful. Confidence intervals may be derived in different ways. In the case of a binomial distribution with trials and probability parameter 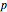, the conventional method for estimating 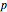uses the normal approximation and produces an interval centered at the point 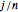, where 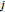is the number of successes obtained in the trials.

Another method, known as Wilson's score method, has some advantages over the conventional one. In general, it has shorter length and, for small values of , it is not centered at this value. A different approach, known as the Clopper–Pearson method, also shows this property, even though, in general, it produces results that differ from Wilson's method.

These three methods are illustrated in this Demonstration, using in each case the coefficients that are commonly used to attain a probability of coverage of at least 95%. Move the sliders to observe the effect of different values of and on the position and length of the resulting intervals.