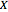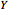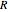Wolfram Demonstrations Project

13,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

Five Famous Fractals

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

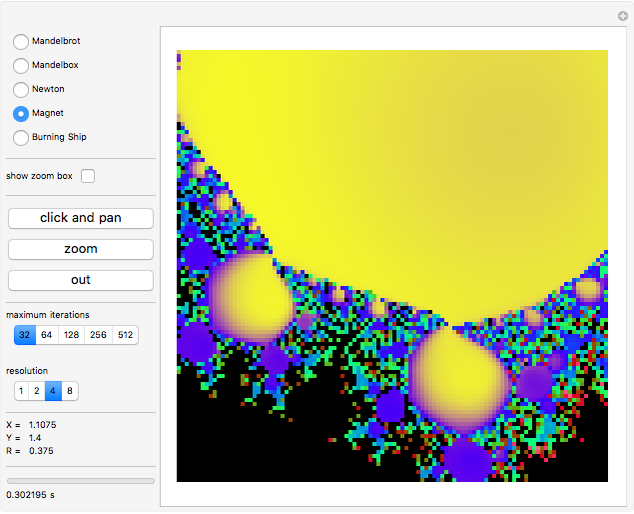
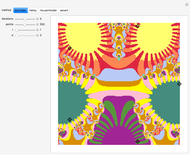
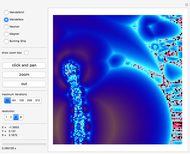
This Demonstration presents a sample from the varied world of fractals. The fractals presented here are images of the complex plane where each pixel is colored differently. Points are colored based on their behavior under iteration of a complex function, with different functions used to generate the different fractals shown here. Sometimes the sequence of iterates diverges by growing without bound in magnitude, and sometimes it converges to a fixed point.

[more]

The values and give the coordinates of the current image's center and is half the width of this image. The status bar at the bottom of the controls shows the progress as a new image is generated and the number of seconds required for the calculation. Use a high number for the resolution when moving about and then choose 1 to get a finer image.

[less]

Contributed by: Roger Glendenning (March 2013)
Open content licensed under CC BY-NC-SA

Snapshots

Details

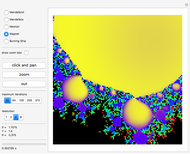
The Mandelbrot set (Wolfram MathWorld) was the first of its type to be visualized using a computer. It has become famous for its beautiful and complex structures. It is produced by the iteration of the simple function .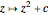

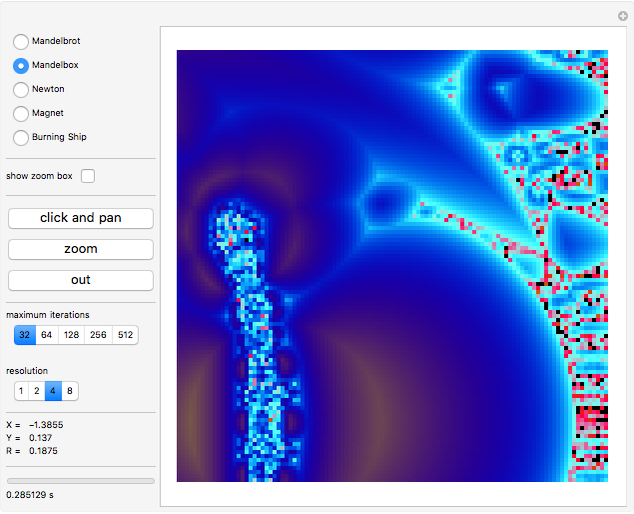
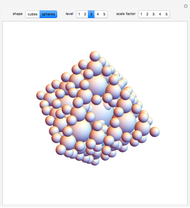
The Mandelbox is a fractal recently discovered by attempting to expand the Mandelbrot set into three dimensions. The iterated function involves geometric folding operations and can be applied to points of any dimensions.

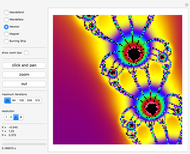
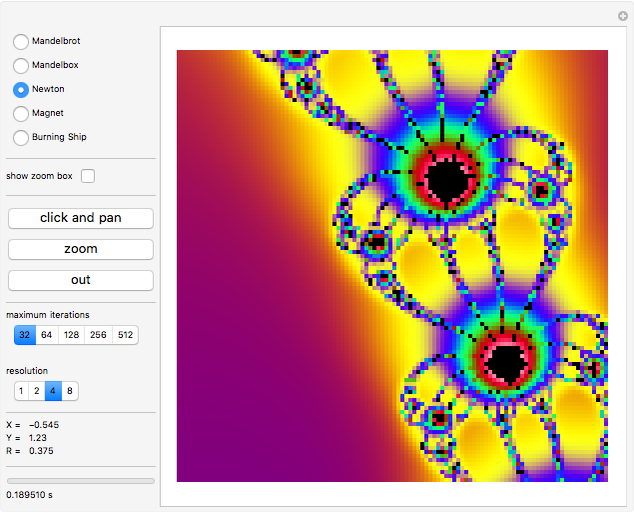
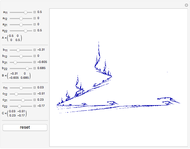
A Newton attraction basin is created by using the iterating function from Newton's numerical method (Wolfram MathWorld) of finding roots 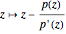. Here the basin is for .

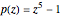The magnet fractal comes from formulas describing magnetic phase transitions, which give a fractal related to the Mandelbrot set; the map is 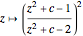. The fractal contains both convergent and divergent points.

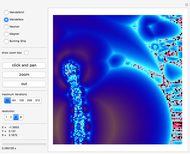
The Burning Ship fractal could perhaps be described as an incorrectly implemented attempt at the Mandelbrot set. Zoom in on the right-hand side around to see the evocative image that gives the fractal its name.

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send