Fourier Series of Simple Functions

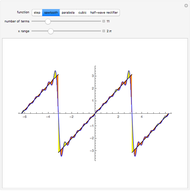
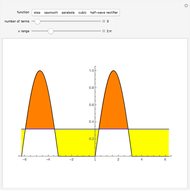
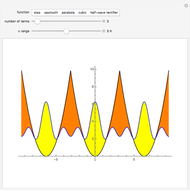
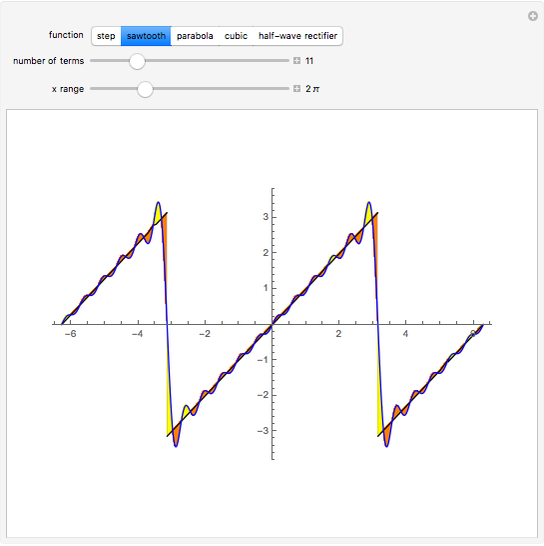
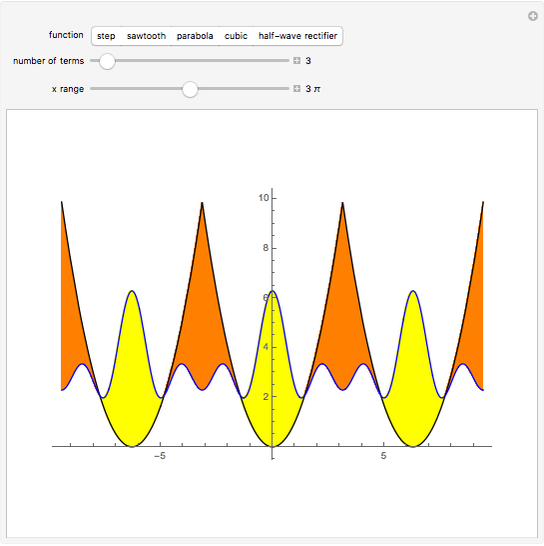
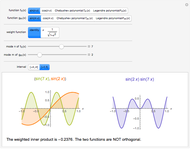
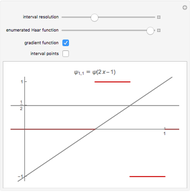
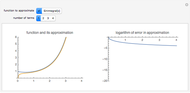
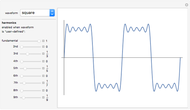
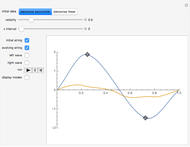
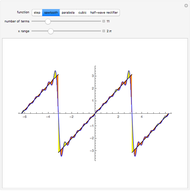
The Fourier series approximations of five different periodic functions are presented together with the corresponding functions themselves. The convergence of the Fourier series (or lack thereof) can be tested by increasing the number of terms in the series. It should be noted that for continuous functions (such as the periodic extension of the parabola), a few terms in the Fourier series provide an excellent approximation. Continuous functions with continuous derivatives (such as the periodic extension of the cubic) can be well approximated with as few as two or three terms.