Likelihood-Based Goodness of Fit in Two-Way Contingency Tables

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
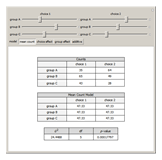
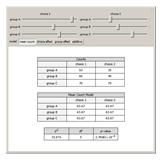
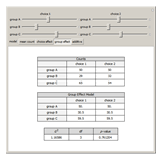
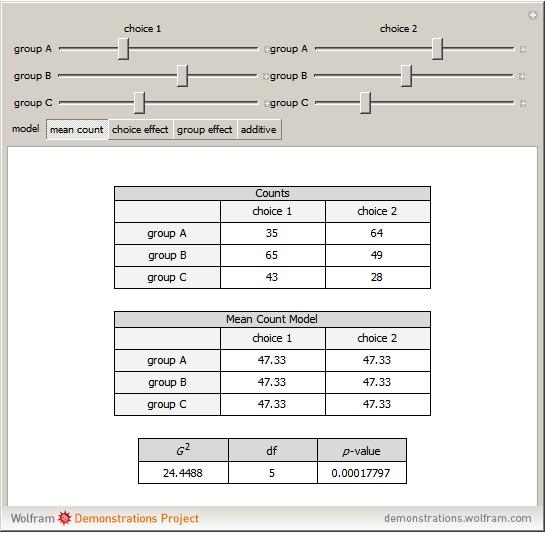
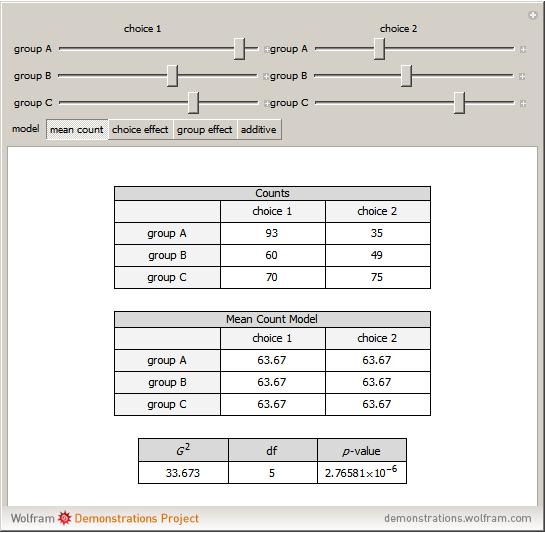
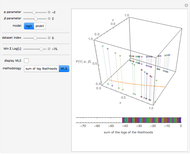
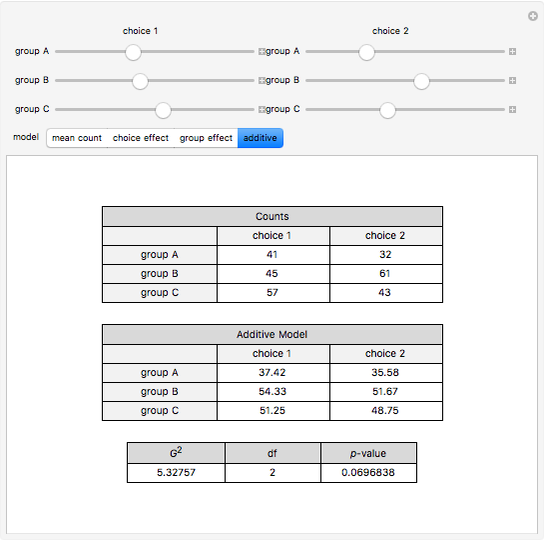
Models of contingency tables are based on the counts by category. In a twoâ€Âway table, models can depend on either, neither, or both of the categories. The 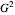 likelihood ratio statistic provides a measure of how well a particular model fits the original counts. The null hypothesis is that the chosen model fits the data well. The alternative hypothesis is that the saturated model (the model with predicted counts equal to the actual counts) is needed. A small
likelihood ratio statistic provides a measure of how well a particular model fits the original counts. The null hypothesis is that the chosen model fits the data well. The alternative hypothesis is that the saturated model (the model with predicted counts equal to the actual counts) is needed. A small 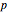 â€Âvalue for the statistic indicates the chosen model does not fit the data well. As the counts in the table get large,
â€Âvalue for the statistic indicates the chosen model does not fit the data well. As the counts in the table get large, 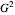 follows a
follows a 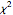 distribution, and a
distribution, and a 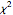 approximation can be used to obtain a
approximation can be used to obtain a 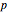 â€Âvalue provided the predicted counts in the table are not very small.
â€Âvalue provided the predicted counts in the table are not very small.
Contributed by: Darren Glosemeyer (March 2011)
Open content licensed under CC BY-NC-SA
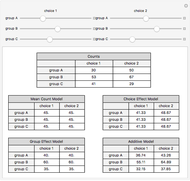
Snapshots
Details
The underlying model is a log-linear Poisson model. The categories are treated as nominal predictor variables.
The mean count model fits the case where each cell is equally likely. This model predicts an equal number for each cell in the table.
The choice effect model fits the case where the count differs across choice, but is constant across group. The group effect model predicts that counts differ across group, but not across choice.
Choice and group have an additive effect on predicted counts for the additive model. The total predicted counts by choice and the total predicted counts by group for this model match those totals for the original contingency table.