Multiplication of Hazard in Expo-Power Distributions

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
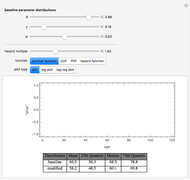
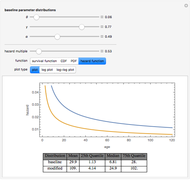
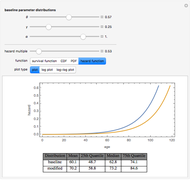
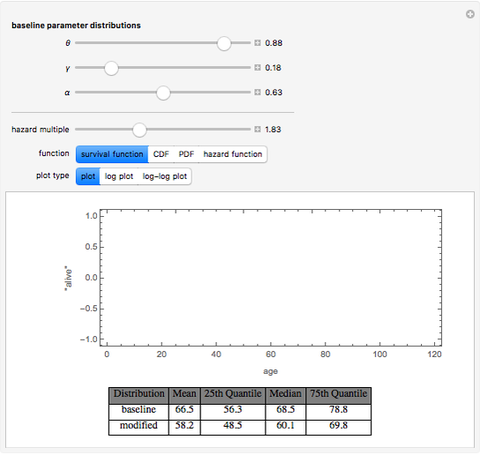
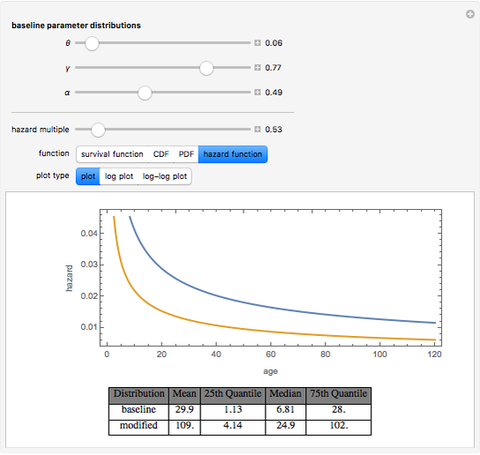
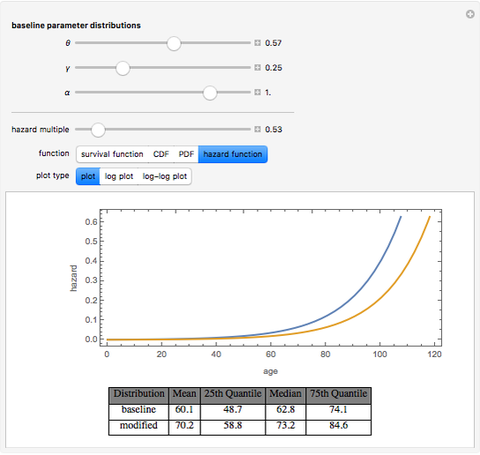
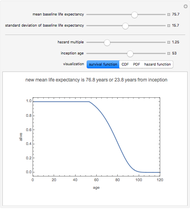
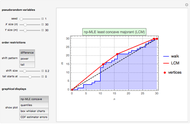
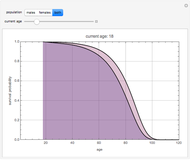
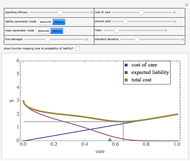
Those involved in examining the duration of phenomena such as physical products or life or legal constructs such as the persistency of an insurance contract often need flexible mathematical models for doing so. One particularly flexible distribution is the so-called expo-power distribution. Unlike less flexible distributions such as the exponential, Weibull, gamma, or Gompertz–Makeham distributions, an expo-power distribution can have hazard functions that are flat, monotonically increasing (e.g. mortality), monotonically decreasing (e.g. insurance contract persistency), U-shaped, or inverted U-shaped. Like the Gompertz–Makeham and Weibull distributions that it nests, the expo-power distribution also has the useful property that a multiple of the hazard function creates a new distribution that is still within the expo-power family. One can thus readily examine questions such as the expected lifetime of an object if one assumes that the hazard confronting that object is a constant percentage of some baseline hazard trajectory. This ability is important because actuaries sometimes use fixed hazard multiples to create mortality tables or lapse tables for subsets of populations for which they have limited data.
[more]
Contributed by: Seth J. Chandler (April 2011)
Insight contributed by: Darren Glosemeyer
Open content licensed under CC BY-NC-SA
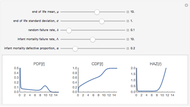
Snapshots
Details
This Demonstration contains several bookmarks that use parameter values that reasonably approximate frequently used baseline distributions in actuarial science such as the 2001 CSO mortality table for composite males and females and the 1971–72 LIMRA lapse rate tables for term and permanent insurance.
The expo-power distribution, like its cousin the Gompertz–Makeham distribution, has the property that a multiple of the hazard function creates a new distribution that is still within the expo-power family. Many distributions lack this property.
The original discussion and naming of the expo-power distribution is found in [1]. Applications of the expo-power distribution to utility theory may be found in numerous articles, including [2].
References
[1] A. Saha and L. Hilton, "Expo-Power: A Flexible Hazard Function for Duration Data Models," Economic Letters, 54, 1997 pp. 227–233.
[2] A. Saha, "Expo-Power Utility: A 'Flexible' Form for Absolute and Relative Risk Aversion," Agricultural Economics, 75(4), 1993 pp. 905–913.
Permanent Citation
"Multiplication of Hazard in Expo-Power Distributions"
http://demonstrations.wolfram.com/MultiplicationOfHazardInExpoPowerDistributions/
Wolfram Demonstrations Project
Published: April 5 2011