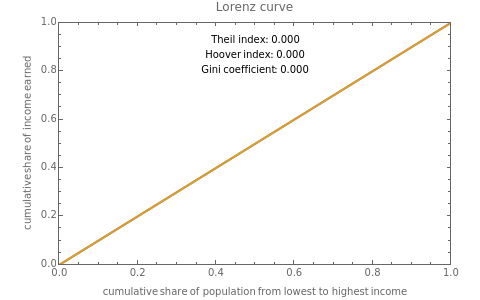
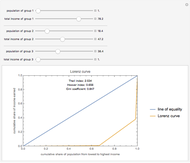
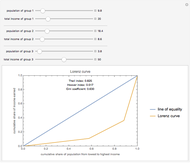
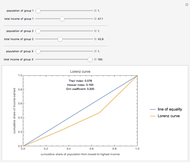
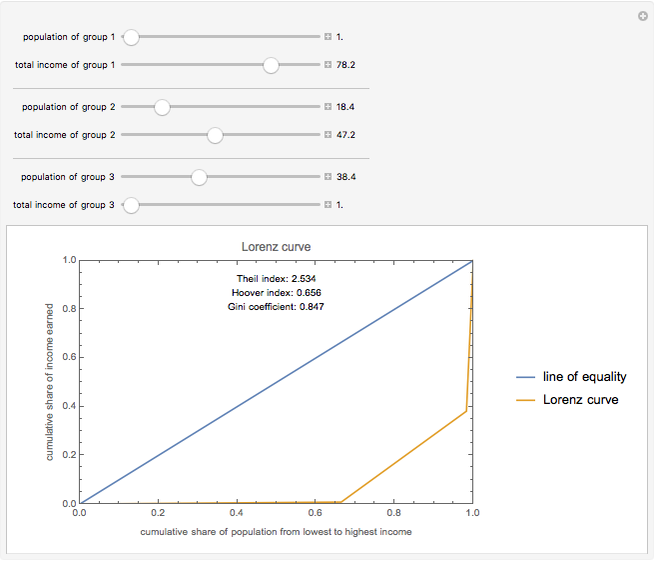
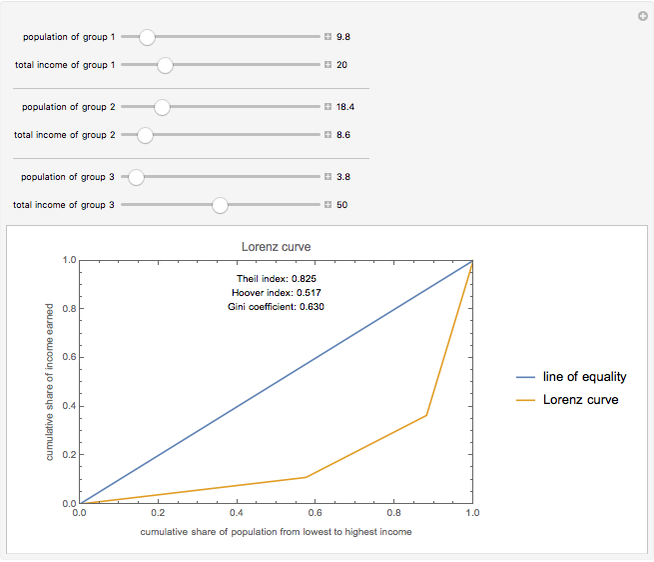
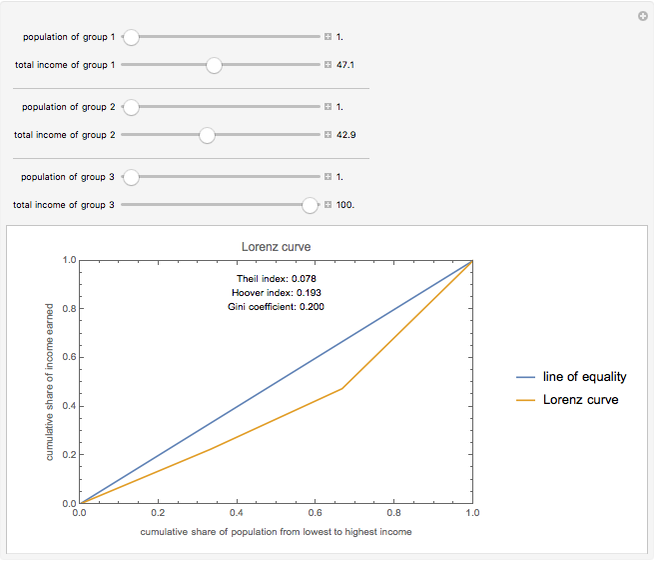
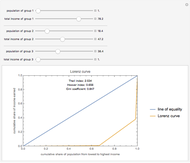
The Lorenz curve is a plot of the cumulative share of the income earned by the cumulative share of the population, with population ordered from lowest to highest incomes. The Gini coefficient is the area between the line of perfect equality and the Lorenz curve divided by the total area under the line of perfect equality, with 0 representing perfect equality and 1 representing perfect inequality. The Hoover index is the portion of income that would need to be redistributed to achieve perfect equality. The Theil index is an entropy index that can be viewed as a measure of redundancy; it is the weighted average of inequality within subgroups and, unlike the Gini coefficient, can be decomposed to view the individual Theil index of subgroups.