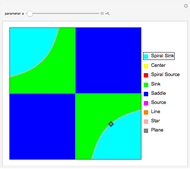
Trace-Determinant Plane

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
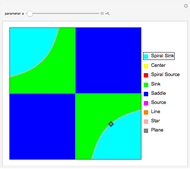
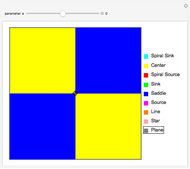
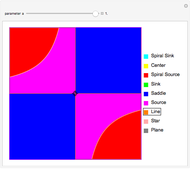
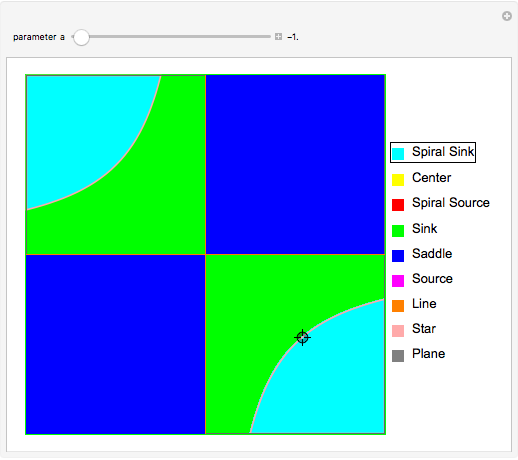
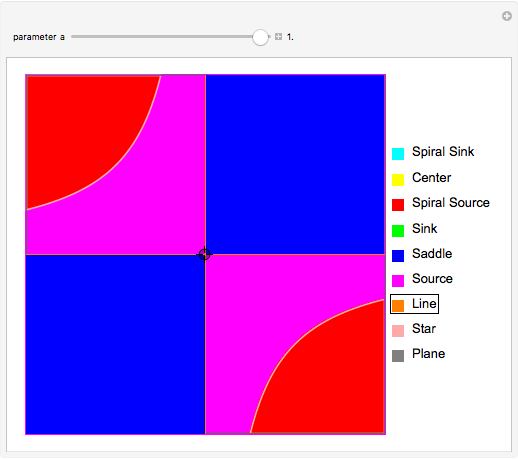
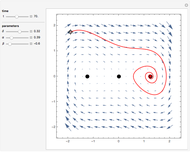
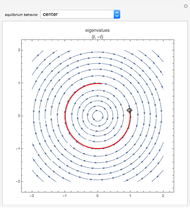
The stability of solutions of a linear system of differential equations with constant coefficients can be classified using the trace and determinant of the coefficient matrix, without having to solve the system. Here the system in question is of the form 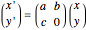 , where
, where  ,
,  , and
, and  are real parameters. The stability of solutions of this system exhibit a bifurcation at
are real parameters. The stability of solutions of this system exhibit a bifurcation at  , and solutions are qualitatively different depending on whether
, and solutions are qualitatively different depending on whether 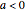 ,
,  , or
, or 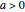 . This Demonstration represents the stability of solutions in the
. This Demonstration represents the stability of solutions in the 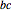 -plane as
-plane as  varies. Drag the locator to identify the different regions.
varies. Drag the locator to identify the different regions.
Contributed by: John Elliott (July 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Chapter 4 of [1] provides a readable account of these ideas and was the inspiration for this Demonstration.
Reference
[1] M. W. Hirsch, S. Smale, R. L. Devaney, Differential Equations, Dynamical Systems and an Introduction to Chaos, San Diego: Elsevier, 2004.
Permanent Citation