2D Root Systems

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
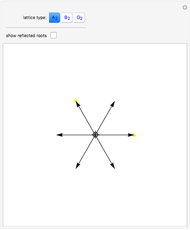
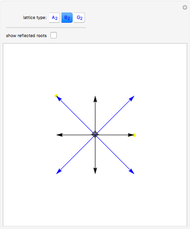
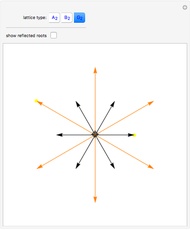
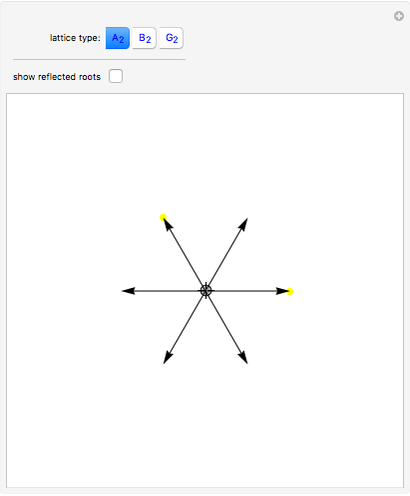
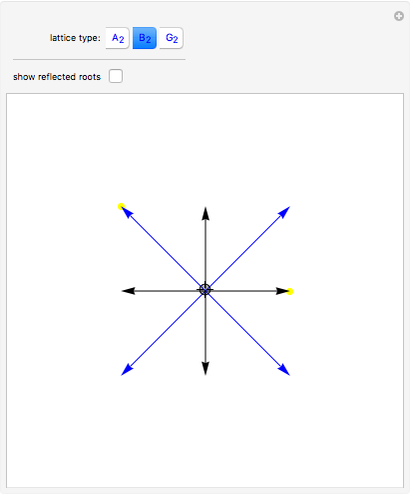
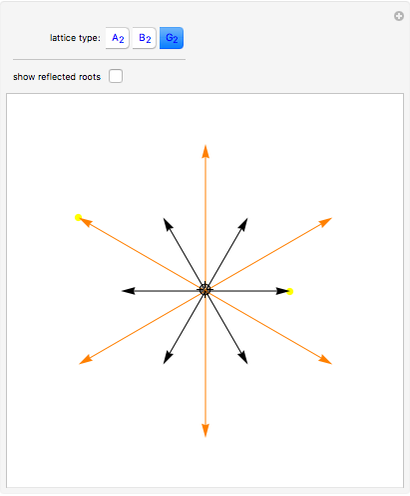
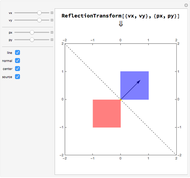
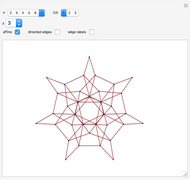
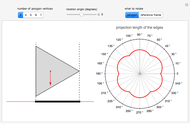
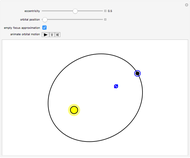
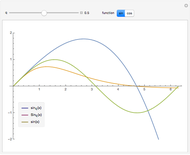
A root system in  is a finite collection of spanning vectors (roots) closed under reflection with respect to planes perpendicular to roots. Any hyperplane in
is a finite collection of spanning vectors (roots) closed under reflection with respect to planes perpendicular to roots. Any hyperplane in  divides roots in two sets—positive and negative roots. There are only three irreducible root systems in
divides roots in two sets—positive and negative roots. There are only three irreducible root systems in  , labeled
, labeled  ,
,  and
and  . Roots of different length are colored differently. Drag the locator to choose the reflection plane. Click on "show reflected roots" and note that the root system is closed under reflections about the line perpendicular to a root.
. Roots of different length are colored differently. Drag the locator to choose the reflection plane. Click on "show reflected roots" and note that the root system is closed under reflections about the line perpendicular to a root.
Contributed by: Oleksandr Pavlyk (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Every root of the root system could be written as an integral linear combination of simple roots. The number of simple roots is called the rank of the root system. Simple roots are marked with yellow dots.
Reflections associated with simple roots generate finite groups. For roots systems relevant for this demonstration these are the Weyl groups  ,
, ,
,  of orders 6, 8, and 12, respectively.
of orders 6, 8, and 12, respectively.
Permanent Citation