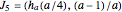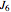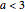Wolfram Demonstrations Project

12,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

A Special Parameter Value for the Logistic Map

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

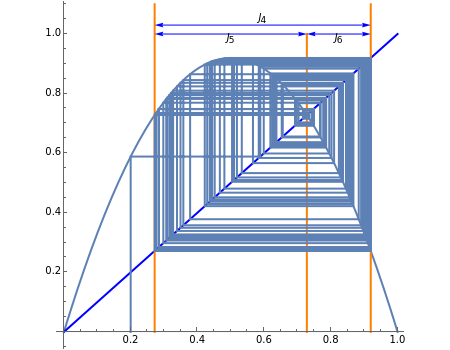
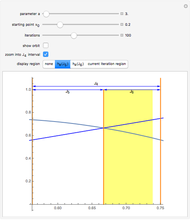
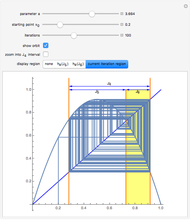
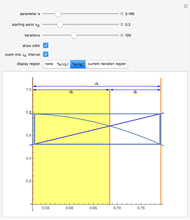
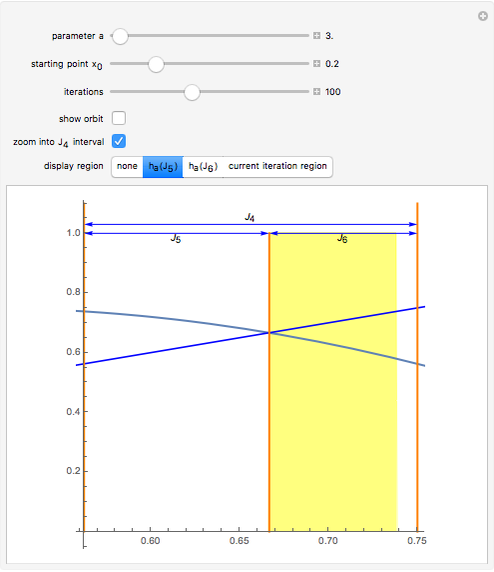
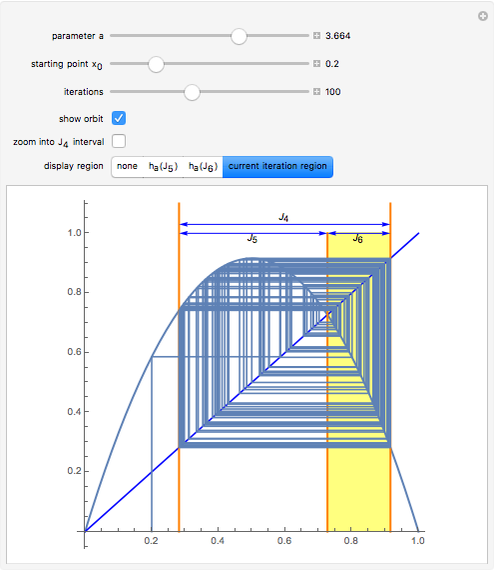
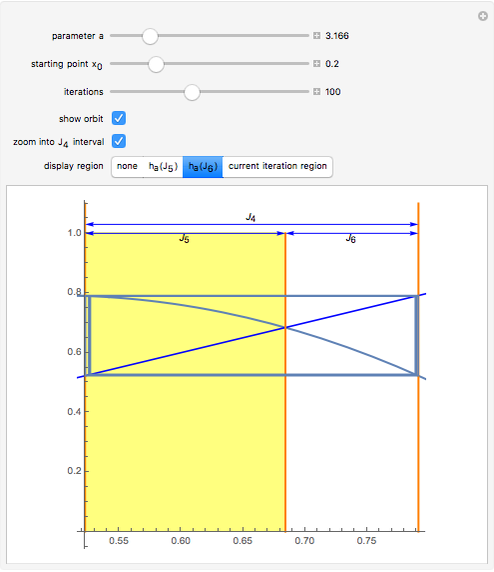
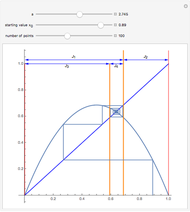
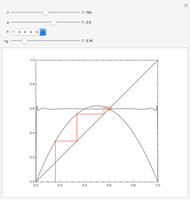
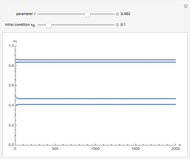
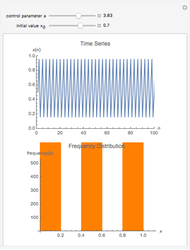
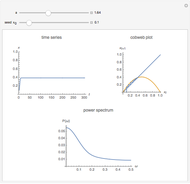
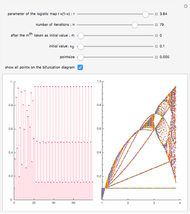
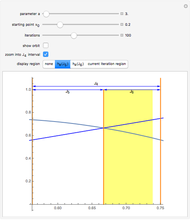
This Demonstration considers the iterations of the logistic map 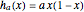for 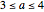. The Demonstration "An Interval Eventually Bounding Trajectories of the Logistic Map" showed how every trajectory with a starting point in (0, 1) is eventually contained in .

[more]

This Demonstration shows that there cannot be any odd periodic orbit if . In fact, in that case the interval is mapped into the interval and is mapped into . It is also easy to show that the points and do not lead to an odd periodic orbit. The previous statements are not true if .

It is possible to limit by , as it is well known that for there is no periodic orbit.

[less]

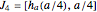Contributed by: Lesinigo Matteo (March 2011)
Open content licensed under CC BY-NC-SA

Snapshots

Details

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send