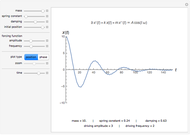
Bifurcations of the Logistic Map

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
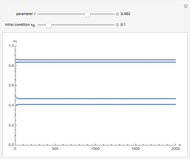
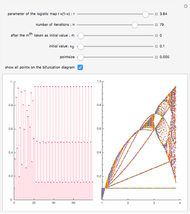
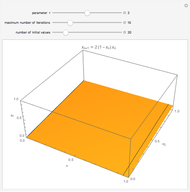
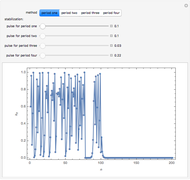
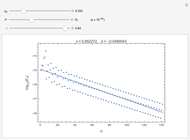
The sequence 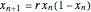 exhibits complicated behavior for certain values of the parameter
exhibits complicated behavior for certain values of the parameter  . When
. When 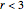 , the sequence converges to a fixed point, but around
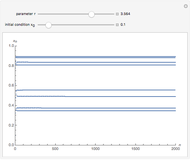
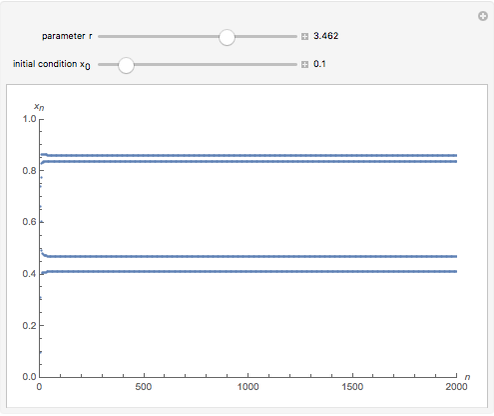
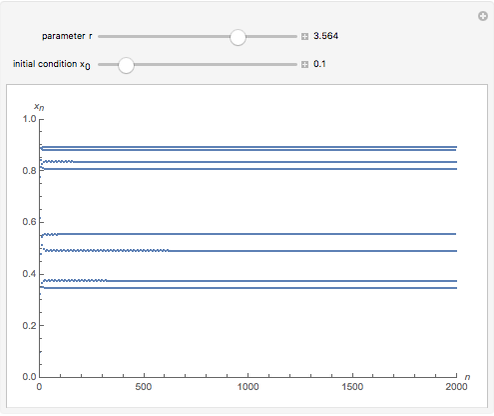
, the sequence converges to a fixed point, but around  this fixed point bifurcates into an attracting two-cycle. As
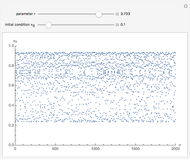
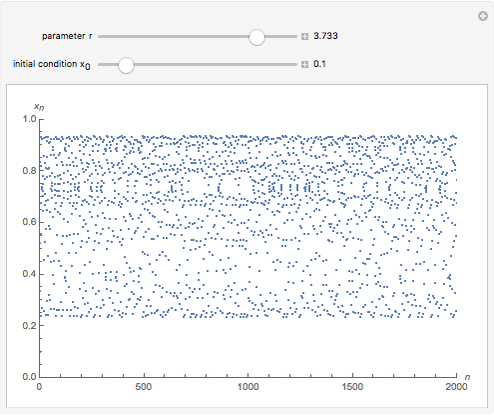
this fixed point bifurcates into an attracting two-cycle. As  increases further, the attractors continue to bifurcate until the sequence displays chaotic behavior around
increases further, the attractors continue to bifurcate until the sequence displays chaotic behavior around 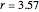 .
.
Contributed by: Rob Morris (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
detailSectionParagraphPermanent Citation
"Bifurcations of the Logistic Map"
http://demonstrations.wolfram.com/BifurcationsOfTheLogisticMap/
Wolfram Demonstrations Project
Published: March 7 2011